The Relationship among Pore-Size Ratings, Bubble Points, and Porosity

Microporous membrane filtration is the technique often applied to the aseptic processing of drug preparations. This is especially appropriate when the ingredients are heat labile. Certain filter performance qualities are of specific interest in such filtrations, namely, the extent of organism removal, the rate of liquid flow, and the total throughput volume. Essentially, it is the numbers and sizes of the pores relative to the number and sizes of suspended particles that determine the filter retention performance, although there are other factors that also affect organism removals (1). The total aggregate space of the pores within the solid filter matrix represents the membrane's porosity. It is constituted of the total number of pores of whatever dimensions. The importance of pore size is obvious. The filter pores are the sites through which the liquid flows. Simultaneously, its suspended particles, such as organisms, are retained at the pores. Ultimately, the throughput is limited by the accumulation of the particles at the pores causing their blockage.

Microporous membranes prepared by the reverse-phase casting process are available from several manufacturers in a series of pore sizes ranging from 0.04 to 8 μm. Curiously, the rating values are not determined by direct pore-size measurements, although several different methods for sizing have been proposed.

Tests such as bubble-point measurements and bacteria challenges are used to assign pore-size ratings. The propriety of translating bubble-point values into pore-size ratings will be discussed later in this article. The assigned numbers are meant to imply particles-size retentions, not dimensional mensurations from which flow properties might also be derived. The bubble points are indicators of the largest size pores present in a membrane; the focus in filtrations being chiefly upon particle retentions.

The significance of pore sizes lies in their implication to particle-size retentions. The quantitative characterization of pore sizes derives from bubble-point measurements coupled with bacterial challenges. These tests are correlative. The former does not compromise subsequent use of the filter. It is a nondestructive test. The latter, although more direct in its diagnosis, is a destructive test in that it contaminates the filter with the test organisms; thus obviating its later application to process filtration.

The organisms used in the microbial challenges differ in accordance with the filter's presumed pore size. The log reduction values necessitated by FDA's definition of a sterilizing filter is the retention of 1 × 10⁷ colony forming units (cfu) per square centimeter of effective filter area (EFA) (2). Such a retention produced against a Brevundimonas diminuta ATCC-19146 confrontation characterizes the 0.2/0.22 μm-rated membranes. Serratia marcescens is the organism commonly used in challenging 0.45 μm-rated membranes. Because Bowman et al. determined that the 0.45 μm-rated membranes retain B. diminuta to approximately the 1 × 10⁵ /cm² level, that test is sometimes used for testing the 0.45-μm membranes (3). The 0.1 μm-rated pore size is mostly tested against Acholiplasma laidlawii. It is fair to say that the testing of 0.1 μm-rated membranes is not yet fully in place within the industry.

Intermittent bubble-point measurement also is used as a process guide during the membrane-casting operation to ensure that the intended pore-size ratings result. The point being made is that the relevant measurements being performed are of bubble points, not of pore sizes. From these, as will be discussed, pore-size ratings and log reduction values (LRVs) are derived.

The pore-size ratings are the remnants of early efforts at membrane classifications that developments in pharmaceutical filtrations have reduced to rather insignificant utility. Pore-size ratings are administrative devices. They serve only as parts numbers or catalogue listings for filter manufacturers. The selection of filters for sterilizing applications is made primarily on the basis of the LRVs that the various filter types impose upon B. diminuta challenges in accord with FDA's definition of a sterilizing filter (2). The relationship of LRVs to experimentally determined bubble-point values enables use of the latter to identify potential sterilizing membranes subject to verification by microbiological assays performed by the filter manufacturer. The putative pore sizes are affixed accordingly. The membranes that meet FDA's stipulation of retaining the 1 ×10⁷ /cm² challenge are listed as being rated as 0.2/0.22 μm.

The membrane pores

Microporous membranes can be prepared by various methods. Among these is the track-etch process wherein a polymeric, dielectric film is bombarded with heavy-mass fission fragments, followed by an alkali etch of the radiation-damaged pathway. This process creates pores that are straight through and regularly cylindrical in shape. Their diameters can be accurately measured with scanning electron microscopy. Despite the relative regularity of their pore structures, these membranes do not usually find application in pharmaceutical processing. The membranes used in pharmaceutical processes are almost exclusively prepared by the phase-inversion technique, generally referred to as the "casting method" (4).

Little is known about the numbers, sizes, and shapes of the pores of microporous membrane so prepared. The membrane structure usually is pictured as being analogous to that of a polymeric sponge. A hypothesized oversimplification of the pore passageways is that of irregular and tortuous capillaries that are, therefore, more extended in length than the filter's surface-to-surface thickness. The pores are marked by irregularly restricted diameters that provide the choke-points that interfere with particle passage. However complex, the pores are pictured as being essentially cylindrical and composed of interconnected spaces extending through the depth of the polymer matrix.

The very concept of a definable "pore" is an artificiality when applied to microporous membranes other than the straight-through columnar pores that characterize the track-etched variety. The complex geometry of the sponge-like membrane results in the pores having ratios of cross-sectional areas to perimeters, called the "hydraulic parameters." These vary over the entire thickness of the membrane (5). A membrane's depth can be constructed of several superimposed unit planes that in their aggregate impose their effect on retention and flow rates (6). The "pores" so considered are presumably connected throughout the unit planes to constitute pathways for fluid flows. However, where particle retentions interfere flow redistributions may result through new "pore" alignments. The "pore" concept arises as a hypothetical construct useful in understanding filter performance. Unlike the track-etched pores, they are not integral, structural pathways for fluid flow.

Pore architecture

The pore structure derives from a cast polymer solution wherein the polymer chain segments are separated from one another by distances that reflect the degree of dilution. It is the inter-segmental distances among the polymeric chains that in their interconnections prefigure the "pores" of the finished membrane. Formulae of various polymer concentrations give rise to different intersegmental separations, ultimately to different porosities, when by proper manipulations the polymer is precipitated as a gel, to be washed and dried to its solid, microporous membrane state. There is inevitably a pore-size distribution and some anisotropic pore shape formation (4).

Figure 1: Free-floating soap bubbles.

It is hypothesized that the formation of the microporous membrane structure accords with the known phenomenon of "soap bubble clustering" (7). The reasons for this resemblance is that in both cases there is the coming together of spheres whose spatial clustering is under the influence of area-minimizing forces. The geometric consequences of these forces is known from the study of soap bubbles (8). Polygonal facets characterize the resulting spaces of a free-floating cluster of soap bubbles (see Figure 1). In the pore formation, the nonsolvent of the casting solution takes the place of the air of the bubbles. In support of such structures, Figure 2 is that of a detergent foam confined between glass plates. The polyhedral spatial structures are obvious. Figure 3 is of a reticulated polyurethane (polymeric) foam. The cellular pores can be seen, in fact, to be polygonal in shape. The phenomenon of clustering through polyhedral spatial arrangements is manifested in other settings as well. It is a trait of zeolitic molecular sieves whose interconnection is through the open panels common to contiguous polyhedra, albeit caused by crystal-packing rather than area-minimizing forces (7). There is, therefore, technical support for the concept of polyhedral microporous structures.

Figure 2: Detergent foam between two glass plates.

In the case of the microporous membranes, the pores, as stated, are formed from the open intersegmental areas prefigured in the casting solution. They are hypothesized to be of various polygonal shapes, framed by polymeric struts and walls, and to be, like the zeolites, interconnected by openings in their common walls. It is these openings that are seen to be the metering and retaining pores of the membranes. As stated, casting solutions of various polymer concentrations give rise to separate but rather similar intersegmental distances. It is these spaces that are ultimately transformed into the membrane pores that, in consequence, are also similar in their dimensions—except for the pore-size distribution that results from the casting solutions' deviation from an ideal homogeneity.

Figure 3: Reticulated polyurethane foam.

Filtration is not a simple sieving process, except perhaps in the case where the filter is a track-etched membrane with pores passing straight through a much thinner film (~10 μm). As stated however, for the membranes prepared by the casting process (thinness ~150 μm), a fluid on passing through encounters pores of different diameters and surface areas. Particles are separated from the fluid by adsorptive attachments to the pore surfaces as well as by the size exclusion mechanism. Thus, the meaning of the average pore size as reflecting the size of a restrictive diameter within a pore passageway is necessarily an oversimplification. Nevertheless, the events taking place at the pores are depicted as if the pores were continuous and integral paths through the depth of the membrane.

Pore-size distributions

Membrane characteristics are assessed because of their pertinence to aseptic processing. However, the pore-size distribution, despite being an important structural feature that influences both flow and retention, is not among them. It is seldom known or investigated although an ASTM method based on airflow rates enables its assay (9). The reason for not assaying the pore-size distributions of filters is that complete organism removal is dependent upon the largest pores of the pore-size distribution retaining the smallest particle of the particle-size distribution. This is the singular circumstance wherein an absolute filtration can eventuate. Given this felicitous situation, only the size of the largest pore has pertinence. In fact, however, the distributions of neither the pore nor organism sizes are likely to be known by the filtration practitioner. Depending on the relative numbers of pores and particles and their sizes, particles may encounter the larger pores of the distribution to escape removal. These larger pores of a distribution are assayed by bubble-point measurement recorded in pressure units (psi or bar) rather than in dimensional units (μm). The accompanying smaller size pores are ordinarily not quantified or measured because they are not seen to influence retentions.

Methods of pore-size rating

By porosimetry. Numerous methods have been used to assess pore size (10). Early on, mercury porosimetry was the method of choice (11–13). In this procedure, mercury is forced under pressure to penetrate into membrane pores. This process is performed at increasing differential pressures, ΔP. The higher the value of ΔP, the smaller the pores that the fluid metal can intrude. Quantitation of the different pore sizes relates to the volume of mercury that is intruded in filling the pores at each of the progressively increasing differential-pressure stages. Airflow porosimetry studies also have been performed (14, 15).

The porosimetry method is flawed by the assumptions necessary to its application. It is not suited to polymeric materials that are at temperatures above their glass-transition points and whose pores are, therefore, liable to stretching and distortion under the pressures of the intruding fluid. Also, the averaging of volume changes required by the technique may mask the true dimensions of the "pores."

By particle retention.Pore sizing based on the retention of organisms of presumably known sizes has been widely investigated (14, 16–18). Pore sizing attempts also were made using latex beads of very narrow particle-size distributions (19, 20) (see Table I). These latter methods, in conjunction with the use of surfactant, have the advantage of eliminating adsorptive effects from those ascribed to sieving (21) (see Table II). Various surfactants were found to differ in their influencing the sizing results (22). The assigned pore sizes assumed the particle to be spherical and the area of pore restriction to be circular in shape. Simplifying assumptions were necessitated to finesse the shape factor that is operative in retentions. The exclusivity of sieve retentions also was assumed, ignoring adsorptive arrests, and at best allowed inadequately for pore-size distributions. Reductions in organism sizes resulting from contact with given liquid preparations, shown to be possible by Sundaram et al. (23) were not investigated. Nor have the possible plasticizing effects of the suspending liquids on membrane pore-size alterations reported by Lukaszewicz and Meltzer in 1980 been investigated (24).

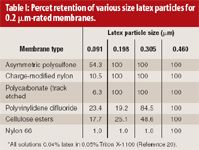
Table I: Percent retention of various size latex particles for 0.2 μm-rated membranes.

The results of these trials usually were judged to be of limited value. This is typical of efforts where simplifying assumptions are used to support a hypothesis, and the very conclusions are limited by the inherent arbitrariness of the necessary premises. They are complicated by the fact that the definitions of the particles themselves may depend on the particle-measuring methodologies, on the procedural protocols, and on the measuring devices used (16).

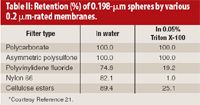
Table II: Retention (%) of 0.198-μm spheres by various 0.2 μm-rated membranes.

By flow through pores. The engineering principle enabling an orifice's dimensions to be sized using the rates of flow it permits can be applied to determining pore sizes. The rate of flow through a pore at a given differential pressure is essentially inversely proportional to its length and directly so to its width or diameter. Thus, the flow characteristics of single pores are described by the Hagen–Poiseuille Law:

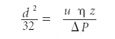
wherein a fluid of viscosity η with an average velocity u of the fluid is related to the tube diameter d and pressure drop, ΔP along a given length z (5).

At the site of the pore's most restricted diameter its retention and flow properties are defined. Nonetheless, flow rate is influenced by more than the pore's area of constriction. It reflects also the length of the pore. Conceivably, a relatively open pore, less constricted and, therefore, less retentive, may be rendered slower-flowing by its extended length. The area of a filter, however, contains numerous pores. Its rate of flow is defined as its flux. It is the total and simultaneous flow through the many pores of the given filter area that is measured. Flux has the dimensions of volume of flow per unit time, under unit pressure differentials, per unit area of filter. Because several pores are involved, the concept of porosity comes into play.

Porosity

By porosity one means the percentage of a filter that is its voids or pores; that is, the ratio of space to solid in the membrane matrix. A given porosity can be constituted of a random combination of various numbers of pores, nonuniform in their lengths and diameters and of various shapes. The more numerous the pores, the more likely a higher flux. However, the lengthier the pores, the lower their individual flow rates. Pores of less-restricted diameters would flow faster but would tend to be less retentive. The filter's sieve retention would be decided by the size of its widest restrictive diameters. It is this dimension that will decide the largest particle to escape capture by penetrating the pore.

As a foreseeable consequence of random pore arrangements, the same flux rates could characterize two membranes differing from one another in their mix of longer and shorter pores and of wider or narrower restrictive diameters, as modified by more or fewer pores. Likewise, the random balancing of numbers, lengths, and widths of pores could produce filters of the same porosity but differing in their flux and retention. As said, the pore-size ratings are indicative of particle retentions. Flow rates are not a function of retention ratings. Individual filters of different pore-size ratings could be characterized by similar rates of flow or even by flow rates opposite from those expected on the basis of the pore-size (retention) rating. Membranes, if any, based on a randomness in pore formation could be of a same pore-size rating, indicative of retentions, but of higher porosities and flow rates. This would depend upon the random mix of pore numbers, lengths, and widths. Longer pores, the accompaniment of thicker membranes produced by filter layering, exhibit reduced flows but tend to greater retentions. Pall and Kirnbauer showed that layering in increasing the membrane thickness, elevated the bubble point to some leveling value (25).

Figure 4: Modelling a fibrous filter by a system of lines drawn at random (n = 25).

Certain filters, especially depth filters composed of fibrous structures, are manufactured by a technology that produces filters of randomly arranged pore structures; hence, their broad pore-size distribution (see Figure 4). Membranes fabricated using the casting technique, however, are characterized by the narrow pore-size distribution that is the result of the physical laws governing solutions. Within the volume of any solution, an equilibration by diffusion, and hastened by stirring, results in the solute molecules becoming evenly spaced from one another. The casting formula is such a solution of polymer dissolved in solvent. Within this solution the polymeric segments are, therefore, essentially equally spaced from one another. It is this relative regularity of intersegmental spacing that prefigures the similarly sized pores of the finished membranes. The resulting pore structure is, thus, not the product of random mixes of various pore lengths and diameters but rather the consequence of influences directed to pore homogeneity. It bespeaks a greater regularity of the pore structures constituting the porosity. The pores of cast microporous membranes should, therefore, be of a rather uniform size. This is, indeed, the case as shown by their narrow pore-size distributions (see Figure 5).

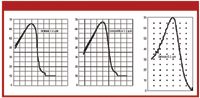
Figure 5: Automated flow pore measurements of two different 1.2 μm membranes.

The possibilities are unlikely of pores of such regularity in size exhibiting identical retention ratings but differing in flow rates. Except for the irregularities introduced by pore-size distributions, organism retention, flux, and porosity should be parallel expressions of the membrane's pore size. This assumes, however, the use of identical polymers, casting solutions, and converting techniques, along with standard systems of measurements. None of these conditions are likely met by competitive filter manufacturers using proprietary practices. Therefore, comparisons, as of flux or of retentions, between filters of various manufacture may not usefully be extended to speculations concerning their structural features or performances.

Ambiguities in pore-size rating

Within any given pore-size designation available in the market, there exists some range to its quantified properties. In its manufacture, a membrane lot may, and indeed will, incur some variation in the in-process bubble-point measurements that are translated into pore size. Each pore-size rating is prepared using a different casting formula. Nonetheless, the preparations do not yield distinct quanta, although the variations within a class are less than those between classes. Each batch or even each filter within a batch of membranes is classified to a single pore-size value, although each of the individual filters comprising the group may have its own bubble point within the range of values that define the given rating.

As Schroeder points out, the pore size–retention correlation is not a step function (26). In its manufacture, a membrane lot will incur some variation. In using nonuniform standards, filter manufacturers might assign their pore-size labels somewhat ambiguously. One fabricator may, on the basis of flux, consider a membrane to be an "open" 0.1 μm. Another filter producer, using a somewhat different rating system might classify it as being a "tight" 0.2 μm. This could give rise to a labeled 0.1 μm, not so handicapped by reduced flows, being compared with a labeled 0.2 μm, not so advantaged by enhanced flows. The more "open" 0.1 μm may not flow faster than an average 0.2 μm but may do so against a "tight" 0.2 μm. Consequently, it becomes an unrewarding exercise to try to compare competitive membranes each rated by their own individual catalogue descriptions as perhaps being of the same pore size, yet exhibiting different flux rates or retentions under test.

As is usually the case in the physical sciences, reliable evaluations and meaningful comparisons must be derived by users from performance data obtained through experimental investigation founded on suitable experimental designs. Another possibility for initial comparisons is the standard ASTM organism challenge test designed for 0.2/0.22 μm-rated filters but extendible to 0.1- or 0.45 μm-rated membranes as well (27).

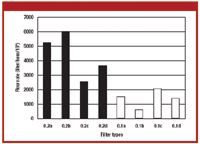
Figure 6: Different flow rates of 0.2- and 0.1-μm rated filters.

Consequences of rating ambiguities

As shown in Table II, Tolliver and Schroeder found that the retention of 0.198 µm latex particles by five commercially available 0.2/0.22 μm-rated membranes differed significantly (21). The particle-retention mechanism was not complicated by adsorptive sequestrations because the solution contained surfactant. Given the size uniformity of latex particles, the variation in retentions based on sieving indicates different reference standards for the like-rated pore sizes. Nonstandard ratings also explain the Lindenblatt et al. (28) report that the flow rates exhibited by four 0.2/0.22 μm-rated membranes, as also of four 0.1 μm-rated filters differed markedly (see Figure 6) (28). Moreover, as indicated in Figure 7, the throughput volumes measured for the nonstandardized similarly rated pores also differed. Comparable data are offered by Jornitz et al. (29).

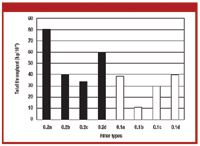
Figure 7: Different throughputs of 0.2- and 0.1-μm rated filters.

Pore-size ratings and validation

Until rather recently it was believed that the sterilization of fluids could be achieved by their filtration through a "sterilizing" membrane whose proper and pertinent identity was confirmed by its pore-size rating, which was itself determined by bubble-point measurement. This belief arose from the membrane's successfully withstanding a microbiological challenge of 1 × 10⁷ cfu of B. diminuta per square centimeter of filter surface. On this basis, the membrane, in accord with FDAs definition, was considered a "sterilizing filter."

Contrary to common belief, B. diminuta was not selected to be the model organism because it was thought to be the smallest microbe—smaller organisms were known for decades (30). Rather, it was chosen as likely to be the smallest organism that might commonly be encountered in nonsterile pharmaceutical preparations. Therefore, its filtrative removal was considered as indicating with a high probability that the membrane used was a sterilizing filter. Using this type filter, identified by its bubble point, was assumed, with this caveat, to ensure a sterile effluent.

Over time, it became evident that positive conclusions based on pore-size ratings were subject to modification by the physicochemical specificity of the organism-suspending fluid, by the individuality of the organism type in its size-changing response to the fluid, in the possible change in pore size induced by the fluid, and by the adsorptive qualities of the filter resulting from its particular polymeric composition. All of these factors are influenced by the filtration conditions in their numerous varieties, but especially by the transmembrane pressure.

A filter may not sterilize the same preparation under different filtration conditions, especially under dissimilar differential pressures (31). A given membrane may or may not retain a particular organism type suspended in a different drug vehicle (3, 31). The organism type need not remain constant in size but may alter in response to its suspending fluid (32–34). The effect of the vehicle upon the polymeric membrane may cause a change in its pore sizes (24). What had once seemed simple is now recognized as being quite complex. Pore-size ratings alone are not sufficient to ensure the validation of a sterilizing filtration.

Given the above, it is surprising that The European Medicines Agency (EMEA) has stipulated certain requirements for a bioburden confronting the sterilizing filter. Its 1996 Notes for Guidance states, "In most situations NFT 10 cfu/100 mL will be acceptable depending on the volume to be filtered in relation to the diameter of the filter. If this requirement is not met, it is necessary to use a prefiltration through a bacteria-retaining filter to obtain a sufficiently low bioburden" (35). In recommending the use of a bioburden-reducing filter when the level of 10 cfu/100 mL is exceeded, the EMEA guideline states, "The type of bacteria-retentive filter and its pore size should also be described in the application. Pore sizes of 0.22 μm are acceptable without further justification" (emphasis added). It would seem that validation by pore size is still acceptable to the EMEA.

Organism quantitation

Conclusions cannot be made regarding the sterile filtration of microorganisms unless methods of quantifying them by culturing and counting are available. Organisms such as the L-forms, nanobacteria, and "viable but nonculturable" entities may not be amenable to such analyses. Concerns about their presence may be justified but without the means to cultivate and count them, it is impossible to attest to their complete absence. It follows that a sterilizing filter can be judged only by its performance in the removal of identifiable and culturable organisms known to be present in the drug preparation (36).

Bubble points and "the largest pores"

An alternative approach focuses on measuring the set of "largest pores" of a filter. These are the least resistant to fluid flow. They, if any, would be the most likely to permit organism passage. The measurement is of the pores' constricted diameters rather than of their lengths. It is at these choke points that the size of a particle just large enough to be retained is defined. The pore diameter varies along the length of the pore passageway and differs among the various pores of the filter; hence, pore-size distributions characterize the microporous membranes.

It is the size of the smallest diameter of such a largest pore that is measured by the bubble-point test. As more accurately stated by the Aerospace Recommended Practice, "No bubble point test measures actual pore size but only allows correlation of the measured capillary pressure with some dimensional characteristics of the pore structures" (37). Although not an absolute measure of specific pore sizes, the pressure levels designating bubble points bear the pore sizes a strong relationship on the basis of the capillary rise experience and provide an indication of their magnitude (38).

The other means of measuring filter integrity are the equal of the bubble point for that purpose. They all are accepted as being of equal reliability when properly performed. The forward flow method—often if somewhat erroneously, identified as a single-point diffusive airflow procedure—is an example. The "diffused air" that is measured, however, is the product of Fick's Law of Diffusion that reflects porosity, the total volume of all the pores regardless of their sizes, and not the diameters of its largest pores:

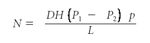
in which N is the permeation rate, D is the diffusivity of gas in the liquid, H is the solubility coefficient of gas in the liquid, L is the membrane thickness, (P₁–P₂) is the differential pressure, and p is the total porosity.

By contrast, the air quantified in the bubble point reflects, albeit inexactly, that which passes only through the set of largest pores in accord with the capillary rise equation. It is the implications to pore size and, therefore, to particle retentions that suit the use of the bubble-point test for this purpose.

The bubble-point equation, based on the capillary rise equation, involves a reciprocal relationship between its value and the size of the "largest pore" diameter. It is the pore diameter that is being sought.

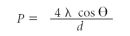
in which P is the bubble-point pressure, d is the pore diameter, λ is the surface tension, and θ is the wetting angle.

As the applied differential pressure rises, the water layers in the largest pores thin. Successively the next smaller pores are likewise affected and so forth. Eventually the water is expelled from them. The affected pores progressively increase in number from a very few. In the process the air that underwent diffusion becomes added to by the bulk airflow occurring through the vacated pores that also increase progressively in amount. The collected air is then, strictly speaking, not composed completely of diffused air. To this extent, the term "diffusive airflow" is a misnomer (39).

The capillary rise situation

Bubble-point measurements are based on the capillary rise phenomenon. When glass capillaries are dipped into water the liquid rises within them. The rise is motivated by hydrogen bonding of water molecules to the capillary's hydrophilic silicate walls. Given capillaries of different radii, the water rises highest in the narrowest capillaries because the ratio of wall area to liquid volume is greatest and the attractive force of the hydrogen bond more directly affects a larger proportion of the water molecules. In the capillaries with wider lumens there is, in effect, a free-standing column of water not in direct contact with the glass walls. Fewer of the water molecules directly experience the intermolecular forces attracting them to the silicate moieties. As a result, the liquid rises to a lesser extent in the wider capillaries and can be expelled by lesser air pressures. The water within the capillaries of the widest diameters is expelled first.

In performing the bubble-point measurement, a water-filled membrane is suitably positioned and retained in a holder so that a progressively increasing air pressure can be directed against its upstream face. It is assumed that membrane pores act like simple, round capillaries in their imbibition of water and in their being emptied under a differential pressure. The water filling the pores prevents frank air passage until the applied air pressure becomes large enough to expel the blocking water from the widest pores. The passage of air then escaping through the vacated passageways is evidenced by the appearance of bubbles in a downstream pool; hence the term "bubble point."

The vacating of the liquid by an imposed air pressure represents a work function, namely, a forced separation, (removal) of the wetting liquid from the polymer surface (40). The bubble-point pressures reflect the various strengths of the bonding interactions between different polymers wet by different liquids. The air pressure needed to separate the water molecules from the pore walls, as occurs upon emptying the pores, quantifies the work function involved. A given polymer type with different liquids, or a given liquid paired with various polymeric type membranes, results in bonds of different strengths and is different as well for each pore size.

Thus, different bubble points (delta pressures) quantify the force expelling the wetting liquid from the pores. Pore-size ratings, were they but assessable, could be applied to all filter types. The rating of whatever pore size, were it to represent the dimensions of a passageway, would not change with the filter type. As stated, however, the bubble-point value, presumably for the same size pore, is different for each polymer–liquid couple—whether for each polymeric membrane with different liquids or for a given liquid with different type filters. Rating filters by bubble-point values peculiar to each solid–liquid couple of each membrane type and to each pore size would be too cumbersome to be practical.

In practice the membrane is measured by bubble point, which is then rather arbitrarily translated into a pore size rating. The translation rests on a conjunction of two items: FDA defined a sterilizing filter as being one that withstands the challenge of 1 × 10⁷ cfu of B. diminuta per square centimeter of EFA. In addition, several experimenters found that an inverse straight-line relationship existed between the log reduction values and the bubble-point levels for the various polymeric-type membrane filters. The higher the bubble point, the smaller the restricted pore diameter and the greater the LRV value (see Figure 8) (41). One could, therefore, read from a graph the bubble-point value of a given filter–liquid couple that would correspond to the LRV of a "sterilizing" membrane.

The corresponding bubble-point value (using the same liquid) would, however, be different for each of the various polymeric-type membranes. But the value, however different for each type membrane, would identify a "sterilizing filter" as defined by FDA's approved 1 × 10⁷/cm² challenge. It could differ as well for organisms for which B. diminuta may not be a model.

Figure 8: Microbe retention to bubble point.

Integrity test values used in membrane making

In casting the polymer solution in the form of a wet-film preparatory to its conversion to a finished, dry microporous membrane, the manufacturer seeks to predict by periodic testing in the wet casting-state the properties that will eventuate in the dried, finished product. Essentially, what is sought in the wet stage is the foretelling of the proper integrity test value of the finished dry filter. The considerable complexities of the solvent evaporation, washing, and drying stages of the fabrication process are involved in negotiating the change from casting solution to dry membrane. The translation of the characteristics of the wet cast film into those of the dry membrane requires a considerable experience in producing membranes.

The wet testing involved is usually proprietary. Presumably, the test values of any of the integrity tests, including the forward flow, based on diffusive airflows, may be serving this purpose. Experimentally determined bubble points are the guiding measuring units known to the authors to be used. Although the membrane is classified in terms of pore-size ratings, the category is determined on the basis of bubble-point values. The higher the bubble-point value, the smaller the pore-size rating and the more likely the retention of smaller organisms. The grouping by bubble point inexorably gives rise to a spectrum of values for each pore-size rating.

Care is taken that membranes intended for sterilization filtrations are not of too low a bubble-point value; this would signify a too open pore arrangement susceptible to organism passage. On the other hand, tighter filters are seen as a guarantee against violating the threshold value that was experimentally established as being capable of removing 1 × 10⁷ cfu of B. diminuta per square centimeter of EFA. In fact, in a responsible address to dependable quality membrane, manufacturers add a (nonstandard) safety margin to the threshold value of the product that is released to the market.

After meeting the bubble-point requirements and the ASTM bacterial challenge test, the filters intended for sterilizing applications are labeled as being of the 0.2/0.22 μm pore-size rating. No industry standards guide the filter purveyor. Each manufacturer is free to use the numbers and limits he considers appropriate. The bubble-point value set by the filter manufacturer for his filter, whether with safety margin or not, is considered the defining number by FDA investigators. It is beyond questioning by the filter users. Pharmaceutical houses, however, may develop and validate their own LRV–bubble point correlations for the filters they use. This is accepted by regulators.

Figure 9: Correlation of bubble points and organism retention.

Bubble point, organism retention

The bubble point had been shown by several experimenters to correlate directly with the log reduction values (LRV) characteristic of a given filter type confronted by a particular type organism (25, 41–43) (see Figure 9). B. diminuta ATCC 19146 was the organism whose different degrees of retention, expressed as LRVs, was shown to correspond to specific bubble-point pressure levels. Establishing the capability of a filter to sustain a challenge level of 1 × 10⁷ cfu of B. diminuta per cm² of efa, expressed as its bubble point, defines its qualification as a "sterilizing filter." This performance accords with FDA's definition of a sterilizing filter (2). The following equation describes the relationship of the bubble point to the pore's diameter, the dimension most influential on its retention properties:

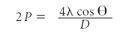
in which θ represents the angle of wetting, λ is the liquid's surface tension, D signifies the pore diameter, and P is the bubble-point pressure in psi.

The equation is correct in indicating the reciprocal relationship of the bubble-point pressure with the restrictive pore width. The critical sensitivity of the wetting action, however, disallows calculating the dimensional size of the pore by way of the equation. Obtaining retentions of 1 × 10⁷ /cm² requires more than a particular pore-size rating. It is dependent also on the suspending fluid's compatibility with both organisms and filter in terms of size alterations of the microbes and pores; on the filtration conditions, especially the differential pressure, temperature and viscosity; and on the numbers, shapes, and size distributions of both the pores and the organisms.

The correlation of a bubble-point pressure level with an (LRV) organism retention of 1 × 10⁷ cfu/cm² EFA was established for the B. diminuta organism cultivated in a prescribed manner (31). This relationship need not necessarily be the same for B. diminuta cultivated to a different size or for other organisms having a different size or size distribution. The more recent recognition that certain organisms, B. diminuta not included, may diminish in size after exposure for a period of time to given drug preparations renders uncertain that a filter found "sterilizing" in one application will necessarily be sterilizing in another (23). No pore-size rating in itself can ensure a sterile effluent. Ralstonia pickettii and Berkholderia cepacia in particular have been studied for their size decrease after undergoing exposure to certain drug preparations.

The point being made is that the filter manufacturer's bubble point was established within a protocol of specified operational steps. Deviations from these may impugn conclusions not so specifically arrived at. The testing is usually outsourced to testing services, intending bias-free results. Correlating the bubble-point values of the various pore-sized filters with the LRVs required for the various filter types using organisms of interest is not a standard protocol. In any case, meeting the manufacturer's bubble point does not exempt the filtration process from the requirement of being validated.

To repeat, the pore-size rating is not assigned on the basis of an actual pore size measurement but according to bubble-point values that have been found to be characteristic of membranes suitable for sterilizing applications. That this identification is warranted is confirmed by a follow-up microbiological assay demonstrating that the membrane does indeed withstand the classic 1 × 10⁷ cfu/cm²B. diminuta confrontation.

Bacterial challenge

An appropriate bacterial challenge is performed to determine the membrane's suitability for the "sterilizing" grade identification before bestowing the 0.2/0.22-μm pore-size rating. The testing is used by each of the individual filter manufacturers using a program based on an American Society for Testing and Materials procedure (27). However, there is not an industry-wide standard for the specifics of the organism challenge test. For example, the organism content of the bacterial test suspension is calculated to furnish the target 1 × 10⁷ cfu challenge per square centimeter of filter surface, but the total organism load is dispensed in from 2 to 20 L of (usually) saline lactose broth. Interestingly, there is reason to believe that the concentration of the organism suspension may influence the sterility of the effluent (1, 44, 45).

All the 0.2/0.22-μm rated membranes of commerce do meet FDA's definition of "sterilizing" membrane, but all do not retain B. diminuta organisms to the same extent. The relative influences of such parameters as bacterial density, volume of test solution, test duration, applied pressure differential, and so forth, have largely not been investigated. Therefore, the distinctions found among different membranes are of an unknown significance. They may reflect the variations in the nonstandard testing.

Consequently, the filters qualified by their fabricators as being suitable for sterilizing trials are labeled and purveyed either as 0.2 or 0.22-μm rated, depending upon their manufacturer. This "pore size" label is affixed to the membranes that exhibit the bubble point, identifying it as a "sterilizing filter." The label signifies that the membrane type did meet FDA's definition of sterilizing filter in the B. diminuta microbial challenge test performed by the filter manufacturer and that it, therefore, has the potential to perform similarly in other filtrations. Whether it would in fact do so would require validation of the filtration process wherein it was used.

Present status

The pore sites of a filter are where the liquid flow manifests itself, where the particle removals take place, and consequently where the throughput volume is defined by the filter blockage. Important though they be, pore sizes are not appraised by direct measurements. They are determined by way of experimentally performed bubble points whose values are transmuted into pore-size ratings. Each filter manufacturer confers its own pore-size designations to its filters. There is no industry standard. In the process, the pore-size ratings, which in any case are not numerical description of the pores' dimensions, are reduced in their significance to parts number in the fabricator's catalogue.

The bubble-point values identify the pores with the largest diameters, those through which suspended particles are most likely to escape capture. In conjunction with information on organism sizes, a coupling of correct pore sizes can be used to attain complete organism removals. It has been established that a correlation exists between bubble-point values and the log-reduction values for the various membrane types. This enables the identification of the filters that can accomplish the removal of 1 × 10⁷ cfu of B. diminuta per square centimeter of EFA, as confirmed by microbiological assays. Such filters are "sterilizing filters" as defined by FDA. This designation qualifies the filter for sterilizing applications. Its actual performance as a sterilizing filter requires validation.

The logic of the above is weakened by the absence of industry standards. The direct assessments of pore sizes are difficult. The converting of bubble-point measurements into pore sizes is encumbered by the complexity of bubble-point values being so highly peculiar to the pairing of the membrane (polymer) type with its particular wetting liquid and for being different again for each pore-size rating of the solid–liquid couple.

It would seem that membrane users would be faced with considerable problems in their choosing the filters best suited for given applications. On the basis of experience, however, the filter user finds that assigned pore-size numbers do offer a level of practical guidance, for example, to particle retentions. Albeit indirectly, bubble points can be interpreted as bearing a relationship to particle sizes. Moreover, although the guidance of formal standards is largely absent, the foci both of filter manufacturers and users are strongly directed to the needs of a federally regulated industry. In this manner, even proprietary actions undergo a peer review. In addition, the development of tools and techniques necessary to pharmaceutical manufacture are shared through numerous technical communications: papers, books, lectures, courses, congresses, and so forth. The need of the industry to meet the requirements set by the regulating agencies promotes shared experiences and cooperative approaches to industry-wide problems. These technical contacts do compensate to an extent for the lack of formal standards. Coupled to a user's experience, the assigned pore-size numbers can offer a level of practical guidance. Nevertheless, it is the bubble points that can be and are measured experimentally. However complicated their values, they can be quantified. It is upon these measurements that, on the basis of experience, the practical applications of filters should be ventured.

Maik W. Jornitz is the group vice-president of global product management, bioprocess of Sartorius North America and is a director of the PDA, Russel E.Madsen, Jr., is the president of The Williamsburg Group, LLC, and Theodore H. Meltzer* is a consultant for Capitola Consulting Company, theodorehmeltzer@hotmail.com Mr. Madsen and Dr. Meltzer also are members of Pharmaceutical Technology's Editorial Advisory Board.

*To whom all correspondence should be addressed.

Submitted: Aug. 11, 2006. Accepted: Oct. 12, 2006.

Keywords: filtration, integrity tests, pore-size ratings

References

1. T.H. Meltzer and M.W. Jornitz, Pharmaceutical Filtration: The Management of Organism Removal (DHI/PDA Publishers, Bethesda, MD, 2006).

2. US Food and Drug Administration, Center for Drugs and Biologics and Office of Regulatory Affairs, Guidance for Industry, Sterile Drug Products Produced by Aseptic Processing: CGMP (FDA, Rockville, MD, 1987 and 2003).

3. F.W. Bowman, M.P. Calhoun, and M. White, "Microbiological Methods for Quality Control of Membrane Filters," J. Pharm. Sci. 56 (2), 222–225 (1967).

4. R.E. Kesting, "Synthetic Polymeric Membranes: A Structural Perspective," in Phase Inversion Membranes (Wiley-Interscience, New York, NY, 3d ed., 1985).

5. P.R. Johnston, Fluid Sterilization by Filtration (Interpharm/CDC, Boca Raton, FL, 2003), Chapters 1, 3, and 9.

6. H.W. Piekaar and L.A. Clarenburg, "Aerosol Filters: Pore Size Distribution in Fibrous Filters," Chem. Eng. Sci. 22, 1399–1408 (1967).

7. R.E. Williams and T.H. Meltzer, "Membrane Structure: The Bubble Point and Particle Retention: A New Theory," Pharm. Technol. 7 (5), 36–42 (1983).

8. F.J. Almgren and J.E. Taylor, "Geometry of Soap Films and Soap Bubbles," Sci. Amer. 235 (l), 82–93 (1976).

9. American Society for Testing and Materials, ASTM Standard F-316: Pore-Size Characteristics of Membrane Filters for Use with Aerospace Fluids (ASTM, West Conshohoken, PA, 1980).

10. T.H. Meltzer, "Chapter 3: Filter Porosity Characteristics," in Filtration in the Pharmaceutical Industry (Marcel Dekker, New York, NY, 1987), pp. 100–122.

11. E. Honold and E.L. Skau, "Application of Mercury-Intrusion Method for Determination of Pore-Size Distribution in Membrane Filters," Science 120, 805–806 (1954).

12. H.M. Rootare and J. Spencer, "A Computer Program for Pore Volume and Pore-Area Distribution Calculations from Mercury Porosimetry Data on Particulate and Porous Materials," Powder Technol . 6, 17–23 (1972).

13. R.E. Williams, Bubble Point Testing and Its Importance to the End User (Filtration Society, Monterey, CA, 1984).

14. J.K. Lee et al., "Pore Size Characteristics of Microporous Membrane Filters by Airflow Porosimetry and Particulate Loading Studies," Swiss Contam. Control 3, 160–164 (1990).

15. J.G. Zahka, "Pore Size Characteistics of Microporous Membranes and Filters by Airflow Porosimetry and Particulate Loading Studies," Swiss Contam. Control 3, 160–164 (1990).

16. B.G. Rogers and H.W. Rossmore, "Determination of Membrane Filter Porosity by Microbiological Methods," Devel. Indus. Microbiol. 2, 453–459 (1970).

17. K.H. Wallhäusser, "Is the Removal of Microorganisms by Filtration Really a Sterilization Method?" J. Paren. Drug Assoc. 33 (3), 156–171 (1979).

18. D.B. Pall, E.A. Kirnbauer, and B.T. Allen, "Particulate Retention by Bacteria Retentive Membrane Filters," Colloids and Surfaces 1, 25–35 (1980).

19. H.G. Schroeder, Selection Criteria For Selection of Sterilizing Grade Filters (Society of Manufacturing Engineers, Philadelphia, PA, 1984).

20. W. Wrasidlo et al., "Effect of Vehicle Properties on the Retention Characteristics of Various Membrane Filters" presented at PDA Spring Meeting, San Juan, PR, 1983.

21. D.L. Tolliver and H.G. Schroeder, "Particle Control in Semiconductor Process Streams," Microcontamination 1 (1), 34–43 and 78 (1983).

22. S.F. Emory et al., "The Effects of Surfactant Type and Latex-Particle Feed Concentration on Membrane Retention," Ultrapure Water 10 (2), 41–44 (1993).

23. S. Sundaram et al., "Application of Membrane Filtration for Removal of Diminutive Bioburden Organisms in Pharmaceutical Products and Processes," PDA J. Pharm. Sci. Technol.53 (4), 186–301 (1999).

24. R.C. Lukaszewiez and T.H. Meltzer, "On the Structural Compatibilities of Membrane Filters," J. Paren. Drug Assoc. 34 (6), 463–472 (1980).

25. D.B. Pall and E.A. Kirnbauer, "Bacteria Removal Prediction in Membrane Filters" presented at 52d Colloid and Surface Symposium, University of Tennessee, Knoxville, TN (1978).

26. H.G. Schroeder, "Rationalization and Valid Scale-Up of Integrity Test Parameters for Sterilizing-Grade Filter Cartridges," Pharm. Technol. 55 (2), 134–142 (2001).

27. ASTM Committee F-838, Bacterial Retention of Membrane Filters Utilized For Liquid Filtration (American Society for Testing and Materials, West Conshohoken, PA, 1988).

28. J. Lindenblatt, M.W. Jornitz, and T.H. Meltzer, "Filter Pore Size Versus Process Validation: A Necessary Debate," Eur. J. Paren. Sci. 7 (3), 67–71 (2002).

29. M.W. Jornitz et al., "Testing for the Optimal Filter Membrane: Required Prerequisites," Genetic Eng. News 24 (13), (2004).

30. J.G. Howard and R. Duberstein, "A Case of Penetration of 0.2 μm-rated Membrane Filters by Bacteria," J. Paren. Drug Assoc. 34, 95–102 (1980).

31. T.J. Leahy and M.J. Sullivan, "Validation of Bacterial Retention Capabilities of Membrane Filters," Pharm. Technol.2 (11), 64–75 (1978).

32. M.J. Gould, M.A. Dawson, and T.J. Novitsky, "Evaluation of Microbial/Endotoxin Contamination Using the LAL Test," Ultrapure Water 10 (6), 43–47 (1993)

33. F.M. Leo et al., "Application of 0.1 Micron Filtration for Enhanced Sterility Assurance on Pharmaceutical Filling Operations," BFS News (a publicaiton of the Pall Corporation), 15–24 (1997)

34. T.H. Meltzer, M.W. Jornitz, and A.M. Trotter, "Application-Directed Selection 0.1 μm or 0.2 μm Rated Sterilizing Filters," Pharm. Technol. 22 (9), 116–122 (1998).

35. EMEA CPMP/QMP/489/95, Note For Guidance in Manufacture of the Finished Dosage Form, 5-6 (April reissue 1996).

36. J.P. Agalloco, "Guest Editorial: It Just Doesn't Matter," PDA J. Pharm. Sci. Technol. 52 (4), 149–150 (1998).

37. Society of Automotive Engineers (SAE), Bubble Point Test. Method, Aerospace Recommended Practice ARP-901 (1968).

38. M.W. Jornitz and T.H. Meltzer, Filtration Handbook: Integrity Testing (PDA, Bethesda, MD; DHI Publishing, River Grove, IL, 2003).

39. D.B. Pall, "Quality Control of Absolute Bacteria Removal Filters," Bull. PDA 29, 192–212 (1975).

40. A. Baszkin, D.J. Lyman, and T.H. Meltzer, "Theoretical Considerations of BubblePoint Measurement: Solid/Liquid Wetting Interaction," Pharm. Technol. Int. 2 (l), 22–31 (1978).

41. P.R. Johnston and T.H. Meltzer, "Comments on Organism Challenge Levels in Sterilizing-Filter Efficiency Testing," Pharm. Technol. 3 (11), 66–70, 110 (1979).

42. W.J. Elford, "The Principles of Ultrafiltration as Applied in Biological Studies," Proceedings of the Royal Society, 112B (London, UK, 1933), pp. 384–406.

43. A.R. Reti, "An Assessment of Test Criteria in Evaluating the Performance and Integrity of Sterilizing Filters," Bull. Paren Drug Assoc. 3l (4), 187–194 (1977).

44. D.C. Grant and J.G. Zahke, "Sieving Capture of Particles by MicroporousMembrane Filters From Clean Liquids," Swiss Contamin. Control 3 (4a), 160–164 (1990).

45. M.L. Roberts and D.J. Valazquez, "Characterizing the Rating and Performance of Membrane Filter for Liquid Applications Using Latex Spheres," Swiss Contam. Quarterly 3, 71–74 (1990).