A Proposed Content-Uniformity Test for Large Sample Sizes

Applications of process analytical technology (PAT) for in-process and/or end-product release testing are attracting wide interest from both regulators and the pharmaceutical industry. Nondestructive measurement techniques such as near-infrared spectroscopy, together with statistical modeling of the obtained spectrum, facilitate fast and precise measures supporting the vision of improved manufacturing process understanding. Such techniques can generate a significant amount of data in real time and thereby open the possibility of improved process control and capability. In such scenarios, the added value of traditional lot-release testing based on a random sample from the batch is questioned.

One of the potential applications of PAT is real-time evaluation of tablet content uniformity. A related issue is the choice of acceptance criteria in light of the increased sample size because the International Conference on Harmonization's (ICH) uniformity of dosage units (UDU) test is based on either 10 or 30 dosage units (1). To address this issue, the Pharmaceutical Research and Manufacturers of America (PhRMA) Chemistry, Manufacturing, and Controls (CMC) Statistics Expert Team (SET) published an alternative to the ICH test in 2006 that could be used as a batch-release specification when testing a large number of dosage units (2). This proposed test was intended as an alternative, not as a replacement, to the ICH UDU test as the official regulatory method. Through interactions with European and American regulators during the second half of 2009, questions about the PhRMA approach were raised; this feedback highlighted the need to modify the test to achieve quality equivalent to or better than the ICH UDU test across the entire test range.

This paper provides a modified version of the test described in the PhRMA CMC SET paper. This modified test is proposed as an alternative test and not as a replacement; it is intended perform similar to or better than the ICH test with the increased sample size. In both the original and modified tests, the proportion of dosage units within 85–115% of label claim (LC) (the "coverage of 85–115% LC") is proposed as the measure of the uniformity of the batch. The acceptance criteria for these tests are based on counting the number of dosage units in the sample outside 85–115% LC and rejecting the batch if that count is too high. The acceptance criteria for these tests are meant to support effective regulatory application of PAT to processing. The tests are nonparametric; simple to implement, use, and regulate; and are applicable to large sample sizes. The modified version of the PhRMA CMC SET alternate test provides the same or better assurance as the harmonized UDU test with respect to the batch's uniformity.

An alternative uniformity of dosage units test

The European Pharmacopoeia, Japanese Pharmacopoeia, and United States Pharmacopeia (USP) contributed to the ICH-harmonized pharmacopeial specification (e.g., USP <905> and Ph. Eur. 2.9.40) for the content UDU, which is based on either 10 or 30 dosage units. The PhRMA CMC SET proposed an alternative to the ICH test to address the issue of developing acceptance criteria for increased sample size due to PAT. The tablet-sampling procedure (e.g., one tablet every three minutes) is established before batch manufacture. The resulting sample size is determined by the sampling procedure, and is larger than 30 dosage units, more likely 100 to 500 units. The PhRMA CMC SET test, called the "Large-N" test in this paper, is a one-tiered counting test for uniformity of dosage units. The nonparametric test is based on counting the number of dosage units outside the 85–115% range of label claim and rejects the batch if that count is outside the set limit.

The number of units (Y) outside the target interval (85–115% LC) is binomially distributed with parameters n (sample size) and p (true proportion outside). When p = 0.048, the quality level (QL) calculated corresponds to 95.2% coverage, associated with a 50% probability of complying with the ICH UDU test. The test translates to finding the largest integer t, called c, such that: c = max{t ; Prob(Y ≤ t || p = 0.048) ≤ 0.5}. Table I provides c for various sample sizes. For example, if 500 units are tested and 23 or fewer results are outside 85–115% LC, the batch passes the test.

Table I: Large-N test acceptance limit for various n.

The test is designed to be more conservative than the ICH UDU test for all levels of batch quality where the probability of accepting a batch is less than 0.5. The acceptance limit, c, depends on the sample size, n.

Figure 1: Large-N (various percent label claim [LC]) operating characteristic curves for n = 100 with International Conference on Harmonizations (ICH) uniformity of dosage units (UDU) test (100% LC). StDev is standard deviation. (ALL FIGURES ARE COURTESY OF THE AUTHORS)

Figures 1 and 2 show operating characteristic (OC) curves for the Large-N test for sample sizes of 100 and 500, respectively, compared to the OC curve for the ICH UDU test when the batch mean is 100% LC. Here, the probability to accept the batch is plotted against batch standard deviation with curves varying by batch mean. If the mean is off target, the standard deviation must decrease to maintain constant coverage; this change results in the shift to the left in the curves. Also, the test is symmetric; a mean of 98% produces the same OC curve as a mean of 102%. Note that for the sample size of 500, the probability of accepting the lot under the Large-N test is slightly higher than passing the ICH UDU test for small standard deviations; this higher probability is expected because increasing the sample size results in a more discriminating test.

Figure 2: Large-N (various percent label claim [LC]) operating characteristic curves for n = 500 with International Conference on Harmonizations (ICH) uniformity of dosage units (UDU) test (100% LC). StDev is standard deviation.

Modified Large-N test

This paper proposes a modification to the Large-N test by increasing the QL from 0.048 to 0.030. This change is demonstrated by OC curves for sample sizes from n = 100 to n = 500. This modification results in a more conservative test than the Large-N test because the number of tablets allowed outside of 85–115% LC is reduced. The value of 0.030 for the QL was chosen because of its performance against the ICH UDU test's OC curve (discussed below) and because the former ICH content-uniformity test allowed 1 tablet (1/30 = 0.033) outside of the 85–115% range. Using the same QL for all sample sizes simplifies the calculation for the acceptable number of tablets.

The modified Large-N test is as follows:

• Define the acceptance limit (c) by calculating 3.0% of n and round it down to the nearest integer. For example, for n = 250, 3.0% of the 250 tablets is 7.5, which will be rounded down to c = 7.

• The batch complies if the number of tablets outside the range of 85.0–115.0% of LC is no more than c.

For sample sizes of 100 to 500 tablets, the proposed alternative test is similar to or more conservative than the harmonized compendia test for UDU. A comparison of the acceptance values using the Large-N and modified Large-N test is provided in Table II.

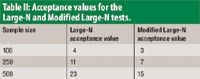
Table II: Acceptance values for the Large-N and Modified Large-N tests.

Figures 3–4 show the OC curves for batch means of 96% and 100% LC and sample sizes of 100 and 500 units for the ICH UDU test, as well as the Large-N and modified Large-N tests. Sample sizes of 100 and 500 units were chosen because they cover the current typical range of sample sizes.

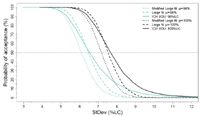
Figure 3: Large-N and modified Large-N operating characteristic curves for n = 100 with International Conference on Harmonizations (ICH) uniformity of dosage units (UDU) test (batch mean = 96% and 100% label claim [LC]). StDev is standard deviation.

The OC curves provide the probability of passing each test at various values of the batch standard deviation (SD). For example, if 100 tablets are tested from a batch with a batch mean of 96% LC (see Figure 3), then for an SD of about 6.4% LC, the probability of passing either the ICH UDU or Large-N test is about 54%, and the probability of passing the modified Large-N test is about 30%. For the same set of curves at a standard deviation of about 4.0% LC, the probability of passing either Large-N test or the ICH UDU test is more than 99.8%. Also, as can be seen in these figures, the test is sensitive to how far the batch mean is from its target (i.e., 100%).

Figure 4: Large-N and modified Large-N operating characteristic curves for n = 500 with International Conference on Harmonizations (ICH) uniformity of dosage units (UDU) test (batch mean = 96% and 100% label claim [LC]). StDev is standard deviation.

Coverage, or the percentage outside 85–115% LC, is a function of the average and the standard deviation of the batch. As the batch mean moves away from target, the standard deviation required to achieve the same probability of passing the test is reduced. Therefore, if a batch is produced off-target, the standard deviation needs to decrease to attain constant coverage. The figures also show that the curves become steeper as the sample size increases; this change is expected because the test becomes more discriminating as the sample size increases. For sample sizes of 100 to 500 units, these curves show that the modified Large-N test is similar to or more stringent than the ICH UDU test.

Discussion of results outside 75–125%

The acceptance criteria for the Large-N and modified Large-N tests do not include a requirement for zero tolerance of tablets outside 75–125% LC, which is a requirement of the 30-sample ICH UDU test. Passing a batch using the Large-N or modified Large-N test with a zero-tolerance criterion depends on the sample size and the true proportion of tablets falling outside 75–125% LC. If the content-uniformity results follow a normal distribution, the following provides justification against a zero-tolerance rule because there is inherent control of the number of tablets outside 75–125% LC through counting the number of tablets outside 85–115%. Adding a zero-tolerance rule would act as a disincentive to collecting the larger sample size, which could result in a deterrent to process understanding.

The modified Large-N test inherently controls the number of tablets outside 75–125% LC through controlling the number outside 85–115% LC. To evaluate the probability of tablets falling outside 75–125% LC, calculations were performed assuming that the content-uniformity results followed a normal distribution. Figure 5 shows the control of tablets outside 75–125% LC through implementation of the modified Large-N test. Note that for a sample size of 500, the probability to accept the batch approaches 0 when 0.1% of the tablets in the batch are outside 75–125% LC for the modified Large-N test, whereas the probability of passing the ICH UDU test is greater than 50%.

Figure 5: Inherent control of the number of tablets outside 75–125% label claim. ICH UDU is the International Conference on Harmonizations uniformity of dosage units test.

The probability of a tablet outside 75–125% LC, the number of tablets inspected until one is found outside 75–125% LC, and the number of lots until this event were calculated under several scenarios (see Table III). These probabilities were determined by 1) assuming a normal distribution that allows a 0.5%, 1%, or 3% chance of an individual tablet to fall outside 85–115% LC or 2) selecting probabilities that required no distributional assumption. For example, a batch with a mean of 98% LC and a 1% chance of a tablet falling outside 85–115% LC has a 0.001473% chance of a tablet falling outside 75–125% LC. Therefore, the expected number of inspected tablets required to find one outside 75–125% LC is (1/0.00001473) or 67,888 tablets. If 10 tablets were sampled per batch, as is the case of the ICH UDU test, then 1 tablet outside of 75–125% LC would be detected, on average, every 6789 batches. Increasing the sample size from 10 to 250 tablets tested per batch results in at least 1 tablet detected outside 75–125% LC every 272 batches. This is simply the result of the laws of probability; increasing the sample size increases the chance of obtaining a value outside of 75–125% LC and has no bearing on the true quality of the batch. The batch quality has not changed, but the batch-rejection rate would. Adding a zero-tolerance requirement for tablets outside 75–125% LC would be a disincentive to increasing the sample size, and hence a barrier to increased process understanding. Note that the probability of falling outside 75–125% LC is all that is needed for the calculation (i.e., the distribution does not matter—only the probability).

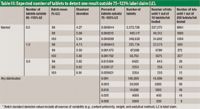
Table III: Expected number of tablets to detect one result outside 75–125% label claim (LC).

Conclusion

The modified Large-N counting test controls the percent of tablets outside 85–115% LC to no more than 3%. As the sample size increases (e.g., from 100 to 500 units), the OC curve becomes steeper, the test's discriminating ability increases, and the level of quality assurance is raised.

The modified test maintains the beneficial properties of the original test. This test has the advantage of being mathematically simple and simple to implement, requiring only a look-up table or a simple mathematical calculation. There is a priori flexibility in selecting the sample size. Similar to the original Large-N test, this test is nonparametric, having the benefit that the test behaves well when the underlying distribution is not normal and testing for normality is not required.

Because this proposed test inherently controls the number of tablets outside 75–125% LC and zero tolerance on this population of tablets could create a disincentive to process understanding and future technology development, the authors recommend careful thought about additional test requirements.

Acknowledgment

The authors wish to thank Fasheng Li, associate director of statistics–Groton at Pfizer (New York) for creating the plots used in the paper. Thank you to Tom Garcia, a research fellow for global regulatory CMC–Groton at Pfizer and Ambarish Singh, associate director of global regulatory sciences–CMC at Bristol-Myers Squibb (New York) for reviewing the paper.

James Bergum* is an associate director of nonclinical biostatistics at Bristol-Myers Squibb, 1 Squibb Drive, New Brunswick, NJ 08903, tel. 732.227.5981, fax 732.227.3005, james.bergum@bms.com. Kim Erland Vukovinsky is a director of nonclinical statistics at Pfizer.

*To whom all correspondence should be addressed.

Submitted: Mar. 15, 2010. Accepted: Apr. 12, 2010.

References

1. USP 33–NF 28 Reissue, General Chapter <905>, "Uniformity of Dosage Units," (US Pharmacopeial Convention, Rockville, MD, Oct. 2010, pp. R–86).

2. D. Sandell, et al., Drug Info. J., 40, 337–344 (2006).