Process Patent Protection: Characterizing Synthetic Pathways by Stable-Isotopic Measurements

Measurements of the abundances of naturally occurring stable isotopes in pharmaceutical materials can be used to quantitatively characterize the sources of the products and the synthetic processes used to produce them (1, 2). Since 2001, industry has had increased interest in the use of stable-isotopic analyses in industrial pharmacology. The results of reconnaissance studies have led to detailed examinations of the mechanisms that determine the observed isotopic compositions. The initial, observational work started with a field study to differentiate individual batches of drug products (3). This work led to an investigation of stable isotopic analyses as monitors of process consistency during drug manufacturing. In that study, various batches of two over-the-counter analgesic drugs were analyzed for isotopic consistency. Batch-to-batch variations in stable-isotopic compositions indicated that either the sources of the starting materials or aspects of the manufacturing process had changed.

To follow up, blind isotopic studies were initiated by the Division of Pharmaceutical Analysis of the US Food and Drug Administration (St. Louis, MO) to determine whether isotopic analyses of drugs could be used to identify the manufacturing source. In the first of two studies, researchers demonstrated that stable-isotopic analyses could distinguish among individual manufacturers and batches of four active pharmaceutical ingredients (APIs) produced by nine manufacturers (4). A second study examined the ability of stable-isotopic analyses to differentiate manufacturer sources of an API as it was transported across national borders. All six manufacturers of the API (naproxen) were recognized successfully (5).

The results demonstrate the utility of stable-isotopic analysis for monitoring and identifying pharmaceutical products and manufacturers, thus providing a new tool for product security and drug authentication. It also can be used to monitor process consistency (i.e., the changes or modifications that occur during the various synthetic steps in a pharmaceutical drug-manufacturing process).

Another area that is of interest for stable-isotopic analysis is its use as a forensic tool in cases of process patent protection. Two sets of variables are pertinent: the stable-isotopic compositions of the starting materials and synthetic intermediates, and the isotopic fractionations that occur during production. Understanding the extent of isotopic fractionation that occurs at each step in a synthetic route can provide valuable insight into which process was used.

In this article, the authors focus on means of constraining the major variables (e.g., the precursors and synthetic pathways) that determine the isotopic compositions of pharmaceutical materials. Ideally, isotopic compositions of pharmaceutical products will be predictable, and the synthetic processes used will be recognizable. In a broader sense, these studies of process authentication bear on process-patent protection and on process analytical chemistry, including process consistency.

Experiment

To fully elucidate the isotopic fractionations that occur between reaction steps, it is ideal to have at least 0.1 mg of all intermediates and products for each stable-isotope ratio being measured.

Analyses. Carbon and nitrogen isotope analyses. For analyses of ¹³C and ¹⁵N ratios, ~0.1-mg solid samples were weighed and placed into tin cups that were crimped tightly closed. The analytical system used was an elemental analyzer–isotope-ratio mass spectrometer (EA-IRMS), and consisted of an elemental analyzer (EA, Carlo Erba 1108, Carlo Erba, Italy), a continuous-flow interface (Conflo II, Thermo Fisher Scientific, Bremen, Germany) and an isotope-ratio mass spectrometer (Finnigan MAT Delta Plus XL, Thermo Electron, Waltham, MA). The oxidation furnace of the EA was operated at 1020 °C, the reduction furnace temperature was at 650 °C, and the chromatographic column was heated at 60 °C. Isotope ratios are reported in terms of δ¹³C values relative to the international Vienna Pee Dee Belemnite (VPDB) standard and δ¹⁵N values relative to the international Air, both of which are IAEA standards. The δ notation is explained later in this article.

Sulfur isotope analyses. Individual solid samples of 0.08–0.10 mg were mixed with 0.5 mg V₂O₅, weighed into tin cups, and sealed. The analysis was accomplished using a second EA–IRMS, that was composed of an elemental analyzer (Eurovector, model 3000r), an interface (Dilutor, Micromass Ltd.), and an isotope-ratio mass spectrometer (Isoprime, Micromass Ltd.). The oxidation furnace of the EA was operated at 1030 °C. The gas flows were 10 mL O₂/min and 150 mL He/min. Isotope ratios are reported in terms of δ³⁴S values relative to the international Canyon Diablo troilite (CDT) standard.

Hydrogen and oxygen isotope analyses. Before analysis, solid samples were equilibrated for several days at ambient temperature with water vapor by exposure to the laboratory atmosphere to fully exchange labile H/D sites and any water present in a hydrated form (6–9). Following equilibration, ~0.2 mg-samples were weighed into silver boats which were then crimped tightly. For solid samples, researchers used a thermal conversion–elemental analyzer (TCEA, Finnigan, Thermo) interfaced to an isotope-ratio mass spectrometer (TCEA–IRMS, Finnigan Delta Plus XL, Thermo). The same system was used for liquid samples, but a direct liquid-injection port was fitted in place of the autosampler. The TCEA pyrolyzes the samples at 1350 °C to quantitatively generate H₂ and CO, which are separated chromatographically at 85 °C. Isotope ratios are reported in terms of δD values and δ¹⁸O values relative to the international Vienna Standard Mean Ocean Water (VSMOW) standard.

Terminology for isotopic relationships between precursors and products. Isotopic calculations are based on only two systems of equations. The first system uses mass balances and the second involves integrated forms of rate equations that pertain only to kinetically controlled isotopic fractionations. Equations describing mass balances are exact when cast in terms of fractional abundances [e.g., ¹³C/(¹²C + ¹³C)]. In contrast, the assessments of differential rates are based on isotope ratios (e.g.,¹³C/¹²C). When these systems must be blended, either approximations or equations with multiple terms are used. Additional information is available in reference 10.

The relevant isotopic parameters are stoichiometry (n), isotopic abundance (δ), the magnitude of the isotopic effect (ε), and a variable related to the conversion of reactants to products (f). The following is an explanation of these terms.

Stoichiometry (n). The symbol, n, represents the stoichiometry of the reaction, specifically the number of atoms of a given element (e.g., carbon) in a molecule involved in the reaction

Isotopic abundance (δ). A measure of isotopic abundance, δ, is usually reported as the difference in parts per thousand, or permil (‰), from an international standard. The δ can be negative or positive, depending on whether the sample is enriched or depleted in the heavy isotope relative to the standard. In the case of carbon, for example, the difference is calculated as: .

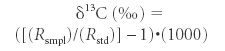
in which R_smpl is the ¹³C/¹²C ratio of the sample and R_std is the ¹³C/¹²C ratio in the standard. Thus, δ is linearly proportional to the isotopic ratio in the sample. Standards are available from the International Atomic Energy Authority and a standard for each isotope is used to determine the zero point of an abundance scale for that isotope. Standards include average seawater for H and O, calcium carbonate for C, air for N, and a meteorite for S. When the sample is depleted in the heavy isotope relative to the standard, δ is negative. When the sample is enriched, it has a positive value. If it has the same isotopic abundance, then δ = 0 (11).

Magnitude of the isotopic effect (ε). The ε value is a measure of the magnitude of an isotope effect. Its value depends on details of the reaction and on the relative mass difference between isotopes. Effects are largest for D versus H and smaller for heavier elements. In general, the values of ε are specific to individual positions within the molecules involved. They are largest at the reaction site, much smaller at neighboring positions, and usually not measurable elsewhere. Like δ, ε relates to the isotopic difference between two materials (e.g., reactant and product) and is usually expressed in permil. For kinetic isotope effects in the system used in this article, ε = –10‰, which means that a reaction site bearing the heavy isotope reacts 10 parts per thousand (or 1%) more slowly than a site bearing a light isotope. For equilibrium isotope effects, ε_A/B = 15‰ would mean that, at equilibrium, A was enriched in the heavy isotope by 15 parts per thousand relative to B. In this instance, A and B refer to specific atomic positions that can be related by a chemical equilibrium.

Variable related to the fractional conversion of reactants to products (f). The f is a measure of the reaction's progress. It is the most important variable governing fractionations caused by isotope effects. Its value ranges from 1 to 0 and depends on factors such as temperature, pressure, or the availability of reactants. In equilibria (A ⇔ B), f indicates the position of the equilibrium, with f_B = 1 indicating a complete conversion to B and, at any position, f_A + f_B = 1. In irreversible reactions, f_X indicates the portion of reactant X which remains unconsumed, with f_X → 0 as the reaction proceeds to completion.

The precision of isotopic analyses is typically calculated by two methods. Pooled standard deviations of raw data are typically computed from sets of duplicate or triplicate measurements (12). From those pooled standard deviations, standard deviations of mean values pertaining to specific substances are calculated. More specifically, the standard deviation of a mean value is the pooled standard deviation divided by n ^½ , in which n is the number of measurements performed on a given sample (13). For carbon, nitrogen, oxygen, and sulfur, the resulting 95% confidence intervals for a result are typically in the range of ±0.1 to ±0.4‰. For hydrogen, the 95% confidence interval is typically ±3‰.

Discussion

The precise quantitation of stable-isotopic compositions in pharmaceutical intermediates and products requires both mass balance and isotopic fractionation equations that are applicable to both single and multistep reaction sequences. We start from the most basic requirement of mass balance and then consider isotopic fractionations in a single reaction. Finally, we discuss applications to the protection of process patents.

Mass balance. For A + B → C, in which reactants A and B are quantitatively converted to product C, two mass balances can be written:

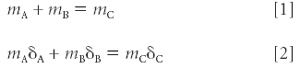
in which, m_A, m_B, and m_C are molar amounts of carbon (or any other element) in A, B, and C and the isotopic compositions of that carbon (or any other element) in A, B, and C are given by δ_A, δ_B, and δ_C. Equation 1 is a mass balance (i.e., carbon in = carbon out) whereas Equation 2 is an isotopic mass balance (¹³C in = ¹³C out). Under the conditions postulated (quantitative conversion), the isotopic composition of C can be computed from those of A and B (10, 11).

Isotopic fractionation. For isotopic fractionations, calculations must take into account factors such as reaction completeness and isotope effects. These effects will cause the isotopic composition of C to differ from that computed using the mass balance equation and assuming quantitative conversion of reactants to products.

To provide a concrete example, assume that A is present in excess whereas B, the limiting reactant, is quantitatively converted to product. In that case:

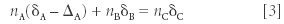
in which n_A, n_B, and n_C represent the numbers of carbon atoms (or any other element of interest) in A, B, and C. Because A is not quantitatively converted to product, the isotopic compositions of the A-derived positions in C can differ from those in the initial reactant. In this case, the isotopic offset is expressed as ΔA. As Figure 1 shows, its value depends on the isotope effects and on the fraction of A that remains unconsumed. If the reaction conditions, particularly the magnitude of the excess of A, are consistent, ΔA will be constant. Because the n values are known exactly, ΔA can be determined from Equation 3 after isotopic analysis of the reactants and product (i.e., determination of δ_A, δ_B, and δ_C).

Values of δ_A, δ_B, and δ_C do not affect the values of ΔA. Accordingly, once ΔA is known for a given reaction and a set of conditions, it is necessary only to know two of the δ values to compute the third. Thus, for example, when ΔA, δ_A, and δ_B, are known, the isotopic value of the product (δ_C) can be calculated.

If neither A nor B is consumed completely during the course of the reaction, and if the rate of the chemical reaction (or position of the chemical equilibrium) is sensitive to isotopic substitution on both reactants, it is necessary to consider values of ΔA and ΔB:

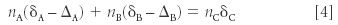
If reaction conditions cannot be manipulated so that f_A and f_B (and thus Δ_A and Δ_B) can be independently driven to completion (i.e., zero), it will be possible to determine only the sum, n_AΔ_A + n_BΔ_B. From theoretical considerations (14), Δ_A and Δ_B can be evaluated separately for all values of f_A and f_B if the isotope effects are known.

Of course, isotopic fractionations such as those previously described accumulate during the different steps of a multi-step synthesis scheme. They can, however, be individually and systematically differentiated, not only for multiple reactants but also for multiple isotopes. To provide an example, consider carbon-isotopic fractionations in a hypothetical four-step sequence:

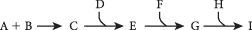
Figure 2 shows illustrative carbon skeletons for reactants and products. Table I summarizes the pertinent quantities. The carbon numbers (n₁, n₂), initial isotopic compositions (δ₁, δ₂), fractions of reactants remaining unconsumed (f₁, f₂), and summed isotope effects (Σε₁, Σε₂) were chosen to be representative of a typical synthetic scheme. All isotope effects were assumed to be kinetic. Values of δ_P* (i.e., the isotopic compositions that would be observed if isotopic fractionations were absent) were calculated using Equation 4 with Δ_A = Δ_B = 0; that is, the simple mass balance Equations 1–2. Values of δ_P, the isotopic compositions that would actually be observed for the successive products, were calculated using exact forms of integrated rate equations (14).

Table I: Properties of a four-step synthetic sequence.*

This example illustrates the interplay of the four factors that control the isotopic compositions of manufactured products, namely the stoichiometries and isotopic compositions of the starting materials, isotope effects associated with the synthetic reactions, and the degree to which conversions of precursors to products are quantitative. The isotopic compositions of all products are dominated by the initial isotopic abundance of the precursor materials and are modulated (viz., depleted) by the degree of completion (f) and the magnitude of any isotopic effects (ε, see Figure 3a). Figure 3b shows a plot that summarizes the difference between the isotopic compositions that are predicted and those that would be observed in the absence of isotope effects (δ_P* – δ_P). The last two columns of Table I shows these values. In the first synthetic step, isotope effects on reactant B are rather large, but that reactant is consumed almost completely. The resulting isotopic fractionation is less than 1‰ (the larger value shown in Figure 3b pertains to the product and reflects fractions affecting both reactants).

In the second step, a large isotope effect and poor conversion of reactant C lead to a large isotopic fractionation at the reaction site. Fractionation is diluted, however, now that the product contains 14 carbon atoms. As shown in Figure 3b, the overall difference between real and hypothetical unfractionated products is barely doubled. In the remaining steps, when isotope effects are moderate and the consumption of reactants is relatively efficient, isotopic fractionation declines.

Applications in the pharmaceutical industry

Measuring and tracking isotopic fractionations in synthetic pathways used to prepare pharmaceutical products has potential uses in process analytical chemistry and for the protection of process patents. In process analytical chemistry, the matrix of information obtainable provides a complete isotopic description of pharmaceutical materials from starting materials through synthetic intermediates to final products. Starting materials reacted under consistent conditions with isotopically controlled reagents should always produce products of known isotopic composition. Divergences from the predicted isotopic pathways suggest uncontrolled variables in pharmaceutical manufacture and provide insight into process consistency. When the goal of process analytical chemistry is understanding manufacturing processes, the complete stable-isotopic record of synthesis summarizes many key process variables: reaction rate as affected by the synthetic pathway, reaction rates, temperature, pressure, compound concentration, and so forth.

Observations such as those summarized in Figure 2 can be used to monitor synthetic processes. Once the sequence of isotopic compositions characteristic of a process is known, any variations must be traceable to (1) utilization of reactants from some new source (δ), or (2) variations in the extent to which reactants are converted to products (f), or (3) changes in the reactions used (ε). In this way, very simple and inexpensive analyses integrate information (n, δ, f, ε) of considerable value for understanding the synthetic pathway employed and for process control.

Understanding isotopic process parameters can be useful in process-patent protection applications. With a full description of a given pharmaceutical synthetic pathway, the isotopic differences between any precursor and another precursor or a precursor and the final product can be predicted or at least provisionally understood in principle. This provides valuable insight into which synthesis process was used. Consistencies in expected isotopic matrix values can provide important information about process counterfeiting.

Summary

Stable-isotopic fractionations resulting from isotope effects are usually large compared with the precision of isotopic measurements. These fractionations significantly modulate the isotopic compositions that would be observed if isotopic compositions of starting materials were the only controlling factor. The fractionations depend not only on the magnitudes of isotope effects associated with the synthetic reactions used, but also on reaction conditions, specifically those affecting the extent to which reactants are converted to products. Because of these phenomena, the final isotopic composition of a manufactured product is directly related to the synthetic scheme used. Subsequently, process analytical chemistry using stable-isotopic analysis can be useful not only for product authentication, but also for process monitoring or reconstructing the processes by which the product was synthesized.

Acknowledgments

The concepts of both stable-isotopic product and process authenticity are covered under either existing or pending patents in either the United States or in G7 countries and Australia owned by Molecular Isotope Technologies LLC. This paper is dedicated to medical-surgical team at UCSD's Thornton Medical Center.

John P. Jasper, PhD,* is the chief scientific officer of Nature's Fingerprint Authentication, a Division of Molecular Isotope Technologies LLC, 8 Old Oak Lane, Niantic, CT 06357, jpjasper@naturesfingerprint.com, tel. 860.739.1926, fax 860.739.3250. Larry E. Weaner, PhD, is a senior research fellow with Johnson & Johnson Pharmaceutical Research and Development (Spring House, PA). John M. Hayes, PhD, is a scientist emeritus with Woods Hole Oceanographic Institution (Woods Hole, MA).

*To whom all correspondence should be addressed.

Submitted: Nov. 3, 2006. Accepted: Dec. 27, 2006.

Key words: isotope fractionation, process analytical chemistry, PAC, process patent protection, stable isotopes, synthesis.

References

1. J.P. Jasper, "Pharmaceutical Security: Using Stable Isotopes to Authenticate Pharmaceutical Materials," Tablets and Capsules 2 (3), 37–42 (2004).

2. J.P. Jasper, R.C. Lyon, and L.E. Weaner, "Stable Isotopes Provide a New PAT [Process Analytical Technology] Tool," Pharm. Mfg. 4 (5), 28–33 (2005).

3. J.P. Jasper et al., "Stable Isotopic Characterization of Analgesic Drugs," Pharm. Technol. 28 (8), 60–67 (2004).

4. J.P. Jasper et al., "Stable Isotopic Characterization of Active Pharmaceutical Ingredients," J. Pharm. Biomed. Anal. 35, 21–30 (2004).

5. A.M. Wokovich et al., "Stable Isotopic Composition of the Active Pharmaceutical Ingredient (API) Naproxen," Pharm. Biomed. Anal. 38, 781–784 (2005).

6. A. Schimmelman, M.D. Lewan, and R.P. Wintsch, "D/H Isotope Ratios of Kerogen, Bitumen, Oil, and Water in Hydrous Pyrolysis of Source Rocks Containing Type-I, -II, -IIS, and -III kerogen," Geochim. Comsochim. Acta 63 (22), 3751–3766 (1999).

7. L.I. Wassenaar and K.A. Hobson, "Improved Method for Determining the Stable-Hydrogen Isotopic Composition (δD) of Complex Organic Materials of Environmental Interest," Environ. Sci. Technol. 34, 2354–2360 (2000).

8. P.E. Sauer et al., "Compound-Specific D/H Ratios of Lipid Biomarkers from Sediments as a Proxy for Environmental and Climatic Conditions," Geochim. Comsochim. Acta 65 (2), 213–222 (2001).

9. Y. Chikaraishi and H. Naraoka, "Compound-Specific δD-δ¹³ C Analyses of n-Alkanes Extracted from Terrestrial and Aquatic Plants," Phytochem. 63, 361–371 (2003).

10. J.M. Hayes, "An Introduction to Isotopic Calculations," (National Ocean Sciences Accelerator Mass Spectrometry Facility, Woods Hole, MA, 2004), http://www.nosams.whoi.edu/docs/IsoCalcs.pdf, accessed Jan. 22, 2007.

11. J.P. Jasper, "The Increasing Use of Stable Isotopes in the Pharmaceutical Industry," Pharm. Technol. 23 (10), 106–114 (1999).

12. J.M. Hayes, "Practice and Principles of Isotopic Measurements in Organic Geochemistry," (National Ocean Sciences Accelerator Mass Spectrometry Facility, Woods Hole, MA, 2002), http://www.nosams.whoi.edu/docs/IsoNotesAug02.pdf, accessed Jan. 22, 2007.

13. J.P. Jasper, "Quantitative Estimates of Precision for Molecular Isotopic Measurements," Rap. Comm. Mass Spec., 15, 1554–1557 (2001).

14. K.M. Scott et al., "Optimal Methods for Estimating Kinetic Isotope Effects from Different Forms of the Rayleigh Distillation Equation," Geochim. Cosmochim. Acta, 68 (3), 433–442 (2004).