Error Assessment of Drug Concentration in Multicomponent Pharmaceutical Mixtures

PM Images/DigitalVision/Getty Images

The increasing complexity of drug products, particularly those with a narrow therapeutic index requires stringent quality checks by the manufacturers. It is important that dosage forms produced by the pharmaceutical industry are in accordance with GMP guidelines. The United States Pharmacopeia recommends using analytical balances that are at least 1000 times more sensitive than the measured quantities in analytical assays (1). Even so, no method has been developed to calculate the error associated with drug dose in multicomponent pharmaceutical mixtures.

A method was published (2) that can, in principle, calculate the maximum potential error of drug concentration in pharmaceutical mixtures composed of an infinite number of ingredients that have been measured on an infinite number of analytical balances. The emphasis of that work was to better understand the error variation in drug dose as a function of the number of ingredients and balance sensitivities (2). In this article, the author initially used the published method, based on differential calculus, to determine the maximum potential error of drug concentration and drug dose in a multicomponent pharmaceutical mixture. Based on estimates from the method, dosage forms can be prepared within the maximum allowable error in drug dose.

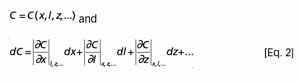
To determine the maximum potential error of drug concentration in multicomponent pharmaceutical mixtures, the following function that describes the variation of the drug in a mixture (2) was used (see Equation 1):

where C is drug concentration, x is the drug, and l, z,… are excipients.

The maximum potential error associated with C can be found from the total differential of the above function, which can be determined by partial derivatization of Equation 1, because the drug and excipients are all independent variables. This calculation is shown in Equation 2:

Executing the algebra of partial derivatives results in:

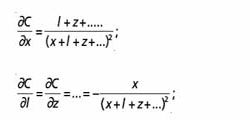
Factoring out C and taking the absolute values of each parameter results in Equation 3:

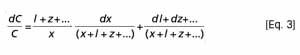
The total differential gives a linear approximation of a change in the dependent variable C, which is derived from (infinitesimal) changes in all of the independent variables. In other words, dC, dx, dl, dz,… are the uncertainties or the maximum potential errors associated with the measurement of the respective quantities C, x, l, z, etc. From the practical point of view, dx, dl, and dz are the sensitivities of the weighing balances used to measure the respective quantities x, l, and z. Given the quantities of drug(s) and excipients of a master manufacturing formula and the sensitivities of the respective weighing balances, one can use Equation 3 to determine dC/C as the maximum potential error of the drug concentration in the mixture.

MethodExample. The following formulation is given to prepare 12 unit doses, each weighing 300 mg, within a maximum allowable error (MAE) of 5 % in the unit dose. Drug (x), excipients (l, w, z), and unit dose (M) were measured on different balances with sensitivity requirements (see Table I).

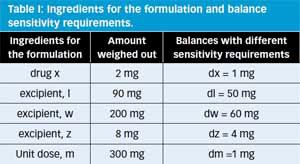
Solution. The quantities needed to fill 12 unit doses are 24 mg, 1080 mg, 2400 mg, 8 mg, and 3600 mg of drug x; excipients l, w, and z; and the powder mixture, respectively. First, the error (m) associated with the unit dose measurement is determined using Equation 4:

The error is subtracted from the MAE to calculate the adjusted MAE related to the drug concentration in the powder mixture:

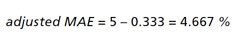
The same Equation 4 is used to determine the error associated with the drug dose when the drug is weighed directly on the balance:

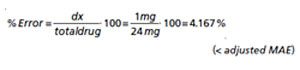
According to these calculations, the maximum potential error resulting from the measurement of the drug on the given analytical balance is less than the adjusted MAE, and one could presume that this is indeed the maximum potential error associated with the drug in the dosage forms.

The reliability of this answer is crosschecked by first defining a key function that expresses the drug concentration in the mixture (Equation 1’) (2).

The two extreme values that are distributed around the average drug concentration can be calculated empirically using Equation 5:

The minimum and maximum potential concentrations around the average value are: 0.006194 < 0.006667 < 0.007169, and the percent error associated with each concentration is shown as follows:

A step further can be taken to calculate the drug amount in each unit dose. D_-, D₊, and D_ave, respectively, represent the minimum, maximum, and average drug mass in each unit dose and are calculated by multiplying the mass of powder mixture (M = 300 mg ± 1 mg) with the corresponding drug concentration as follows:

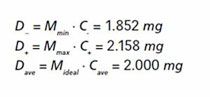
The percent relative errors are:

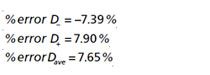
Notice that the average error in drug dose equals the error associated with the unit dose measurement (m = 0.33 %) plus the error of the drug concentration in the mixture (C_ave = 7.31%).

Alternatively, because drug and excipients are measured on balances of different sensitivity, the maximum potential error associated with the drug concentration in the mixture can be calculated more easily using Equation 6, which is the total differential of Equation 1ʹ (2).

Note that dC/C is called the relative error. It is unitless and is related to the absolute error dC through Equation 7:

The absolute error is, dC=C · 0.07306=0.006667 · 0.07306=4.870 · 10^-4 g/g, where C is equal to the ideal drug concentration C_ideal or C_ave. Both C and dC have units of concentration, and accordingly, the weight concentration of the drug in the powder mixture is in the range:

The bounds of drug quantity in each unit dose are calculated by multiplying the minimum and maximum concentration values of the drug with the minimum and maximum mass of the unit dose. Thus, the minimum and maximum drug quantities are D_- = 1.848 mg and D₊ = 2.153 mg, and the percent errors associated with these two quantities are:

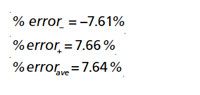
Indeed, the error associated with the drug concentration in the mixture is larger than the maximum allowable one. If no other more sensitive analytical balances are available in the premises, the aliquot method of weighing needs to be used to prepare the pharmaceutical formulation within the limits of the MAE. To reduce the error associated with the measurement of drug x, the amount of drug x weighed on the specified balance needs to be increased. Equation 6 is used to determine the least allowable weight (LAW) within the limits of the adjusted MAE of 4.667 % (or dC/C = 0.04667) in the powder mixture. However, changing the amount of drug x in the mixture would require proportional changes of the excipient quantities, so that the ratio of drug x to the other ingredient remains the same (batch process scale-up). An additional equation is needed, which calculates the ratio of drug in the final mixture (Equation 8 from Eq. 1’).

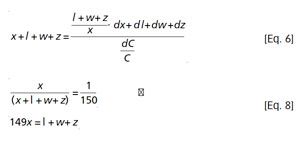
Substituting Equation 8 into Equation 6:

The excipients were calculated by scaling up the quantities of the master formula using simple dimensional analysis, I = 1691mg, w = 3757mg, and z = 150.3mg.

The total mixture, which is also the stock powder (S), can be calculated:

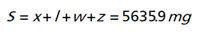
It can be verified that the answer is within the limits of the maximum potential error using the empirical Equation 5 once more. The minimum and maximum drug concentrations are 0.006362 and 0.006984, and the percent error associated with the drug concentration in the mixture are:

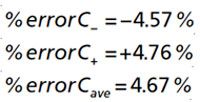
The drug amount in the unit dose is D_ave = 2.00 mg, D- = 1.90 mg, and D₊ = 2.10 mg, and the errors associated with the drug dose are:

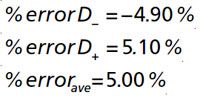
For an infinite number of ingredients (and analytical balances), one could follow exactly the same process to calculate the MAE and the LAW of the drug or any other ingredient using Equation 1 and Equation 3 (2, 3).

Conclusion
It has been demonstrated that only the calculation of the potential error associated with the drug measurement, without accounting for the error associated with the measurement of other excipients present in the mixture, does not provide a true estimate of the uncertainty associated with the drug concentration in pharmaceutical mixtures. The method described in this communication can be used by a research formulator to provide accurate evaluation of the maximum potential error of the drug dose in pharmaceutical mixtures and finished products composed of an infinite number of ingredients measured on multiple balances of variable sensitivities.

References
1. USP 29-NF 24 General Chapter <41> “Weights and Balances” (US Pharmacopeial Convention, Rockville, MD, 2006), p. 2499.

2. M. Savva, Anal Chem Insights 1 (1) 1-3 (2006).

3. M. Savva, “Methods and Products for Error Determination of Drug Dose in Pharmaceutical Mixtures,” US patent 7831393 Nov. 2010. 

Article DetailsPharmaceutical Technology
Vol. 40, No. 2
Pages: 26–29

Citation:
When referring to this article, please cite it as M. Savva, “Error Assessment of Drug Concentration in Multicomponent Pharmaceutical Mixtures," Pharmaceutical Technology 40 (2) 2016.