Achieving Zero-Order Release Kinetics Using Multi-Step Diffusion-Based Drug Delivery

Abstract: Zero-order release, in which a drug is released at a constant rate, is the ultimate goal of all controlled-release drug-delivery mechanisms. It leads, in principle, to the best control of plasma concentration and offers several advantages, including improved patient compliance and reduction in the frequency of drug administration. Although there are several techniques that formulation scientists can use to achieve zero-order release, most of them are complex, expensive, time consuming, and difficult to manufacture. In addition, most formulation techniques result in, at best, first-order release. This article provides a novel approach of obtaining the zero-order release of a freely water-soluble active drug by adding methacrylic acid copolymers alone or in combination with cellulose polymers in a tablet matrix.

Oral delivery is the most widely-used method for administering therapeutic agents for systemic effects, because it is both convenient for patients and cost effective for drug manufacturers (1). Oral drug-delivery systems that immediately release the active agents into the gastrointestinal tract are associated with several disadvantages, such as:

• Drugs with a short half-life have to be administered frequently, which increases the likelihood of poor patient compliance.

• The drug’s plasma concentration typically fluctuates, which could lead to over or under medication should it rise or fall beyond its therapeutic range.

• Often, an excess amount of the active agent has to be administered so that even if it is metabolized, degraded, or excreted, there will be enough drug to maintain an effective dose for a prolonged period--an approach that is not only wasteful, but that can lead to overdosing side effects.

• Fluctuating plasma concentrations could precipitate adverse reactions, especially among drugs that have less than a two-fold difference between the minimum toxic dose and the minimum effective dose (i.e., narrow therapeutic index) (2, 3).
  

A more desirable drug delivery system, such as controlled-release delivery, would thus dispense a therapeutic agent at a predetermined rate for a specified period of time (3-6). Typically, pharmaceutical drugs are coated with acrylic or cellulosic polymers so that the API can diffuse through the coating to produce controlled release of drugs (7-10). Zero-order release can be achieved, in principle, by surrounding the tablet core with a polymer membrane that is permeable to both drug and water. When the core becomes hydrated in the gastrointestinal tract, the drug will dissolve until it reaches its saturation concentration (i.e., solubility). The core serves as a saturated reservoir of drug. Drug release occurs when the drug permeates into the membrane from the reservoir and then diffuses across the membrane into the gastrointestinal fluid (11).

The coating, however, can break prematurely in vivo, typically in fed conditions, thus precluding zero release and resulting in a dose-dumping effect. It was hypothesized that this limitation could be overcome by producing multi-diffusion kinetics for the drug in matrix-type formulations.

Kinetic models
To analyze the mechanism of drug release-rate kinetics, the results of in-vitro release profiles can be plotted in various kinetic models, such as zero order, first order, Higuchi, and Korsmeyer-Peppas. Mathematical modeling increases understanding of the release mechanism and in turn helps to reduce the number of experiments required to optimize the formulation. The mathematical models used in this paper are described as follows.

The diffusion-based drug release can be either Fickian or non-Fickian. In Fickian diffusion, the rate of release is independent of the drug concentration in the tablets.

Zero-order release kinetics describe systems where the drug release rate is constant over a period of time. The equation for zero-order release is shown in Equation 1, where Q_tis the cumulative amount of drug released at time t, Q₀is the initial amount of drug, K is the release kinetic constant, and t is the time at which the drug release is calculated or measured (12):

In non-Fickian diffusion, several factors can affect the drug release, and based on these factors, the release can be predicted by several mathematical models as follows.

First order. In typical first-order release kinetics, the drug release rate depends on its concentration. The first order release equation can be explained as shown in Equation 2 (12):

Hixson and Crowell. Hixson and Crowell proposed a correlation between drug release from the particle and surface area and diameter area of the particle. The proposed equation shows that the drug release from the particle is proportional to the cubic root of its volume, and derived an equation that can be described as shown in Equation 3 (13):

Higuchi. Higuchi developed several theoretical models to study the release of water soluble and low soluble drugs incorporated into semi-solid and/or solid matrixes. Mathematical expressions were obtained for drug particles dispersed in a uniform matrix behaving as the diffusion media. To study the dissolution from a planar system having a homogeneous matrix, Equation 4 was obtained, where Q∞ is the total amount of drug released (14):

Korsemeyer and Peppas. Korsemeyer and Peppas developed a simple, semi-empirical model that relates exponentially the drug release to the fractional release of  the drugs. Hence, the final equation can be written as shown in Equation 5, where n is the diffusional exponent (15):

Sahlin and Peppas. The Sahlin-Peppas equation is a modification of the Korsmeyer-Peppas equation. When the drug is released due to a combination of diffusional and relaxational mechanisms during the non-Fickian release process, the Sahlin-Peppas equation provides an estimation of drug release. The equation can be written as shown in Equation 6 (16), where m is the Fickian diffusion coefficient, K1 is the release kinetic constant for non-Fickian diffusion, and K2 is the release kinetic constant for polymer relaxation:

In this article, a formulation strategy that uses methacrylic acid copolymers alone or in combination with cellulose polymers in a matrix-type tablet is described. In such a formulation, the API will follow the multi-diffusional pathway to produce a zero-order release from the matrix tablet. If necessary, the matrix tablet can be coated with controlled-release polymers to a minimal weight gain to further control drug release. The objective of this study was to achieve a formulation based on multi-step diffusion of drug to obtain a pH-independent, zero-order release for a freely water-soluble drug. Mathematical modeling was used to investigate the drug release kinetics, which helped in minimizing the number of experiments required to optimize the formulation and process.

Materials and methods
Eudragit RS/RL and hydroxypropyl methylcellulose (HPMC) were used as the release-controlling polymers in this study. Eudragit is the tradename of copolymers derived from esters of acrylic and methacrylic acids. Compared to cellulosic polymers, they are more flexible. Eudragit RL is more drug permeable than Eudragit RS, but both form a non-eroding network by entangling drug molecules when heat cured. By using a combination of these polymers, the desired drug release through the release-controlling polymer network can be obtained (17). HPMC is a swellable hydrophilic polymer known for rapid hydration, good tableting compression, and desirable gelling characteristics. HPMC forms a gelled network when hydrated and the drug release is controlled by the diffusion rate based on the HMPC molecular weight (18).

Apart from the release-controlling polymers, other excipients such as fillers, gliding agents, and non-tacking agents were used in the formulations as listed in Table I.

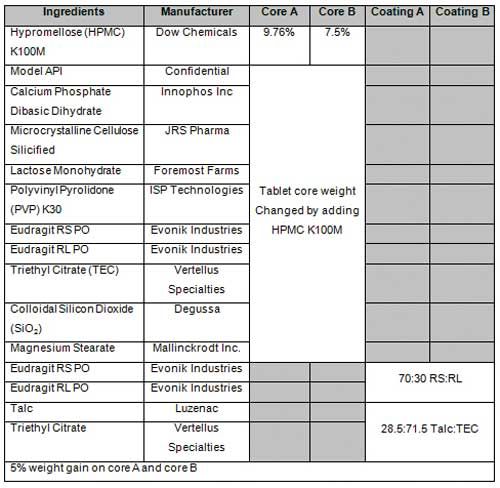
Preparation of core tablets. Two sets of matrix tablets were prepared. For each set, a freely soluble active model drug and appropriate ratios of methacrylic acid copolymers (Eudragit RS/RL), binder (hypromellose K100M), glidant (colloidal silicon dioxide), disintegrant (calcium phosphate dibasic dihydyrate), diluents (microcrystalline cellulose silicified, lactose monohydrate), and lubricants (magnesium stearate) were screened through a 20 mesh sieve, then dry blended in a planetary mixer. The resulting mixture was wet granulated with a binder solution comprising polyvinyl pyrolidone K30 and triethyl citrate, then dried and compressed into round tablets with a target hardness of 15 KP. The core tablets were heat cured at 35 C for two hours so that the methacrylic acid copolymers form a structure entangling the drug molecules. Eudragit RS/RL and hypromellose K100M both use diffusion as a controlled-release mechanism, but because they possess extremely different characteristics, together they formed a homogenous, intermeshing gel structure, which resulted in a multi-diffusion system that would release the active drug at zero order.

Preparation of coating solution. The coating suspension was prepared by dissolving methacrylic acid copolymers (Eudragit RS/RL PO at a ratio of 70:30) into a 1:1 mixture of ethanol and isopropyl alcohol. Triethyl citrate (TEC) was added as a plasticizer, and talc was added as an anti-tacking agent.

Coating of tablets. The tablets were coated in 15” pan coater (Compulab coater). The core tablets were prewarmed to 35 C, and the suspension was sprayed onto the tablets. The coated tablets were dried and cured at 35 C for two hours.

Evaluation of coated tablets. USP apparatus II (paddle method) (19) at 100 rpm with sequential dissolution (2 hours at pH 1.2 followed by 10 hours at pH 6.8) was used to obtain the drug release profile. A variety of mathematical models were evaluated to investigate the mode of drug-release kinetics.

Results and discussion
In formulations A and B, the core tablet contains a water-soluble excipient (lactose anhydrous) and gelling polymer (hypromellose). The cores also contain release-controlling polymers Eudragit RS and Eudragit RL along with TEC as a plasticizer. Due to this combination of release mechanisms, the drug will release from the core tablets via multi-step diffusion—erosion of the core tablets (due to water-soluble filler) and diffusion through the network of gelled hypromellose and Eudragit.

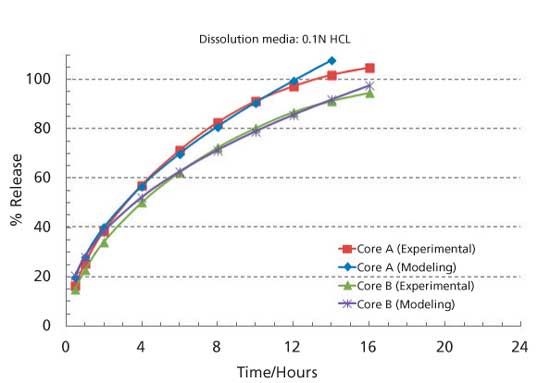
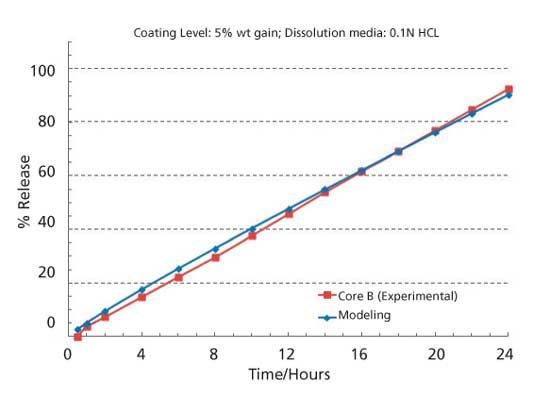
This diffusion can be explained using the Korsemeyer-Peppas model. This model was found to fit the drug-release profile after examining cores that indicate the combined effect of diffusion and erosion mechanisms. The diffusional exponent in formulation B was brought down to 0.45 by reducing HPMC in the formulation. This approach helped reduce the initial release burst (see Table II). The dissolution profiles of the core tablets (experimental and predicted using a mathematical model) are presented in Figure 1.

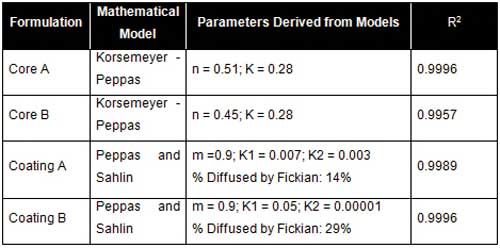
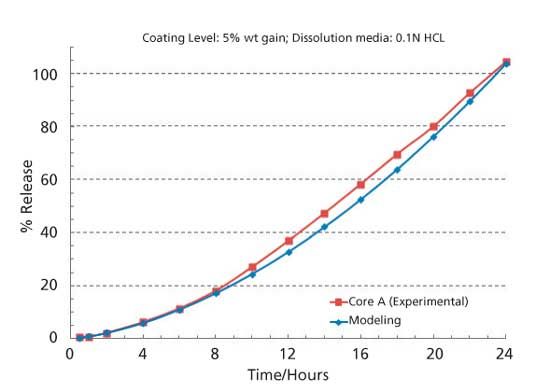
Because the dissolution profiles of the core tablets showed pseudo zero-order release, the core tablets in both formulations were further coated to 5% w/w using a combination of Eudragit RS and Eudragit RL. Due to this coating, the drug was released via ternary diffusion mechanism. This release was explained using the Sahlin-Peppas model, as it accounts for the combination of drug release through the hydrated matrix core and polymer- chain relaxation of the Eudragit coating. By optimizing these release-controlling polymers, reduction of the diffusional exponent of the tablet core to the Fickian diffusion was achieved (n = 0.45), and hence zero-order release was obtained. Table II shows the release parameters extracted through mathematical models for both formulations. The dissolution profiles of formulation A and formulation B are presented in Figure 2 and Figure 3, respectively.

Conclusion
Through the use of mathematical models, the number of experiments needed to optimize the desired drug-release profile was reduced. It was possible to use a semi-empirical approach to achieve zero-order release of a water-soluble drug through multi-step diffusion. Several mathematical models were investigated (zero/first order, Higuchi, Hixon-Crowell, Korsemeyer-Peppas, and Sahlin-Peppas) to identify the drug-release kinetics. Based on diffusional exponent and release kinetic constants, the mechanism of release indicated non-Fickian diffusion through Core A and Fickian diffusion through Core B. By reducing the gel network in Formulation B, the type II drug transport (zero-order) was achieved in Formulation B.

Acknowledgements
The author would like to thank Yogesh Chachare, Sateesh Sathigari, Ram Kasina, Anthony Qu, Raj Thota, and Senthil Kumar Manickam, all from Patheon.

References
1. P. Roshan, B. Uttam, T. Panna, J. Sci. Eng. Technol. 1 (5) 55-67 (2008).
2. M. Hemnani, et al. AJPTR 1 (4) 127-143 (2011).
3. T. Ghosh and A. Ghosh, J. Appl. Pharm. Sci. 1 (2) 38-49 (2011).
4. H. Kojima, et al., Eur. J. Pharm. Biopharm. 70 (2) 556-562 (2008).
5. M. Chavanpatil, et al.,  Int. J. of Pharm. 304 (1-2) 178-184 (2005).
6. C. Maderuelo, A. Zarzuelo, and J. Lanao, J. Control. Rel. 154 (1) 2-19 (2011).
7. D. Murthy and J. Ratna, Indian J. Pharm Sci. 70 (5) 555-564 (2008).
8. H. Yao, et al., Int. J. of Pharm. 364 (1) 21-26 (2008).
9. R. Langer, Science 249 (4976) 1527-1533 (1990).
10. K. Sungwon, et al., Eur.  J. of Pharm. and Biopharm. 71 (3) 420-430 (2009).
11. J. A. Champion, Y. K. Katare, and S. Mitragotri, J. Control. Rel. 121 (1) 3-9 (2007).
12. J. Peek, C. R. Middaugh, and C. Berkland, Adv. Drug Deliv. Rev. 60 (8) 915-928 (2008).
13. A.W Hixson and J.H. Crowell, Ind. Eng. Chem 23 (8) 923-931 (1931).
14. T. Higuchi, J. Pharm. Sci. 52 (12) 1145-1149 (1963).
15. R.W. Korsmeyer, et al., Int. J. Pharm. 15 (1) 25-35 (1983).
16. N.A. Peppas and J.J. Sahlin, Int. J. Pharm. 57 (2) 169-172 (1989).
17. R. Bodmeier and O. Paeratakul, Pharm. Res. 11 (6) 882-888 (1994).
18. Y. Huang, et al., Drug Dev. Ind. Pharm. 29 (1), 79-88 (2003).
19. J.L. Baxter, et al., Int J of Pharm. 292 (1-2) 17-28 (2005).

About the Author
Abhijit Gokhale is a senior formulation and process development scientist at Patheon Pharmaceuticals, [email protected], 2110 East Galbraith Road, Cincinnati USA 45237.