Statistical Solutions: Square Root of (N) + 1 Sampling Plan

Originating in the 1920s as a sampling scheme for agricultural regulatory inspectors, the square root (Sqrt) of the lot size (N) + 1 was semiformalized in an unpublished report by the Association of Official Agricultural Chemists (now Association of Analytical Chemists) in 1927 (1). Although it was used by nearly every company and by the US Food and Drug Administration, usually for incoming raw materials, most quality personnel questioned the validity of Sqrt(N) + 1 because it could not be found in statistical texts. So the question became, Is it valid and should companies and FDA continue to use and promote it?

Lynn Torbek

In February 1995, the US federal government cancelled the Mil Std 105E sampling plan. As a result, ANSI/ASQ Z1.4 became a popular attribute sampling approach (2). Z1.4 is used extensively for contract negotiations between companies and between companies and governments. In the pharmaceutical industry, Z1.4 is used for the attribute sampling of raw materials, in-process materials, and finished products.

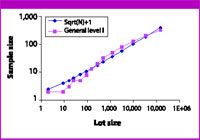
Figure 1: Sqrt(N) + 1 versus Z1.4 General Level I.

According to Z1.4, section 9.1: "A sampling plan indicates the number of units of product from each lot or batch which are to be inspected (sample size or series of sample sizes) and the criteria for determining the acceptability of the lot or batch (acceptance and rejection numbers)." Z1.4 is a statistically valid and statistically based attribute sampling plan with the following characteristics:

• The population, lot, or batch size N is considered
• The sample size n is specified and related to the lot size by Table I of Z1.4 (2)
• An accept number Ac is given (see Table II-A of Reference 2)

• A reject number Rc is given (see Table II-A of Reference 2)
• The operational characteristic (OC) curve was calculated statistically using known statistical distributions (see Tables X-A to X-S in Z1.4 of Reference 2)
• A key point on the OC curve, the acceptance quality limit (AQL), helps characterize the plan.

Consider lot size N = 1000 for an incoming raw material. For less critical materials, it is common to use General Inspection Level I in Z1.4, which leads to a sample size of n = 32. Using the criteria of accept on zero, Ac = 0, and reject on one, Re = 1, Table X-G shows that the AQL for the probability of acceptance P_a = 0.95 is 0.160%. That is, if the average level of nonconformance is equal to or less than 0.160%, then the lots in the series will be accepted 95% of the time.

Now consider the attribute sampling plan based on Sqrt (N) + 1, where Ac = 0 and Re = 1. It is also statistically valid and statistically based in that it has these statistical characteristics:

• The population, lot, or batch size N is considered
• The sample size n is specified and related to N by n = Sqrt (N) + 1
• An accept number is given Ac = 0
• A reject number is given Re = 1
• The OC curve is calculated statistically using known statistical distributions (3)
• The AQL helps characterize the sampling plan.

Consider again the raw material with N = 1000. The sample size is n = Sqrt (1000) + 1 = 32.6 or n = 33. Using Ac = 0, and Re = 1, software calculations show (3) that the AQL for P_a = 0.95 is 0.153%. For all practical purposes, the two plans are the same, given the slight difference in the sample size.

Sample sizes for Sqrt(N) + 1 are very close to the sample sizes in Z1.4 General Level I. For small lot sizes, Sqrt (N) + 1 samples are actually larger than Z1.4 General Level I (see Figure 1).

Both Z1.4 General Level I and Sqrt(N) + 1 are universally used in the industry for attribute sampling and inspection. Both are, as demonstrated, statistically based and statistically valid. We can have as much confidence in Sqrt(N) + 1 as we do of Z1.4 General Level I. Any comments or publications to the contrary are incorrect.

Sqrt(N) + 1 sampling is recommended in the FDA document, "CBER 03/01/92 Draft Points to Consider in the Manufacture of In Vitro Monoclonal Antibody." According to FDA's docket no. 91N-0466, section V3, " ... another protocol for testing representative lots (e.g., square root n + 1/yr, where 'n' equals the number of lots of product produced per year) may also be found to be satisfactory" (4).

Sqrt(N) sampling is recommended in FDA's Investigations Operations Manual, which states, " ... a general rule is to collect samples from the square root of the number of cases or shipping containers but not less than 12 or more than 36 subs in duplicate" (5).

Sqrt(N) + 1 is a statistically correct and valid sampling plan and can be used with the same care and caution as Z1.4 General Level I would be used.

References

1. H. Saranadasa, "The Square Root of N Plus One Sampling Rule: How Much Confidence Do We Have?" Pharm. Technol.27 (5), 50 (2003).

2. American Society for Quality (ASQ), ANSI/ASQ Z1.4-2008 (Milwaukee, WI, 2008).

3. W. Taylor, Guide to Acceptance Sampling (Taylor Enterprises, Inc, Lake Villa, IL, 1992).

4. FDA, CBER, "Draft Points to Consider in the Manufacture of In Vitro Monoclonal Antibodies," Docket 91N-0466, Mar. 1992.

5. FDA, Investigations Operations Manual, Subchapter 4.3: Collection Technique, section 4.3.7.2 Random Sampling, www.fda.gov/ICECI/Inspections/IOM/ucm122525.htm#4.3.7.2, accessed July 31, 2009.

Lynn Torbek is a statistician at Torbeck and Assoc., 2000 Dempster Plaza, Evanston, IL 60202, tel. 847.424.1314, Lynn@Torbeck.org, www.torbeck.org.