Ensuring virus safety for biological products

Ensuring the safety of viruses present in biologicals and products derived from blood is vital for manufacturing laboratories, control authorities and hospitals. Different clearance technologies, such as heat treatment, chemical disinfection radiation and filtration, are used to remove viruses from biologicals.¹

Viral inactivation parameters are estimated by the cytopathogen effect (CPE) from samples as a function of treatment time and concentration of the biological products. The formula is based on Poisson distribution (ln0.05=2.9957) and indicates that three virus particles (VPs) out of 100 can be present in the treated products at a 5% probability level. Negative CPE samples could even be detected. Inactivation parameters can also be calculated using this formula to obtain more information about the efficacity of virus removal. Further linear regression analysis with the inactivation parameter "logVP/total volume'' can be used to estimate the inactivation trend.^2–4

The aims of the study are to estimate the inactivation parameters from samples taken during 0–10 h of the virus clearance process and perform a linear regression analysis using statistical validation software.⁵

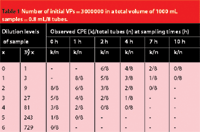
Table 1: Number of initial VPs=3000000 in a total volume of 1000 mL samples=0.8 mL/8 tubes.

Methods of evaluation

To evaluate the virus inactivation trend in biologicals used for therapeutic interventions two statistical principles were applied:

Poisson distribution. The distribution of the virus particles in the products follows Poisson distribution. This means the virus particle numbers can be estimated by the formula (ln0.05=2.9957). To obtain an estimate of VP/1.0 mL the (2.9957/8)*10 values are multiplied by the first negative (0/8) at higher dilution level values (at 3^x) of the product at each sampling time. Further inactivation parameters are derived from this basic formula.

Table 2: Formulae and estimation of inactivation parameters from the first 0/8 levels, where k=0.

Linear regression analysis. The parameters derived from the logVP/1000 mL estimates are used as dependent variables (y) on inactivation times (x=independent variable) to perform linear regression analysis.^3,4 A computer program calculates variance; linear regression function parameters, a retransformed estimate of y (the number of viral particles) from x; 95% confidence limits (CLs); and a retransformed estimate of x (the inactivating time in hours, where the estimated virus particle=1.0) from y with a 95% CL.⁵ It is important to control the fulfilment of the required validity assumptions and residuals.^6,7

Table 3: Interpretation of the formulæ.

Results

Table 1 describes the evaluation of the viral inactivation of biological products. The initial number of VPs is 3000000 in the total volume (1000 mL) of the product and indicates the sampling times (0–10 h) during the treatment and the 3^x dilution levels (1–729) with the related CPE positive or negative k/n (total tubes) values. Tables 2 and 3 describes the estimation of the inactivation parameters assuming Poisson distribution of the virus particles. Since ln0.05=2.9957 the survived VP is ≤3VP/0.8 mL sample at a CL of 95%. From the 2.9957/0.8 mL value the following inactivation parameters are calculated: (a) VP/0.8 mL, (b) VP/1.0 mL, (c) VP/1000 mL, (d): logVP/1000 mL, (e) y^{^}_log, (f) reduction rate, (g) 1/reduction rate in %.

Table 4: Analysis of variance (Anova) for linear regression of y (log c) on x (0–10 h).

Tables 4 and 5 present the result of the linear regression analysis of y (d:logVP/1000 mL) dependent variable on x (process time: 0–10 h) independent variable. Statistics of the Regranal–Anovar and regression parameters are given with the standard error (SE) and 95% a confidence interval (CI). The Null Hypothesis (H0) is rejected given significant regression: P=0.00042 (i.e., there is a virus-removal trend). As there is no significant deviation from linearity the regression equation (y_log=a+bx) can be used for extrapolation.

Table 5: Linear regression function paramaters.

Tables 6 and 7 show that the predicted values for 10 h, 20 h and 30 h treatment are 4542.1, 10.3 and 0.02 VPs in 1000 mL of product respectively. The estimated clearance time to obtain one VP is 23.83 h. Table 8 illustrates that all statistical validity criteria on assumptions and residuals are fulfilled.

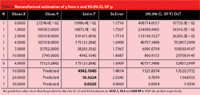
Table 6: Retransformed estimation of y from x and 95.0% CL OF y.

Discussion

Viral safety of biological products derived from blood or biological fluids of human or animals is a fundamental prerequisite for using them as therapeutics. The viral inactivation can be tested by statistical evaluation of the CPE trend from the clearance treated samples.

Table 7: Retransformed estimation of x from y and 95.0% CL of x:

This article describes the estimation of the viral inactivation parameters and the linear regression analysis of the virus decreasing trend calculated from the samples of the clearance treated biological product. The results provide information on the length of treatment needed for obtaining a 0/8 (k/n) value in the concentration level (3^x) of the products; the inactivation parameters estimated from the ln0.05=2.9957 value; how to calculate the prediction for survival number and rates of VP from the regression equation; and the probability level of the validity of the viral inactivation of biological product by using a statistical validating regression model.^6,7

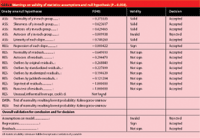
Table 8: Warnings on validity of statistics: assumptions and null hypothesis (P=0.050).

The statistical model described ensures the virus safety evaluation required by regulatory authorities can be provided by companies that produce biological products.^8–11

Laszlo Lugosi is Professor of preventive medicine. He was head researcher of the BCG Laboratory at the Bela Johan National Institute of Public Health, Budapest (Hungary). Currently, he is the charter owner and medical director of Medwinstat International Ltd, studying medical validity of models in life and health sciences.

Imre Molnar is the IT director of Medwinstat International Ltd. Formerly, he was a software engineer at the Medical University of Budapest (Hungary).

References

1. G. Kern and M. Krishnan, Pharm. Technol. Eur., 18(12), 29–36 (2006).

2. J.M. Huraux, J.C. Nicolas and H. Agut (Eds.), Virologie (Flammarion, Paris, France, 1991).

3. G. Bouveno and M. Vray (Eds), Essai Clinique: Théorie, Pratique et Critique, 3rd Edition (Flammarion, Paris, France, 1999).

4. P. Armitage, G. Berry and J.N.S. Matthews (Eds), Statistical Methods in Medical Research, Fourth Edition (Blackwell Science, Oxford, UK, 2002).

5. MEDwInSTAT Statistically Validating Software for Life and Health Sciences, Version w5.00, MEDwInSTAT Software International Ltd, Budapest, Hungary, 2004. http://members.chello.hu/medwinstat.software

6. D.J. Finney (Ed), Statistical Methods in Biological Assay, 3rd Edition (Griffin, London, 1978).

7. N.K. Jerne. and E.C. Wood, Biometrics, 5(4), 273–299 (1949).

8. Committee for Proprietary Medicinal Products: Ad Hoc Working Party on Biotechnology/Pharmacy and Working Party on Safety Medicines, Biologicals, 19(3), 247–251 (1991).

9. FDA, "Points to consider in the manufacture and testing of monoclonal antibody products for human use." www.fda.gov/cber/gdlns/ptc_mab.pdf

10. Millipore, "Ensuring Compliance: Regulatory Guidance for Virus clearance Validation," www.millipore.com/viresolve

11. EMEA, "Guideline on Virus Safety Evaluation of Biotechnological Investigational Medicinal Products." www.emea.europa.eu/pdfs/human/bwp/39849805en.pdf