Effects of Initial Average Particle Size on Tableting: Evaluating Predictive Tools for Crystal Engineers and Formulators

The indentation-size effect forms the basis of the relationships connecting average particle size (r^0.5 )with densification, tensile strength, and hardness of one-component tableting systems. Those structure–property relationships also have been verified by quantitative data presented in the literature, and they may serve as a predictive tool for crystal engineers and formulators to design particles with the right average size for tableting at the beginning of product development. In general, densification, tensile strength, and hardness increase as the average particle size decreases, and a significant amount of compaction pressure is required for densification to occur. These effects present a potential problem when compacting particles that are very small.

Producing pharmaceutical tablets involves combining the active pharmaceutical ingredient (API) and excipients. When a high API drug-load is desired, selection or manipulation of the excipients or process alone may not be sufficient to compact the tablet. The average particle size of an API influences compactability, but how particle size affects compactability remains unclear. Correlations between average particle size and tablet properties such as densification, tensile strength, and hardness are important because those correlations can generate predictions, which facilitate the selection and design of appropriately sized particles.

Loose powders have a very low initial relative density ρ₀. Relative density ρ_r is defined as the density of the compact (mass of a compact divided by its volume) divided by the true density, which is determined by helium pycnometry (1). The cold isostatic compaction of powders by the application of macroscopic compaction pressure P (i.e., uniaxial or hydrostatic pressure) is divided into four irreversible densification stages as P increases (2, 3). Those stages are particle rearrangement, local plastic deformation at the interparticle contacts, elastic and plastic deformation of the particles, and bulk deformation of the compact by fragmentation once it reaches a state of closed porosity.

In stage one, weak interparticle attractive forces counteract gravitational forces and produce dangling bonds (2), which form low-density aggregates of particles. In stage two, mechanical compaction begins to take place. The fractional packing density ρ0 increases from 0.35 to 0.74 (2). At this stage, each particle averages 4.75 contacts and begins to experience "jamming." The onset of general particle deformation starts around ρ₀ = 0.85 (2). Beyond that threshold, only the application of very high pressure produces further densification in stages three and four.

The densification phenomenon usually is described as the first-order kinetics of applied macroscopic pressure P by the Heckel equation (4):

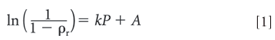
in which k and A are constants obtained from the slope and intercept of the plot ln (1/(1– ρ_r)) versus P, respectively. Two important mechanical properties of tablets: tensile strength σ (1) and hardness H (5) further relate to the relative density (ρ_r ) by:

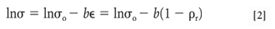
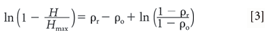
in which є, b, and lnσ₀ in Equation 2 are porosity and constants obtained from the slope and intercept of the plot ln σversus (1 – ρ_r), respectively. H_max and ρ₀ in Equation 3 are, respectively, the maximum hardness at zero porosity and the initial relative density needed for minimal shear strength of the powder bulk. When one substitutes Equation 1 into Equation 2 and Equation 3, tensile strength and hardness become functions of macroscopic compaction pressure P.

Interestingly, empirical studies suggest that densification (Equation 1), tensile strength (Equation 2), and hardness (Equation 3) of tablets are not affected only by packing structures є and ρ_r and pressure, but that those properties are also affected by initial average particle size. (1, 6, 7). Although the effects of initial average particle size are implicitly included in the material characteristic constants of k, A, b, and ln σ₀ in Equation 1 and Equation 2, this article presents three explicit relationships that link average particle radius r with densification, tensile strength, and hardness during stage two of densification. At a minimum, those relationships require the establishment of only two data points and serve as predictive tools to engineer the appropriate average particle size in direct tableting.

Modeling

Helle et al. derived a simple relationship that assumes all powders are monosized spheres and the number of contacts per particle resembles microindentation and increases during stage-two compaction (2, 8):

in which H is the indentation hardness of the particle material. Helle et al. also derived a semi-empirical relationship between the contact size L and the average particle radius r for a given level of density that is reached ρ_r during stage-two densification (2, 8):

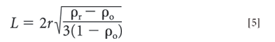
Effects of initial average particle size on powder compaction that involve interparticle contacts is conceptualized as the indentation-size effect (ISE) among particles. Hardness increases with decreasing indentation size. The phenomenon of ISE is explained by the strain-gradient plasticity. Under the influence of strain-gradient plasticity, plastic strengthening becomes important when a material in question is plastically deformed in very small volumes (e.g., at the tips of cracks or microindentations). The semi-empirical ISE equation has the form (2, 9):

in which H_o is the large-size hardness lower limit, L₀ is a material parameter, and L is the imprint size.

Particle-size dependence of densification. If one combines Equations 4, 5, and 6 and assumes L << L₀ , the average particle size r dependence of densification becomes:

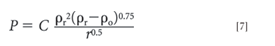
in which C is a constant:

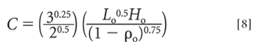
Particle-size dependence of tensile strength. By substituting Equation 2 into Equation 7, the average particle size r dependence of tensile strength becomes:

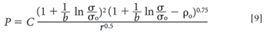
Particle-size dependence of hardness. By linking Equation 3 with Equation 7 and assuming that H/H_max << 1 and that ρr–ρ < 1–ρ₀, the dependence of hardness on the average particle size r becomes:

Results and discussion

To test the validity of Equations 7, 9, and 10, those equations were plotted against some useful quantitative data from the literature using Microsoft Excel (Microsoft Corp., Redmond, WA). The cold compaction data of pure paracetamol powders (P, ln (1/(1 – ρr)), and 2r) were taken from Figure 3 of Garekani et al. (6) and were replotted in Figure 1 by using Equation 7. The raw data is shown in Table I. We assumed ρ₀ to be less than ρ_r and set ρ₀ to 0.35. Equation 7 was also extended to 80% drug-load tablets, and the compaction data of P, ρ_r, and 2r from Figure 9 of Sprockel et al. (10) were replotted in Figure 2. The raw data are summarized in Table II. In Figure 3, the tensile strength data of pure L-lysine monohydrochloride dihydrate tablets such as P, σ σ₀, and 2r from Figure 2 and Table II of Sun et al. (1) were replotted by using Equation 9. ρ_o was also set to 0.35. The raw data is shown in Table III. Crushing-strength data of pure α-lactose monohydrate powders of P, H, and 2r from Figure 6 of Vromans et al. (7) were replotted as hardness in Figure 4, which has ρ_o = 0.35 and H_max = 40. The raw data are compiled in Table IV.

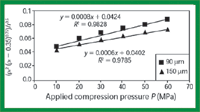
Figure 1: Effects of average particle size on densification of pure paracetamol powders.

Apparently, linearity in Figures 1, 3, and 4 seems to verify the validity of Equations 7, 9, and 10 and suggests that the initial particle-size effects of r^0.5 on densification, tensile strength, and hardness, which are different from the average number of interparticle contacts and the contact force, for they scale with r² , the square of particle size (2, 11). There are two caveats, however. First, the particle size r that we used is the average of each particle size fraction by sieving. For example, r =105 μm in Figure 1 actually is the average of the particle fraction of 105–210 μm in the published article (6). Second, strictly speaking, hardness is the resistance of a material to penetration. By assuming pure α-lactose monohydrate powders are ductile, however, we were able to test their parallel crushing strength using Equation 10. The nonlinearity in Figure 2 mainly results from the measurement fluctuations of the original compaction data (Figure 9) of Sprockel et al. (10).

Table 1

Different slopes and different y-intercepts of straight lines in each figure could imply that factors other than average particle size also have profound effects on densification, tensile strength, and hardness. These factors include and are not limited to morphology (12), water content in the particle (12), crystallinity (13), lattice defects (13), impurities (13), and cracks (14), which are all strongly process dependent. Isolation of the effects of average particle size from other factors requires a consistent processing route, powders of various sizes sorted by careful sieving, and plotting a curve for each specific average particle size instead of a curve for each compaction pressure.

Table II

Equations 7, 9, and 10 further suggest that at a constant compaction pressure P, densification ρ_r, hardness H, and tensile strength σ may increase as the average particle size r decreases. Apparently, small average particle size favors compactability and tablet strength. The inverse proportionality of compaction pressure P and the initial average particle size r, however, indicate that strong difficulties may be encountered in the cold compaction if powders are made too fine (15). That is probably a result of the increase in the number of grain boundaries, which resist plastic deformation (16). Large initial particles that are brittle also may resist compaction when they are fragmented into small pieces during densification.

Table III

Future work requires systematic experiments and direct measurements in powder compaction. Further refinements in the modeling of the effects of initial average particle size on tableting should include the elastic flattening of the contacts (17). Equations 7, 9, and 10 can be used to link Hiestand indices (18) and the effect of average particle size.

Table IV

The latest developments in crystal engineering and particle nanotechnology, which both allow for the design and control of particle size at the submicron and nanosize levels, reinforce the importance of studies that describe the effects of initial average particle size on tableting. Structure–property relationships that relate the average particle size with densification, tensile strength, and hardness on tableting serve as predictive tools for crystal engineers and formulators to choose and design particles of the appropriate size for tableting, thereby decreasing the development time for API and tablet production.

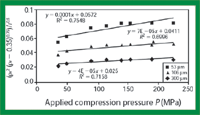
Figure 2: Effects of average particle size on densification of 80% ([(2S)-2-mercapto-1-oxo-4-(3,4,4-trimethyl-2,5-dioxo-1-imidazolidinyl)butyl]-L-leucyl-N,3-dimethyl-L-valinamide), 19.5% microcrystalline cellulose and 0.5% magnesium stearate.

Conclusion

Quantitative data from the literature show strong relationships among average particle size r^0.5 with powder densification, tensile strength, and hardness. The inverse proportionality between compaction pressure and initial average particle size indicates potential difficulties in compaction if particles are made too fine. The linearity of those relationships allows a rapid evaluation of tablet performance for any powder with a minimum of two data points. Upon further refinements and more powder compaction experiments, structure–property relationships similar to Equations 7, 9, and 10 could be useful predictive tools for crystal engineers and formulators involved in tablet manufacture.

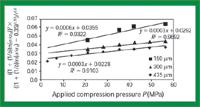
Figure 3: Effects of average particle size on tensile strength of pure L-lysine monohydrochloride dihydrate powders.

Acknowledgments

This work was supported by a research grant from the National Science Council of Taiwan, Republic of China (NSC 93-2119-M-008-003 and NSC 94-2119-M-008-001).

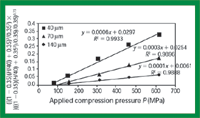
Figure 4: Effects of average particle size on hardness (crushing strength) of pure a-lactose monohydrate powders.

Tu Lee PhD,* is an assistant professor at the Department of Chemical and Materials Engineering and at the Institute of Materials Science and Engineering, at the National Central University, Chung-Li, Taoyuan, Taiwan 320, Republic of China, tel. +886 3 422 7151, ext 34204, fax +886 3 425 2296, tulee@cc.ncu.edu.twChung Shin Kuo is a graduate student, Department of Chemical and Materials Engineering, National Central University.

*To whom all correspondence should be adressed.

References

1. C. Sun and D.J.W. Grant, "Effects of Initial Particle Size on the Tableting Properties of L-Lysine Monohydrochloride Dihydrate Powder," Int. J. Pharm. 215 (1–2), 221–228 (2001).

2. J. Gil Sevillano, "Size Effects in Powder Compaction," J. Mat. Res. 16 (5), 1238–1240 (2001).

3. C. Sun and D.J.W. Grant, "Influence of Elastic Deformation of Particles on Heckel Analysis," Pharm. Dev. Technol. 6 (2), 193–200 (2001).

4. R.W. Heckel, "Density–Pressure Relationships in Powder Compaction," Trans. Metal. Soc. AIME. 221, 671–675 (1961).

5. M. Kuentz and H. Leuenberger, "A New Model for the Hardness of a Compacted Particle System, Applied to Tablets of Pharmaceutical Polymers," Powder Technol.111 (1-2), 145–153 (2000).

6. H.A. Garekani et al., "Effect of Compression Force, Compression Speed, and Particle Size on the Compression Properties of Paracetamol," Drug Dev. Ind. Pharm. 27 (9), 935–942 (2001).

7. H. Vromans et al., "Studies on Tableting Properties of Lactose: The Effect of Initial Particle Size on Binding Properties and Dehydration Characteristics of (α–Lactose Monohydrate," Drug Dev. Ind. Pharm. 12 (11–13), 1715–1730 (1986).

8. A.S. Helle, K.E. Easterling, and M.F. Ashby, "Hot-Isostatic Pressing Diagrams: New Developments," Acta Metall. 33 (12), 2163–2174 (1985).

9. W.D. Nix and H. Gao, "Indentation Size Effects in Crystalline Materials: A Law for Strain-Gradient Plasticity," J. Mech. Phys. Solids 46 (3), 411–425 (1998).

10. O.L. Sprockel et al., "Control of Compactability through Crystalliztion in Tablet Formation," (Bristol-Myers Squibb Company, USA) PCT Int. Appl. WO 02088664 A2, 2002.

11. N. Ouchiyama and T. Tanaka, "Estimation of the Average Number of Contacts between Randomly Mixed Solid Particles," Ind. Eng. Chem. Fundam.19 (4), 338–340 (1980).

12. M. Radeka et al., "Compaction Mechanism as the Function of Atomized Powder Particle Size," Ceramics Int. 21 (4), 249–255 (1995).

13. R. H�auch, "Modification of Starting Materials to Improve Tableting Properties," Pharm. Ind. 45 (4), 435–440 (1983).

14. T. S. Srivatsan et al., "An Investigation of the Influence of Powder Particle Size on Microstructure and Hardness of Bulk Samples of Tungsten Carbide," Powder Technol. 122 (1), 54–60 (2002).

15. E. Merisko-Liversidge, G.G. Liversidge, and E.R. Cooper, "Nanosizing: A Formulation Approach for Poorly Water-Soluble Compounds," Eur. J. Pharm. Sci.18 (2), 113–120 (2003).

16. A. Hasnaoui, H. Van Swygenhoven, and P. M. Derlet, "Dimples on Nanocrystalline Fracture Surfaces as Evidence for Shear Plane Formation," Science300 (5625), 1550–1552 (2003).

17. Mechanical Behavior of Materials, F.A. McClintock and A.S. Argon, Eds. (Addison-Wesley, Reading, MA, 1966).

18. H.E.N. Hiestand and D.P. Smith, "Indices of Tableting Performance," Powder Technol. 38 (2), 145–159 (1984).