Dielectric Spectroscopy: Choosing the Right Approach

The US Food and Drug Administration's process analytical technology (PAT) initiative reflects the need for improved quality control in the pharmaceutical industry. As the complexity of pharmaceutical products increases, quality control becomes more costly and difficult. New analytical instrumentation should be developed to address the quality control problems associated with more complex pharmaceutical products. Dielectric spectroscopy, one of many process analytical technologies, has been used for several decades. As any other technology, it is continuously evolving. New instrumentation, algorithms, and materials broaden the applicability and capabilities of this method. Selecting the optimal architecture for a sensing system intended for each specific application requires a good understanding of main principles and engineering trade-offs common for this field. This article is written primarily for field practitioners who need to make a judicious choice of the best measurement technique for their application. Main principles and design features of dielectric sensors are discussed in the framework of pharmaceutical applications.

The range of potential applications of dielectric spectroscopy is quite broad. Virtually any physical process change leads to changes in dielectric properties of samples. Process variability is a primary concern for the pharmaceutical industry (1). Exposure to mechanical and thermal stress can cause a change in the physical properties of pharmaceuticals. Such variations are important to control because physical properties generally determine the efficacy of the drug. For instance, tablet coatings control the rate of drug delivery within the body of a patient (2–5) and influence the bioavailability of the drug. In addition, coatings protect the active ingredient from chemically harsh environments in the body (6). Similarly, API content in a given sample determines the potency of the drug. The drying process of pharmaceuticals is critical because 70% of global granulated pharmaceutical product is made using wet granulation (7). In wet granulation, liquid binding gels are used to facilitate bonding between active ingredients. At this stage, it is important to measure moisture because specific moisture levels are required for the formation of the correct-size granules. Further, in high-shear wet granulation methods, incorrect moisture levels can lead to a process-induced transformation (PIT) (8). In these transformations, the properties of the active ingredients can change, resulting in reduced efficacy.

Overview of sensing methods

Process analytical technologies. A number of sensing technologies can be used to detect physical properties of pharmaceuticals. Most commonly used technologies include near infrared spectroscopy (NIR) and Raman spectroscopy. Digital imaging methods, optical methods, and dielectric spectroscopy also are used to measure various physical properties.

NIR spectroscopy is widely used to measure API content, distribution of contaminants, moisture content, and polymorphism determination (9). Similarly, Raman spectroscopy has demonstrated success in measuring pharmaceutical reaction times, polymorphism, and differences between solid-state forms (8).

Digital imaging methods are used to monitor particle size in pharmaceutical powders (10). Optical methods have been used for inspection of tablet coatings (6), measurement of constituent concentration of pharmaceutical powder mixtures (11), and quantitative analyses of ascorbic acid in pharmaceuticals (11, 12). For example, laser induced breakdown spectroscopy (LIBS) is used to measure coating thickness of tablets (13).

Previous work using dielectric spectroscopy. Dielectric spectroscopy is a promising method to study physical properties of pharmaceutical solutions, colloids, microcapsules, gels, and emulsions. Craig provides a thorough review of these applications (14), which are summarized in this section.

Pharmaceutical solutions are important because the solubility of a drug in the solvent directly influences its rate of disassociation in the body, which in turn impacts bioavailability. Dielectric properties such as the static dielectric constant of solutions has been used to study the solubility of cosolvent systems, reaction rates of pharmaceutical solutions, and drug stability (14).

Colloids are two-phase systems that consist of particles suspended in a medium. Each particle in a colloidal solution has an electrical layer surrounding it that determines the stability of the system by affecting the aggregation or disassociation of colloidal particles. As a result of these forces, dielectric response of colloidal solutions provides information about particle size in the colloidal solutions. For example, a study conducted by Paul and Vogey suggested an inverse-square dependency between particle radius and dielectric relaxation frequency of the medium (15). This relationship is illustrated in the following equation:

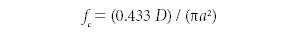
in which D is the diffusion coefficient of counterions with a particle radius of a, and f_c is the loss peak frequency. The inverse-square relationship between relaxation frequency and particle radius was confirmed in a subsequent study (16).

Microcapsules have attracted attention as an improved drug delivery method. Coating thickness and uniformity of microspheres directly influences the rate of dissolution in the body, making it very important to study its structural properties. Previous studies show methods to relate the relative dielectric permittivity and thickness of the capsule wall (17). Additional information provided by dielectric measurements includes volume fraction of microspheres in solution and the dielectric properties of the interior core of microcapsules (14).

Dielectric spectroscopy has shown promise for the measurement of structural and interfacial properties of gels. Gels are complex systems with a number of dielectric relaxation processes that can be related to unique physical characteristics. For example, previous studies showed the response of aqueous gels containing cetosteryl alchohol and cetrimide show four distinct bulk processes (18), These responses were attributed to the relaxation of water, ions in bilayers formed resulting from the presence of cetosteryl alchohol, and migration of ions in channels between these bilayers. Therefore, dielectric spectroscopy can be used to monitor multiple physical properties of complex structures. Dielectric spectroscopy was used to study the likelihood of high surface contact between nasal mucosa and pharmaceutical gels by measuring conductivity as pharmaceutical drug ions transported from the gel into the nasal mucous membrane (19).

Emulsions and microemulsions have attracted interest as an effective drug delivery method for drugs that are insoluble in water. Dielectric spectroscopy has been used to classify the types of emulsions. For example, dielectric properties are used to distinguish between water-in-oil and oil-in-water emulsions (14).

The remainder of this article discusses key issues in dielectric sensor selection. Measurements of tablet coating thickness are used to illustrate methods of dielectric data processing.

Dielectric-sensing selection guide

There are two major properties of dielectric spectroscopy that are typically varied to suit the desired application: the spatial distribution of the interrogation field and the interrogation frequency range. Changes to the field's spatial distribution of the field used in dielectric spectroscopy enables measurements in the following modes: bulk, surface/low-penetration, multiple penetration depth, imaging, and a combination of these. The frequency range can be optimized for a particular material of interest and can vary from 10^–6 to 10¹¹ Hz, although a frequency range of only a few orders of magnitude is typically used.

Based on the measurement constraints for the application, the spatial distribution of the field and the frequency range are ascertained by answering a series of questions, which are discussed in the following sections.

Through-field or fringing-field? In dielectric spectroscopy, the attenuation and phase shift of an electric field at specific frequencies are measured as it passes through a material of interest. Attempts are typically made to either linearize the field or to limit the penetration of the field to a known depth into the material of interest. In general, through-field implies a "pitch and catch" configuration in which one electrode delivers an excitation signal and another electrode measures the resulting signal after passing in an approximately linear fashion through the material of interest such as in a parallel plate capacitor configuration (see Figure 1a). In the case of fringing-field sensing, one electrode delivers an excitation signal, and a second electrode is placed such that the original signal passes through the material of interest in a nonlinear fashion. The most common fringing field configuration is one in which the excitation and sensing electrodes are mounted in a coplanar fashion on the same substrate (see Figure 1b).

Figure 1 (ALL FIGURES ARE COURTESY OF THE AUTHORS.)

Through-field or fringing-field sensing techniques are typically chosen on the basis of the following: only one sided access to sample is available, significant variations in thickness exist, and the sensor must operate in an electrically noisy environment. To determine whether through-field or fringing-field is desired for an application, access to the material of interest must first be determined. If two-sided access is possible and the sample thickness does not vary significantly, through-field is recommended. If only one-sided access is available or if the sample thickness varies significantly, then a fringing-field measurement is recommended. To obtain an effective measurement with either sensor type, a basic knowledge of the system is important. The nominal thickness of the sample should be known as well as the primary sample constituents and the likelihood of metallic impurities.

A common electrode arrangement for through field imaging is seen in electrical tomography (ET). In this method, the electrodes are distributed around the edges of a cylindrical surface. Individual electrodes are electrically excited and the current or voltage induced on other electrodes is measured. The measured values are used to reconstruct distribution of physical properties in the material by solving the inverse problem. ET is used for biomedical, geophysics, and industrial process control applications (20–22). Compared with other imaging methods such as X-ray tomography and magnetic resonance imaging, ET is fast, noninvasive, and inexpensive in instrumentation. Although ET is useful for measurements in cylindrical surfaces, it is not viable for scenarios in which one-sided measurements are required.

In the case of fringing-field measurements, a common example is referred to as a fringing electric field (FEF) sensor. An FEF sensor can perform three distinct measurement functions depending on how it is configured: defined penetration depth measurements, multiple penetration depth measurements, and one-sided measurements.

In the case of the FEF sensor, the field is nonlinear and often has smaller signal strength than that of the through-field configuration. However, the field is concentrated much closer to the electrodes and therefore has a defined penetration depth. Penetration depth depends primarily upon how close the electrodes are to each other.

Dielectric spectroscopy can be performed with FEF sensors such that only the layer closest to the electrodes is examined. By altering the geometry of the electrodes or by selecting the electrodes that are being excited and those being measured, the penetration of the field can be progressively moved further into the material. By using multiple channel devices, various channels can be used to measure dielectric properties of the material at different penetration depths (see Figure 2).

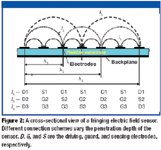
Figure 2 (ALL FIGURES ARE COURTESY OF THE AUTHORS.)

FEF sensors measure the material of interest from a single side. They are formed by a coplanar distribution of electrodes. Figure 2 shows the cross-sectional view of an FEF sensor. The spatial wavelength of the sensor, which is defined as the distance between centers of electrodes with the same polarity, can be varied depending on the excitation of the electrodes. The penetration depth of the sensor is mainly dependant on the spatial wavelength and is approximated as one-third the spatial wavelength. Conceptually, the penetration depth is an effective measure of the rate at which the electric field decays away from the sensor surface. Variations in penetration depth also can be obtained from the various electrode excitation patterns to provide the sensor with access to different layers of the test specimen. In Figure 2, the parameters l₁, l₂, and l₃ represent three different ways of connecting the electrodes. D represents the driving electrodes; an AC voltage signal is applied to these. S represents the sensing electrodes. At these electrodes, current–voltage measurements are made. G represents the guard electrodes. In the table below the figure, the cross-sectional view of the sensor in Figure 2, each row corresponds to one of the three connection schemes; each column corresponds to the types of connections for the electrode directly above the column used in the different connection schemes. For l₁, every other electrode is driven and the undriven electrodes are treated as sense electrodes. For l₂ and l₃ several undriven fingers are chosen as guard electrodes and only the current–voltage at the sensing electrodes is measured. The guard electrodes are either connected to ground or kept at the same voltage potential as the sensing electrodes by using unity-gain buffer amplifiers. The spatial wavelength of the sensor is increased (λ₃ > λ₂ > λ₁) by using various connection schemes. A ground plane below the electrode surface eliminates the effects of underlying material and stray noise in the medium. Major advantages of this technology include one-sided access to materials under test, profiling and imaging capabilities, and model-based signal analysis.

Direct sensing or preconcentrators? There are many cases in which gas or liquid analytes must be sensed in small concentrations close to, or below, the detection threshold of a dielectric spectroscopy measurement device. Direct sensing is simpler than preconcentration; however, direct sensing is not always possible. Preconcentration should be performed in the following cases:

• When the analyte is at a concentration at or below the measurement threshold

• To select an analyte of interest from a mixture of gases or liquids.

The advantage of direct sensing is that it is does not require the modification of the measurement setup for a particular analyte. However, because the preconcentration process acts as an amplifier, the signal-to-noise ratio may better than the direct-sensing case.

To give a feel for typical magnitude of the effect, consider polyimide used for humidity measurements. Polyimide is hydrophilic and can absorb water for as much as 3% of its dry weight (23, 24). Because of the high dielectric permittivity of water (ε_r ≈ 80) in comparison with the dielectric permittivity of dry polyimide (ε_r ≈3), even relatively small changes in humidity may increase the dielectric permittivity of polyimide by as much as 30% (25, 26).

Dielectric spectroscopy has been used with gaseous analytes as well. With gases, the preconcentrator material will typically form a compound with the gas and may require heating of the substrate to increase the analyte absorption. Metal compounds are used as preconcentrators for gaseous analytes (27, 28). For example, use of Ni-PC (tetra-t-butyl phthalocyaninatonickelCII) coating as a preconcentrator improves the sensitivity and signal-to-noise ratio of interdigital sensors toward ethanol fumes (27). Preconcentrators improve the selectivity of the sensor for a particular analyte because it is possible to select a preconcentrator with an affinity for only the analyte of interest. Hagen et al., provided examples of highly specific preconcentrators in which the addition of a chromium layer between interdigitated gold electrodes and a zeolite Pt-Na-Y membrane resulted in highly selective sensors for hydrocarbon vapors such as butane (29).

Benefits associated with preconcentrators include improved sensitivity and versatility with various analytes. However, with the use of preconcentrators, sensor response is subject to hysterisis because the sensitive layer cannot reach immediate equilibrium with the environment.

Single frequency or spectroscopy? Relaxation processes in dielectric spectroscopy are very similar to relaxation processes in the optical regime. However, the interrogation frequencies used in dielectric spectroscopy are lower than optical frequencies, so this technique studies molecular interactions such as polymer reconfiguration within a matrix, percolation processes, and moisture diffusion. A major advantage of dielectric spectroscopy is that it can be performed over a wide band of measurement frequencies. The lowest boundary for frequency in existing dielectric spectroscopy is around 1 μHz, and the highest is in THz range. It is rarely practical to go to such extremes; most practical industrial measurements are accomplished in the range from 1 Hz to 100 MHz. In the PAT context, time available for inline measurements can be a limitation for a spectroscopic approach. This can be overcome by selecting frequencies at which the response to properties of interest provides adequate sample resolution. In this section, experimental data is analyzed using a spectroscopic approach, and, after some postprocessing, a single frequency optimum for measurements is selected.

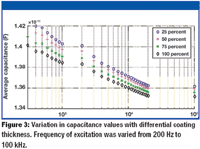
Figure 3 (ALL FIGURES ARE COURTESY OF THE AUTHORS.)

A spectroscopic approach involves a detailed study of various processes that occur at different frequencies. Application of an oscillating electromagnetic field to samples results in either ionic charge transport, at low frequencies, or dielectric loss caused by energy dissipation at higher frequencies. These phenomena are dependant on physical properties of the dielectrics such as viscosity, density, and moisture content. Therefore, the response of a sample over a range of excitation frequencies provides information pertaining to the physical structure and composition. Figure 3, Figure 4, and Figure 5 provide an example of spectroscopic measurement. The impedance spectra of tablets with 25, 50, 75, and 100% coatings were measured. Figure 3 shows a monotonic trend in capacitance with coating levels. In Figure 3, the smooth trend in the frequency spectrum is a characteristic of dielectric spectroscopy measurements. Unlike other technologies such as NIR, measurement peaks resulting from relaxation times are visible with wide frequency bands spanning several decades. Moreover, the separation between samples at lower frequencies is greater because at lower frequencies the system is not as dissipative. It should be noted that the discontinuity in measurement from 1 kHz to 2 kHz is a result of the switching of internal circuitry in the measurement instrumentation and does not reflect dielectric behavior. Figure 4 shows the conductance spectrum for the four samples. At lower frequencies, the conductance measurements are too low for instrumentation. At low frequencies, conductance is a result of charge transport processes. Figure 5 shows the variation in phase spectrum with various coating thicknesses.

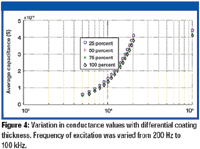
Figure 4 (ALL FIGURES ARE COURTESY OF THE AUTHORS.)

All chemicals that constitute a pharmaceutical sample influence the output of the sensor. Hence it is important to isolate the effect of the analyte under study on the sensor output from those from other chemicals. This is a complex problem that requires extraction and isolation of the contributions of each constituent based on a weighted convolution of their known relaxation processes and that observed in the mixture.

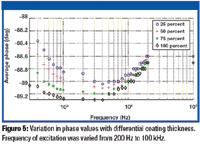
Figure 5 (ALL FIGURES ARE COURTESY OF THE AUTHORS.)

A simple solution is to create a calibration-based estimation model that uses the spectroscopic data from a narrow frequency range and makes approximations on the type of relaxations, transport mechanism, and chemical processes being observed (30–32). This method is most commonly used in situations in which the variations in the composition are in a narrow range and speed is of more importance than accuracy.

In a rigorous and exhaustive approach to model multianalyte concentration variations, the authors consider the material under test to be a homogeneous mixture of the analyte, other constituent chemicals, and air. By varying the proportion of this mixture, we can vary the relative concentrations of the materials. The effect of variation in proportion of the mixture on its dielectric property is nonlinear and requires considerable effort to model. The static (frequency-independent) relative dielectric permittivity for an isotropic ensemble of N equivalent molecules each having a dipole moment, μ, and contained in a spherical volume, V, is given by the following equation:

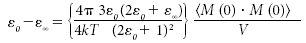
in which M(0) is the instantaneous dipole moment of the macroscopic sphere at the arbitrary time t=0. The equilibrium value of the scalar product ‹M(0)M(0)› taken over all the complexions of the ensemble will be independent of time for a stationary thermodynamic system. Considering a system of N constituents in the mixture of volume V

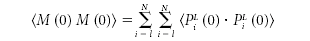
in which P_i^L (0) is the instantaneous liquid dipole moment of the ith constituent of the mixture. Detailed analysis of mixtures and their dielectric properties can be found in References 33–37.

Obtaining wideband spectroscopic measurements can be lengthy process because the time available for inline measurements is limited. Alternatives for such measurements are single-frequency or selected-frequency excitations. In this case, the response of a sample at a single or selected frequency can be used to monitor changes in physical properties. It should be noted that the observation of dielectric spectra at just selected frequencies could result in measurement artifacts being misinterpreted as dielectric phenomena; therefore, such an approach is possible if there is some prior knowledge of the sample response at the chosen frequency. Off-line studies can establish such characteristics of the sample. To illustrate a single- and selected-frequency approach, data presented in Figure 3 is analyzed further to establish a dependency between capacitance and coating thickness. Ideally the mapping between measured capacitance, C, and the coating thickness, T would be of the form

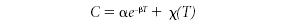
The first term of this equation represents the exponential decrease in the effect of the core layers as the thickness increases. The second term is a function that accounts for the escaping electronic fields. Because these are mostly owing to the zero-order field lines and their dispersion depends on the material property of the coating, this loss also can be written as a function of the thickness, χ(T); α and β are constants. To obtain thickness as a function of capacitance, the previous equation can be rearranged, expanded as a series in T, and the higher order terms neglected. This gives a polynomial fit. Because there are only four data points and at least one degree of freedom is needed to validate the fit, a quadratic expansion is chosen. Thus, the thickness of the coating can be obtained from the measured capacitance using the equation

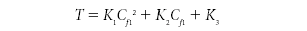
in which T is the thickness of the coating, C_f1 is the capacitance measured at frequency f₁, and K₁, K₂, and K₃, respectively, are the quadratic, linear, and offset coefficients.

Table I: Results of the ranking algorithm showing the top three frequencies ranked by mean error and standard deviation. Error values are normalized.

To determine these coefficients and the frequency to be used, a simple algorithm is performed. First, the measured capacitances are averaged over the four samples for each of the coating levels. For each frequency, the minimum capacitance is subtracted to remove offset errors. Then the values are divided by the maximum capacitance at each frequency to remove the linear errors. The resulting capacitance ratios at each frequency are individually fitted to the quadratic equation above, and the resulting error in estimation is computed. The mean of the estimation errors and the standard deviation is tabulated for each frequency. The frequencies are ranked on the basis of least mean error and the least standard deviation. The product of these rankings is used as a figure of merit for selection of the frequency f₁. Table I shows the results obtained from the algorithm detailed above. It shows that the measurements at 100 kHz are the most suitable for the estimation process. The coefficients K₁, K₂, and K₃ were determined by least square methods. The resulting equation for estimation of coating thickness is

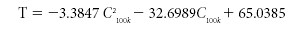
in which C_100k is the mean compensated and normalized capacitance at 100 kHz. Figure 6 shows the comparison between the actual coating thickness and estimated coating thickness. This process of estimation must be further validated by conducting experiments with many more coating levels and samples. Therefore, for inline process measurements, a selected frequency of excitation can be ascertained with some postprocessing of off-line data.

Figure 6 (ALL FIGURES ARE COURTESY OF THE AUTHORS.)

Single sensor or sensor array? Single dielectric spectroscopy sensors can yield a large amount of information about a particular sample. However, in some cases it is important to sense multiple analytes simultaneously. An array can be constructed in which each sensor has a different functional material placed on its surface (see Figure 7). Each functional material is chosen based on known properties of sensitivity or selectivity. Dielectric sensors have been fabricated to sense many volatiles, dissolved chemicals, and solids in a heterogeneous medium. Analytes studied using dielectric sensors include toluene and ethanol (38), n-octanes, 1-proponal, ethylacetate, n-hexanes (39), a wide variety of toxic chemical warfare and industrial agents ranging from nerve agents to rocket fuel and many volatile organic compounds (40), and varying polarity hydrocarbons, aromatics, polar volatile organic compounds (41). This wide variety of chemical sensing capabilities is accomplished by using an equally varied number of polymer bases above the dielectric sensor electrodes. When constructing an array of chemically sensitive dielectric sensors, the electric field excitation frequency also can be tuned to target a dielectric relaxation process that is unique to a specific analyte.

Figure 7 (ALL FIGURES ARE COURTESY OF THE AUTHORS.)

In the process of designing a new sensor that can detect a specific analyte, one method to choose which polymer substrate to use is to determine the chemical solubility of the analyte in the polymer (42). A wide variety of chemicals and solvents can be sensed once the solubility of each analyte is known. Dielectric sensors can be quickly designed by simply studying the solubility of each analyte of interest into each polymer matrix that is used. By using other tuning parameters of dielectric sensors (e.g., the the electric field excitation frequency) and the electrode geometry, dielectric sensors can be designed for both selectivity and sensitivity to many analytes of interest on a single substrate.

Real-time or not? Real-time measurements in a process may be required if the result of those measurements are used to control another step in that process in a time-sensitive manner. Depending on how quickly events occur within the process, measurements may need to be taken very quickly and coarsely or more slowly and rigorously. For example, if moisture must be known before entering a drying cycle, real-time operation implies that the measurement is performed before the material enters the drying cycle. Continuous measurements imply real-time measurements when each measurement must be performed at a sufficient rate so that information about the process is not lost. While dielectric relaxation processes can vary widely from many seconds to less than a nanosecond, faster relaxation processes are typically used to achieve real-time operation in a process.

Conclusion

Dielectric spectroscopy is a promising technology that has found uses in many fields and is now being realized as a useful tool for the pharmaceutical industry. This paper discusses a number of versatile adaptations made possible by advances in electrode materials, fabrication techniques, and measurement systems. Presently, dielectric spectroscopy offers process analysts a wide variety of options, much like an advanced tool, where parameters can be tweaked as desired to implement a "tailor-made" process control solution. For instance, the measurement frequency can be changed to optimize measurements for slow bulk process or for fast intermolecular interactions. Similarly, changing the structural properties of electrodes such as coating of sensor heads can result in improved sensitivity to a particular analyte, or, changing the physical spacing between electrodes can selectively measure specific regions in a sample. Dielectric measurements can be made in a noncontact, nondestructive mode; therefore, measurement instrumentation is relatively easy to integrate at different stages of the process. Measurement time issues not withstanding, a process engineer could chose to obtain data at an early stage of the process and implement a feed-forward control system with the same ease as more conventional feedback-based control. Further, for industrial dielectric measurements, the frequency range is generally below 100 MHz; therefore, instrumentation required for such measurements is relatively inexpensive and does not have signal integrity issues associated with high frequency instrumentation. Dielectric spectroscopy has been widely investigated for measurement of pharmaceutical properties; however, majority of the work has been focused on establishing the feasibility of this technology at a laboratory level. Further work, more inline with the PAT initiative, will focus on identifying specific process needs and customizing sensor designs and circuitry.

Further reading

A significant amount of literature is available that describes the theoretical aspects of dielectric behavior, algorithms, and signal processing methods used for the processsing of dielectric data and sensor design for dielectric measurements. One of the earliest models describing the frequency dependence of dielectric behavior was proposed by Debye (43). Jonscher describes dielectric relaxation emphasizing solids materials (44). Dielectric spectroscopy also has been explored in detail for polymeric materials (36). An understanding of dielectric behavior for engineers is presented by Coelho (45). Specifically for pharmaceuticals, a comprehensive review of applications is provided by Craig (14). MacDonald provides a review of methods for measurement of dielectric properties (46). A number of algorithms have been proposed for calibration and correlating data to physical property distributions (47–49). Rapid advancements in microtechnology have resulted in an increase in the number and complexity of electrode structures available for dielectric measurements. Notable references for sensor design are also available (50–56). A comprehensive overview of interdigital dielectric sensors is provided by Sundara-Rajan (57). A detailed review of currently available dielectric spectroscopic systems can be found at www.ee.washington.edu/research/seal/pharmatech/.

A. Mathur is a graduate student, K. Sundara-Rajan* is a PhD candidate, G. Rowe is a PhD candidate, and A. V. Mamishev is an associate professor at the Sensors, Energy, and Automation Laboratory, Department of Electrical Engineering, University of Washington, Seattle, tel. 206.351.8101, kishore@u.washington.edu.

*To whom all correspondence should be addressed.

Submitted: Oct. 22, 2007. Accepted: Jan. 15, 2008

References

1. V.H. Steve, "Focusing Process Analytical Technology on the Business Objectives of Modern Pharmaceutical Production," J. Proc. Anal. Technol. 2 (4), 15–17 (July 2005).

2. Z.P. Hu et al., "Colon Delivery Efficiencies of Intestinal Pressure-Controlled Colon Delivery Capsules Prepared by a Coating Machine in Human Subjects," J. Pharm. Pharmacol. 52 (10), 1187–1193 (2000).

3. B. J. Lee, S.G. Ryu, and J.H. Cui, "Controlled Release of Dual Drug-Loaded Hydroxypropyl Methylcellulose Matrix Tablet Using Drug-Containing Polymeric Coatings," Int. J. Pharma. 88 (1), 71–80 (1999).

4. B.C. Lippold and R.M. Pages, "Control and Stability of Drug Release From Diffusion Pellets Coated With the Aqueous Quaternary Polymethacrylate Dispersion Eudragit((R)) RS 30 D," Pharmazie 56 (6), 477–483 (2001).

5. A.G. Ozturk et al., "Mechanism of Release From Pellets Coated With An Ethylcellulose-Based Film," J. Controlled Release 14 (3), 203–213 (1990).

6. K.E. Peiponen et al., "Optical Coating Inspection of Pharmaceutical Tablets by Diffractive Element," Measure. Sci. Technol. 8 (7), 815–818 (1997).

7. M.D. Tousey, "The Granulation Process 101: Basic Technologies for Tablet Making," Pharm. Technol. 26 (10), 8–13 (2005).

8. H. Wikstrom, P.J. Marsac, and L.S. Taylor, "In-Line Monitoring of Hydrate Formation During Wet Granulation Using Raman Spectroscopy," J. Pharm. Sci. 94 (1), 209–219 (2005).

9. K.A. Bakeev, "Near-Infrared Spectroscopy As a Process Analytical Tool Part II: At-Line and on-Line Applications and Implementation Strategies," Spectroscopy 19 (1), 39–42 (2004).

10. L. Niklas, A. Osmo, and Y. Juoko, "Does a Powder Surface Contain All Necessary Information for Particle Distribution Size Analysis ?," Eur. J. Pharm. Sci. 17, 217–227 (2002).

11. R.R. Shinde et al., "Frequency-Domain Photon Migration Measurements for Quantitative Assessment of Powder Absorbance: A Novel Sensor of Blend Homogeneity," J. Pharm. Sci. 88 (10), 959–966 (1999).

12. T. Lenarczuk, S. Glab, and R. Koncki, "Application of Prussian Blue-Based Optical Sensor in Pharmaceutical Analysis," J. Pharm. Biomed. Anal. 26 (1), 163–169, Aug. 2001.

13. M.D. Mowery et al., "Rapid at-Line Analysis of Coating Thickness and Uniformity on Tablets Using Laser Induced Breakdown Spectroscopy," J. Pharm. Biomed. Anal. 28 (5), 935–943 (2002).

14. D.Q.M. Craig, Dielectric Analysis of Pharmaceutical Systems (Taylor & Francis Group, UK, 1995).

15. E. Vogel and H. Pauly, "Dynamic Dipole Polarizability of a Dielectric Sphere in an Electrolyte," J. Chem. Phys. 89, 3823–3830 (1988).

16. W.C. Chew and P.N. Sen, "Dielectric Enhancement Due to Electrochemical Double Layer: Thin Double Layer Approximation," J. Chem. Phys. 77, 4683–4693 (1982).

17. K. Sekine and T. Hanai, "Dielectric Analysis of PMMA Microcapsules Containing an Aqueous KCl Solution to Derive a Distribution Function of the Realease Rate of KCl," Colloid Polymer Sci. 269 (9), 880–888 (1991).

18. L.A. Dissado, R.C. Rowe, and A. Haidara, "The Characterization of Heterogeneous Gels by Means of a Dielectric Technique 1: Theory and Preliminary Evaluation," J. Colloid Interface Sci. 117 (2), 310–324 (1987).

19. H. Helene, S. Maria, and G. Kimura, "Drug Molecules As Probes for Studying the Compatibility Between Gels and Mucous Tissue Using Dielectric Spectroscopy," J. Pharm. Sci. 94 (5), 1090–1100 (2005).

20. D. Isaacson, J. Mueller, and S. Siltanen, "Biomedical Applications of Electrical Impedance Tomography," Physiolog. Measure. 24 (2), editorial, 2003.

21. W. Daily, A. Ramirez, and A. Binley, "Remote Monitoring of Leaks in Storage Tanks Using Electrical Resistance Tomography: Application at the Hanford Site," J. Environ. Engineer. Geophys. 9 (1), 11–24 (2004).

22. T. York, "Status of Electrical Tomography in Industrial Applications," J. Electronic Imaging 10 (3), 608–619 (2001).

23. G. von Guggenberg, "Application of Interdigital Dielectrometry to Moisture and Double Layer Measurements in Transformer Insulation," Department of Electrical and Computer Engineering, Massachusetts Institute of Technology, Cambridge, Massachusetts, 1993.

24. J. Melcher, Y. Daben, and G. Arlt, "Dielectric Effects of Moisture in Polyimide," IEEE Transactions on Electrical Insulation 24 (1), 31–38 (1989).

25. D. D. Denton, J. B. Camou, and S. D. Senturia, "Effects of Moisture Uptake on the Dielectric Permittivity of Polyimide Films," in Proceedings of the 1985 International Symposium on Moisture and Humidity (Washington, D.C, 1985), pp. 505–513.

26. Kapton Polyimide Film: Summary of Properties (Du Pont Company, 1998).

27. J. Fabien et al., "AC Impedance Based Chemical Sensors for Organic Solvent Vapours," Sensors and Actuators B, 363–369 ( 1996).

28. R. Igreja and C.J. Dias, "Dielectric Response of Interdigital Chemocapacitors: The Role of Sensitive Layer Thickness," Sensors and Actuators B 115 (1), 69–78 2005.

29. G.Hagen et al., "Selective Impedance Based Gas Sensors for Hydrocarbons Using ZSM-5 Zeolite Films With Chromium(III)Oxide Interface," Sensors and Actuators B 119 (2), 441–448 (2006).

30. K. Sundara-Rajan, L. Byrd, and A. V. Mamishev, "Moisture Content Estimation in Paper Pulp Using Fringing Field Impedance Spectroscopy," IEEE Sensors Journal 4 (3), 378–383, June 2004.

31. K. Sundara-Rajan, L. Byrd, and A. V. Mamishev, "Estimation of Moisture Content in Paper Pulp Containing Calcium Carbonate Using Fringing Field Impedance Spectroscopy," Proceedings of 58th Appita Annual Conference (Canberra, Australia, vol. 2, 2004), pp. 413–420.

32. K. Sundara-Rajan, L. Byrd, and A. V. Mamishev, "Measuring Moisture, Fiber, and Titanium Dioxide in Pulp With Impedance Spectroscopy," TAPPI Journal 4 (2), 23–27 (Feb. 2005).

33. J. K. Moscicki and G. Williams, "Model Calculations of the Phase Behaviour of Rod-Like Particles in Solution: Polydisperse Systems Having Asymmetric Distributions of Rod-Lengths," Polymer 24 (1), 85–90 (1983).

34. J. K. Moscicki and G. Williams, "Concentrated Solutions of Polydisperse Rodlike Polymers in the Isotropic and Lyotropic Liquid-Crystalline Phases. II. Model Simulation of the Dielectric Relaxation Behavior of Poly(n -Alkylisocyanates) in Toluene and Comparison with Experimental Results," J. Polymer Sci. Polymer Phys. 21 (2), 213–231 (Feb. 1983).

35. J. K. Moscicki and G. Williams, "Concentrated Solutions of Polydisperse Rodlike Polymers in the Isotropic and Lyotropic Liquid-Crystalline Phases. I. The Effects of Molecular Length Distribution on Phase Behavior," J. Polymer Sci. Polymer Phys. 21 (2), 197–212 (Feb. 1983).

36. J.P. Runt and J.J. Fitzgerald, Dielectric Spectroscopy of Polymeric Materials: Fundamentals and Applications (American Chemical Society Washington DC, 1997).

37. G. Williams and D. C. Watts, "Non-Symmetrical Dielectric Relaxation Behavior Arising From a Simple Empirical Decay Function," Transactions of Faraday Society 66, 80–85 (1970).

38. A. Hierlemann et al., "Application-Specific Sensor Systems Based on CMOS Chemical Microsensors," Sensors and Actuators B: Chemical, 70 (1–3), 2–11 (Nov. 2000).

39. I. Rui and C.J.Dias, "Dielectric Response of Interdigital Chemocapacitors: The Role of Sensitive Layer Thickness," Sensors and Actuators B 115 (1), 69–78 (2005).

40. T. E. Mlsna, S. Cemalovic, M. Warburton, S. T. Hobson, D. A. Mlsna, and S. V. Patel, "Chemicapacitive Microsensors for Chemical Warfare Agent and Toxic Industrial Chemical Detection," Sensors and Actuators B: Chemical, 116 (1–2), 192–201 (July 2006).

41. S.V. Patel et al., "Chemicapacitive Microsensors for Volatile Organic Compound Detection," Sensors and Actuators B: Chemical 96 (3), 541–553 (Dec. 2003).

42. M. P. Eastman et al., "Application of the Solubility Parameter Concept to the Design of Chemiresistor Arrays," J. Electrochem. Society 146 (10), 3907–3913 (1999).

43. P. Debye, Polar Molecules, Chemical Catalog Co., 1929.

44. A.K. Jonscher, Dielectric Relaxation in Solids (Chelsea Dielectrics Press London, 1983).

45. R. Coelho, Physics of Dielectrics for the Engineer (Elsevier Scientific Publishing Company Amsterdam, 1979).

46. J.R. MacDonald, Impedance Spectroscopy : Emphasizing Solid Materials and Systems (Wiley New York, 1987).

47. B.A. Berdnikov and A.V. Mamishev, Investigation of Properties, Optimization, and Development of Parameter Estimation Algorithms for a Three-Wavelength Interdigital Sensor. Final UROP Report ( High Voltage Research Laboratory Massachusetts Institute of Technology, Feb. 1997).

48. A.V. Mamishev et al., "Development and Applications of Multi-Wavelength Interdigital Dielectrometry Sensors and Parameter Estimation Algorithms," Joint Symposium on Electrostatics (Stanford University, CA, 1998), pp. 169–181.

49. A.V. Mamishev et al., "Development and Applications of Fringing Electric Field Sensors and Parameter Estimation Algorithms," J. Electrostatics 46 (2), 109–123 (1999).

50. M.C. Zaretsky and J.R. Melcher, "Complex Permittivity Measurements of Thin Films Using Microdielectrometry," Conference on Electrical Insulation and Dielectric Phenomena (Claymont, DE, 1986), pp. 462–471.

51. M.C. Zaretsky, J.R. Melcher, and C.M. Cooke, "Moisture Sensing In Transformer Oil Using Thin-Film Microdielectrometry," IEEE Transactions on Electrical Insulation 24 (6), 1167–1176 (Dec. 1989).

52. M.C. Zaretsky, P. Li, and J. R. Melcher, "Estimation Of Thickness, Complex Bulk Permittivity And Surface Conductivity Using Interdigital Dielectrometry," IEEE Transactions on Electrical Insulation 24 (6), pp. 1159–1166 (Dec. 1989).

53. P.A. von Guggenberg and J. R. Melcher, "A Three-Wavelength Flexible Sensor for Monitoring the Moisture Content of Transformer Pressboard," Proceedings of The 3rd International Conference on Properties and Applications of Dielectric Materials, vol. 2 (Tokyo, Japan, 1991), pp. 1262–1265.

54. S.D. Senturia, "The Future of Microsensor and Microactuator Design," Sensors and Actuators A: Physical 56, 151–166 (Mar. 1996).

55. S. D. Senturia and S. L. Garverick, "Method and Apparatus for Microdielectrometry," US Patent No.4,423,371, Dec. 1983.

56. X. Li, A.S. Zyuzin, and A.V. Mamishev, "Measuring Moisture Content in Cookies Using Dielectric Spectroscopy," in Electrical Insulation and Dielectric Phenomena, 2003 Annual Report, pp. 459–462.

57. K. Sundara-Rajan, A.V. Mamishev, and M. Zahn, "Fringing Electric and Magnetic Field Sensors," Encyclopedia of Sensors (American Scientific Publishers, 2005).