A Design of Experiments for Tablet Compression

The tablet is perhaps the most common pharmaceutical dosage form, and the one with which patients are most familiar. Tablets have been a preferred dosage form for many decades, yet it is still challenging for formulation scientists to develop a robust tablet while minimizing the amount of waste created during the development process.

The current study was intended to determine whether tablet hardness and weight could be controlled during the compression process with proper adjustment to certain machine parameters. In this study, a Pharmatech MB015 intermediate bulk container (IBC) blender was used to prepare a blend consisting of microcrystalline cellulose (MCC, Avicel PH102, FMC BioPolymer) as the bulk ingredient and magnesium stearate (Ligamed) as a lubricant to improve flow behavior. The blend ratio of 98% MCC to 2% magnesium stearate was rotated in the asymmetrical IBC bin for 10 min at a speed of 20 rpm.

Following the blending process, the powder mixture was dispensed into a hopper above a Fette (Rockaway, NJ) 102i tablet press. The 102i is a 24-station research-scale rotary tablet press capable of producing 172,800 tablets/h at its maximum rotational speed. For this set of experiments, 12 of the tablet press's stations were set up, and the selected tablet tooling produced a standard convex round tablet with a 7.5-mm diameter and a cup depth of 0.79 mm. Pretrial testing was performed to establish upper and lower limits for the variables that were determined crucial to producing a robust tablet. All testing was performed during the course of three days in a controlled environment of 70 °F and 45% relative humidity.

Five parameters were selected as main variables for the tableting process. The first was turret speed, for which a limit of 20,000–70,000 tablets/h was set. The turret speed, normally converted from tablets/h to rpm, is simply the rotational speed of the circular table in which the tablet die sits. Increases in speed increase the production rate.

The second variable was feed-frame paddle speed, for which limits of 10–70 rpm were set. Tablet presses use either a gravity-fed feeder or a paddle feeder. The press used in this study incorporated a paddle feeder, which rotates several paddle wheels inside a housing where the powder blend is dispensed. The paddles sweep over the tablet dies and fill them as the paddles rotate. High speeds typically push more powder into the die cavity than low speeds do.

The third variable was tablet-cylinder height during precompression, for which limits of 2–4 mm were set. This variable represents how low the compression roller is set to force the tablet punches through the precompression zone to tamp down the powder and compact the blend slightly before main compression occurs. Large numbers indicate that less force is applied to the powder. Tablet-cylinder height is often converted into a force measured in kilonewtons. Small values typically result in hard, thin tablets, assuming that the amount of powder fill is constant. The purpose of precompression is to remove entrapped air from the filled tablet-die cavity. This task aids the main compression step in producing a robust tablet.

The fourth variable was tablet-cylinder height during main compression, for which limits of 2–3 mm were set. Like the precompression cylinder height, the main compression height is also set at a distance that directly affects the main compression force applied to form the tablet. Large numbers result in soft tablets. Small values typically result in hard, thin tablets, assuming that the amount of powder fill is constant.

The final variable was fill-cam height. A fixed fill cam of 12 mm was chosen for this study. The weight-adjustment ramp determines how low the lower tablet punch sits inside the die at the moment that the feed frame scrapes away the last of the excess material so that the material in the die cavity is level with the die table. This setting affects how much the final tablet will weigh. Large numbers result in heavy tablets. A large fill cam–weight adjustment height means that more powder will be inserted into the die, which typically results in a heavy tablet. The term "fill-cam height" is useful for the purposes of this study simply because it is a shorter description of the weight-adjustment process step.

A tablet is expected to conform to numerous requirements, such as dissolution rate, content uniformity, friability, and thickness. Only the following two responses were selected for the current experiments:

• Tablet hardness (kP): For this study, a Dr. Schleuniger (Manchester, NH) model 8M hardness tester was selected. The tablet is set into the test zone on its flat surface while an actuated arm squeezes the tablet until it fractures. The amount of kilogram-force needed to fracture the tablet is measured and recorded. Four tablets were tested from each experiment, and the average value was recorded.

• Tablet weight (g): During each experiment, 10 tablets were weighed on a Mettler Toledo XS603-S laboratory balance, and the average value was recorded.

Because each factor listed above had a wide range, the pretrial testing focused on producing a tablet with a testable hardness value. The lower limit for the selected Dr. Schleuniger model 8M is 0.8 kp. A tablet hardness of less than 0.8 kP is too soft to hold its shape and falls apart with gentle handling. The upper limit for tablet hardness was chosen based on the maximum allowable compression force of the selected 7.5-mm round tablet tooling. Once the Fette tablet press achieved the maximum allowable force, the upper precompression and main compression limits were selected at a value just below that of the maximum force.

Assuming excellent powder-blend flow properties, tablet weight is primarily a function of the fill-cam size and weight-adjustment ramp setting. Fill cams are produced by the tablet press manufacturer and come in a range of sizes. The cam size is selected according to the desired tablet thickness or weight range. Fill-cam selection also can depend on the shape and size of the tablet being produced. For the 7.5-mm round tablet used in this study, a fixed 12-mm fill cam was selected. This selection permitted the experiments to test the upper and lower limits of the cam size required to produce a tablet that held its shape when handled. It was determined that the weight adjustment ramp should be set to lower and upper limits of 8 and 10 mm, respectively.

Experimental process and screening-study results

Once pretrial experiments were completed, a broad design envelope was established using the five factors previously defined. A screening study was designed using a 2^5–1 -level fractional factorial approach, which produced 16 experiments, as opposed to a full factorial experimental design, which would require 32 experiments. Although only 16 experiments were performed in the screening study, the fractional factorial design produces the same resolution as the full factorial design. In addition to reducing time and material, this 2^5–1 fractional factorial design tests for interactions between each of the five factors. For the screening study, three centerpoint experiments were added to the design to test for nonlinearity (i.e., curvature in the data) and to help determine whether there was a significant lack of fit to the resulting model equation. Centerpoint experiments set all five factors to their midpoint between the low and high limits.

Table I: Results of the screening study for the design envelop for manufacturing a simple tablet formulation.

The screening study data is shown in various tables. Table I shows the design summary, Table II shows the analysis of variance (ANOVA) for tablet hardness, and Table III shows the ANOVA for tablet weight. All experimental run data was entered into Design-Ease software (Stat-Ease) for analysis.

Table II: Analysis of variance for factorial model according to tablet hardness.

A response ratio of 11.8485 suggests that the model should be transformed. After detailed analysis of the optional transformations, a square-root transformation appeared to present results with the highest confidence.

Table III: Analysis of variance for factorial model according to tablet weight.

The model F value of 509.46 implies that the model was significant. The chance that a model F value this large could occur as a result of noise is less than 0.01%.

Values of p > F less than 0.0500 (at a 95% confidence interval) indicate that model terms were significant. In this case A, C, D, E, CD, and CE are significant model terms (see Table I).

The curvature F value of 109.91 implies that curvature in the design space, as measured by the difference between the average of the center points and the average of the factorial points, was significant. The chance that a curvature F value this large could occur as a result of noise is only 0.01%.

The lack-of-fit F value of 1.74 implies that the lack of fit was not significant relative to pure error, and this result is desirable. The chance that a lack-of-fit F value this large could occur as a result of noise is 41.83%.

The software produces equations that show how the hardness will respond when any of the factors in the equation change. R² measures the confidence that the hardness will be what is expected. Adjusted R² takes into account the variances of the errors. For the first equation, R² is 0.9964, adjusted R² is 0.9945, and adequate precision is 70.834.

The R² of 0.9964 is in reasonable agreement with the adjusted R² of 0.9945. Adequate precision measures the signal-to-noise ratio. A ratio greater than 4 is desirable. The ratio of 70.834 indicates an adequate signal. The following model equation, in terms of coded factors, can be used to establish the design space:

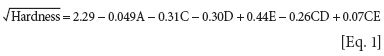
The model F value of 999.17 implies the model was significant. The chance that a model F value this large could occur as a result of noise is less than 0.01%.

Values of prob >F less than 0.0500 indicate that the model terms were significant. In this experiment, A, B, C, and E were significant model terms.

The curvature F value of 1.16 implies the absence of curvature. The chance that a curvature F value this large could occur as a result of noise is 30.09% .

The lack-of-fit F value of 2.17 implies that the lack of fit was not significant relative to pure error, and this result is desirable. The chance that a lack-of-fit F value this large could occur as a result of noise is 35.76%.

For the second equation, R² is 0.9968, adjusted R² is 0.9958, and adequate precision is 70.577. The R² of 0.9968 is in reasonable agreement with the adjusted R² of 0.9958. The adequate precision ratio of 70.577 indicates an adequate signal. The following equation, in terms of coded factors, can be used to establish the design space:

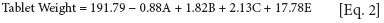
Augmented study approach

The screening-study analysis helped determine a model equation that successfully describes the design space. The hardness and tablet weight models had R² values close to 100%. The models were significant, with at least 95% confidence in the results. The centerpoint runs showed minor curvature in the design space, and an augmented experimental study with additional centerpoints would help determine its effect, since only three centerpoint runs were performed during the screening study. The screening study also showed no evidence that an upper or lower limit had been reached for the hardness or the tablet weight. An augmented study allows for the outer limits of the original screening study to be broadened to expand the factor limits and eventually aid in optimization.

A central composite design (CCD) was selected for the augmented study to enable a response-surface method approach to optimization. Design-Expert software was used to prepare the augmented study factor limits and experimental run sheets. For the augmented study, an additional 15 runs were added to the original screening study of 19 runs, with new upper and lower limits. The 15 augmented runs included five centerpoint runs. For the 10 remaining runs that were not centerpoints, each factor was set at the new upper or lower limit, while the other four factors were set to their midpoints.

The limits for the augmented study are shown in Table IV. Note that the coded values for some of the factors are not at a full ±2 as might be expected. The augmented experimental runs showed that if the limits for some of the factors were extended to two full experimental units, the resulting tablet would not have a measurable response. Modifications to the lower limits had to be made to produce valid data for subsequent analysis. The repercussions of not using the two full experimental units are that the standard error of prediction are no longer symmetrical throughout the design space.

Table IV: Response surface method: augmented central composite design.

Analysis of experimental design

Hardness response. Two responses, tablet hardness and tablet weight, were measured throughout the set of experiments. Each measure response was analyzed individually. Using Design-Expert software, a half-normal plot of the experimental data results was constructed from the original screening results. The half-normal plot for the tablet hardness is shown in Figure 1. Only the significant factors are identified in the half-normal plot. After the sample tablets were pulled from the rotary tablet press, the tablets were left stationary for 2 min before being tested, according to the standard protocol, as previously described. During a formal campaign, the finished batch may be held for at least 24 h before being sampled and tested again. The purpose of the delay is to ensure that the tablets did not rebound because of the compressed material's elasticity. Elastic tablets tend to expand after compression, thus causing a decrease in measured tablet hardness.

Figure 1: Half-normal plot of tablet hardness.

The half-normal plot indicates that factors A, C, D, and E, and interactions CD and CE significantly affected the hardness response. The analysis of variance shown in Table V supports the plot in Figure 1.

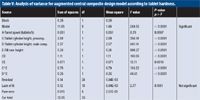
Table V: Analysis of variance for augmented central composite design model according to tablet hardness.

In the next equation, R² is 0.9881, adjusted R² is 0.9842, and adequate precision is 56.625. The R² of 0.9881 is in reasonable agreement with the adjusted R² of 0.9842. The adequate precision ratio of 56.625 indicates an adequate signal, so the following model, in terms of coded factors, can be used to establish the design space:

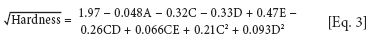
The 95% confidence intervals for all significant factors were verified to exclude zero, which supports the determination that all identified terms in the model equation influence the response. A normal plot of residuals was constructed to determine whether residuals followed a normal distribution (see Figure 2). A plot of residuals versus predicted was constructed to discover convincing trends to the residuals or suspected outliers in the data (see Figure 3). All data points are equally distributed and are within two standard deviations from the mean with two exceptions: one high point is 2.206 standard devations, and one low point is –2.312 standard deviations. The two data points that lie beyond two standard deviations of the mean could be investigated, and these experiments may warrant being rerun to confirm the data response. The data showed no potential outliers.

Figure 2: Normal plot of residuals for hardness.

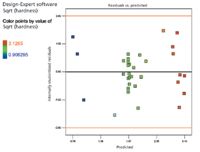
Figure 3: Residuals versus predicted plot for hardness.

Plots were generated for the interactions of CD and CE. These plots are included in the appendix of supporting figures. The 3D-contour CD interaction plot is shaped like a spade because of the data collected during the centerpoint experimental runs. The interaction plot shows that tablet hardness increased as main compression and precompression heights were reduced (see Appendix Figures 1 and 2). This makes logical sense because when the compression rollers are pulled close together, high compression forces are imposed on the tablet, thus resulting in hard tablets. Both 3D plots and standard interaction plots support this analysis.

Appendix Figure 1: Hardness interaction plot for precompression and main compression.

Appendix Figure 2: Hardness 3D interaction plot for precompression and main compression.

The 3D contour CE interaction plot shows a response similar in shape to that of the CD interaction plot, but the shape is more easily interpreted as a channel (i.e., an inverted ridge with a slope that has not yet reached its minimum). The CE interaction plot (see Appendix Figures 3 and 4) indicates an increase in hardness when the fill-cam height (i.e., factor E) is low or high and the precompression roller height is reduced (thus increasing compression force), as would be expected. The tablet weight responded linearly as the fill-cam height (i.e., the weight-adjustment ramp) changed.

Appendix Figure 3: Hardness interaction plot for precompression and fill-cam height.

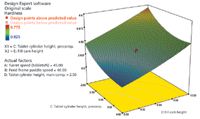
Appendix Figure 4: Hardness 3D interaction plot for precompression and fill-cam height.

Weight response. Tablet weight is a crucial tablet characteristic, and data analysis is necessary for this response. The half-normal plot for tablet weight using the original screening results is shown in Figure 4. The plot indicates that factors A, B, C, and E significantly affected the weight response. The analysis of variance shown in Table VI supports the plot in Figure 4.

Figure 4: Half-normal plot of tablet weight.

In the next equation, R² is 0.9955, adjusted R² is 0.9945, and adequate precision is 126.423. The R² of 0.9955 is in reasonable agreement with the adjusted R² of 0.9945. The adequate precision ratio of 126.423 indicates an adequate signal, so the following equation, in terms of coded factors, can be used to establish the design space:

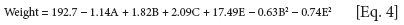
The 95% confidence intervals for the coefficients of each of the significant terms were verified to exclude zero, which supports the determination that all identified factors in the model equation influence the response. A normal plot of residuals was constructed to determine whether the distribution was normal (see Figure 5).

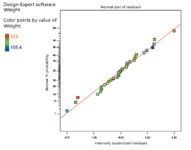
Figure 5: Normal plot of residuals for weight.

A residuals versus predicted plot was constructed to look for trends in the data (see Figure 6). All data points are equally distributed and are within two standard deviations from the mean, with two exceptions. One high point is 2.422 standard deviations, and one low point is –2.473 standard deviations). The two data points that lie beyond 2 standard deviations of the mean could be investigated, and these experiments may warrant being rerun to confirm the data response. The data have no potential outliers.

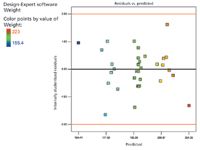
Figure 6: Residuals versus predicted plot for weight.

No two-factor interactions can be plotted, though factor E (i.e., fill-cam height and weight-adjustment ramp) had a significant effect on tablet weight. As can be expected, the amount of powder placed into the die cavity had a direct effect on tablet weight. This is clearly evident in Appendix Figure 5. The plot shows that as the weight ramp or fill cam height is increased, tablet weight increases.

Appendix Figure 5: One-factor plot, tablet weight versus fill-cam height.

Optimization study and discussion of response

Once the initial screening study and the augmented CCD study were concluded, sufficient data had been generated to support an optimization study. The optimization process provides an opportunity to set the two responses, hardness and weight, to desirable outcomes and allow Design-Expert software to produce recommended experiments that should yield tablets with the desired criteria. Optimization is the ultimate goal of any experimental-design process. In this case, a target hardness of 7 kP and a weight of 190 mg were selected. The upper and lower factor limits from the augmented CCD study were used during the optimization process, and eight experiments were performed.

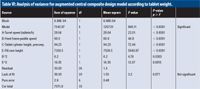
Table VI: Analysis of variance for augmented central composite design model according to tablet weight.

The results of this study were mixed. Two runs were acceptable, but the other six produced undesirable hardness. The results of the optimization experiments are shown in Table VII. For added benefit, output responses from the tablet press were recorded for precompression force and main compression force in kN. Although it would have been preferable to have several more factor setpoint combinations to produce the desired tablet characteristics, several hypotheses can explain why the other experiments did not produce the desired tablet.

Table VII: Optimization study results.

The predicted response 3D plot shows how optimization run 7 responded at the given factor setpoints shown in Table VII. In Figure 7, a small window is evident on the concentric rings in the x axis. The window shows that a limited range of error is permissible to maintain the desired hardness and weight values. The area in red on the 3D contour represents the most desirable response. Further studies could be performed in the small red region with the intent of clearly defining the acceptable factor tolerances when moving a product into a manufacturing environment.

Figure 7: 3D response plot for optimization run 7.

High turret speeds can cause tablet weight to decrease, especially if the feed-frame paddles are turning slowly. This effect occurs when insufficient powder is placed into the tablet-punch die in the brief amount of time that it is under the feed frame. It is also possible that at such high radial velocities, high centrifugal forces push the powder fill out of the die.

When precompression or main compression forces were high, tablet defects were observed in the form of lamination. Lamination likely occurs when the tablet is compressed too quickly, causing the tablet to separate in layers. This defect was often associated with high turret speeds. Lamination may also result from inadequate removal of the entrapped air in the die cavity during the precompression step. A tablet that has begun to laminate may provide false readings on the hardness tester. Lamination was more prevalent when main compression forces were less than precompression forces.

Experienced formulation scientists and production operators understand the complexities of tablet-press operation, and this knowledge can help them to prepare targeted experimental designs that would produce optimization responses with great flexibility in the processing specification setpoints. As the processing specification setpoint error gets larger, the likelihood that the batch will be rejected for undesirable responses decreases. The larger the allowable variation from the processing setpoint, the greater the chance of producing an acceptable tablet.

Conclusion

This study demonstrated that tablet hardness and weight could be controlled during the compression process with proper adjustment to certain machine parameters. Tablet compression is a complex process in solid-dosage manufacturing. An experimental design is a useful tool, especially to the inexperienced user. Experimental design provides great insight into how all of the factors interact and what responses those interactions produce. To an experienced operator, experimental design provides a tool for generating processing-specification setpoints that have large tolerances so that manufacturing batches produce a safe and high-quality product for the patient with minimal rejection and rework. In this study, five factors were selected for all experiments, and the otherwise unknown relationships and interactions were discovered during the experimental process. As a formulator gains knowledge and experience in tablet compression, he or she can use experimental design tools such as Design-Expert with great success when the interactions between the factors are understood.

High turret speeds can result in reduced tablet weight if the feed-frame paddle speed is insufficient. High speeds can also result in tablet lamination if the precompression forces are too high. In production environments, high tablet speed is most desirable because it results in high throughput. Manufacturers must strike a balance between producing robust tablets and maintaining high production rates.

Tablet presses normally use paddle feeders as opposed to gravity feeders. It is necessary to maintain sufficient paddle speed to ensure that the tablet die is properly filled. If the paddle speed is too high, it can overwork the formulation and cause content-uniformity failures. If the paddles pass through the blend too quickly, they can cause the constituents to separate because of their various densities. This effect may be most prominent in ungranulated direct-compression blends. In the most extreme cases, powder overworked through high-speed feed-frame paddles can be subject to shear forces, which can discolor the granulation by overheating the material.

Precompression force is a measure of how much force is imparted to the tablet punch through the precompression zone to tamp down the powder and slightly compact the blend before main compression. This experimental design process showed that light tamping force is preferable to high precompression force. The goal is not to form the tablet at this step, but to remove a significant amount of air in the powder bed to minimize the potential for tablet lamination.

Main compression force is similar to precompression force. Main compression rollers are often larger in diameter than precompression rollers. The larger diameter means that the tablet punch set spends a longer amount of dwell time in the main compression zone forming the tablet. Because high-speed presses have short compression zones, it is typical for a production tablet press to have large diameter compression rollers to extend the dwell time for as long as possible. To incorporate large diameter compression rollers, tablet-press manufacturers must increase the overall turret or die-table diameter to accommodate the larger compression rollers.

The weight-adjustment ramp is an adjustable factor that depends on the fill cam that has been chosen. Fill cams are often chosen according to the size of the tablet being produced. This setting most directly affects how much the final tablet will weigh. For heavy tablets, it is sometimes necessary to increase feeder paddle speed to ensure that the tablet-die cavity is completely filled.

Because tablet lamination was the most common defect, further studies could develop a relationship between establishing limits for precompression force and turret speed to produce tablets that are most likely to be free from this defect.

Matthew N. Bahr is an investigator at GlaxoSmithKline, 1250 S. Collegeville Rd., Collegeville, PA 19426, tel. 610.917.7648, matthew.n.bahr@gsk.com.

Submitted: Mar. 14, 2011. Accepted: May 16, 2011.

Citation: When referring to this article, please cite it as "M.N. Bahr, 'A Design of Experiments for Tablet Compression,' Pharmaceutical Technology Europe 23 (9) 72-81 (2011)." This article was first published in Pharmaceutical Technology Europe's September 2011 print edition and will be published in Pharmaceutical Technology North America's October 2011 print edition.