Mean Kinetic Relative Humidity: A New Concept for Assessing the Impact of Variable Relative Humidity on Pharmaceuticals

It is well established that the rate of chemical degradation of solid-state pharmaceutical products, such as tablets and capsules, is highly dependent upon the environmental temperature and humidity. Indeed, this concept is the underpinning theoretical basis for the pharmaceutical industry guidelines that provide recommendations for long-term, intermediate, and accelerated storage conditions and for establishing expiration dates (1).

The concept of mean kinetic temperature (MKT) was first introduced in 1971 (2) and was further developed in the 1990s (3). MKT can be defined as the single calculated temperature at which the total amount of degradation over a particular period is equal to the sum of the individual degradations that would occur at various temperatures (4). Thus, MKT may be considered as an isothermal storage temperature that simulates the effects of variable storage temperature. The MKT has been found to be useful in a number of pharmaceutically relevant applications, such as providing a scientific means of summarizing variable climatic temperatures (5), handling storage and distribution of pharmaceuticals (6), and for handling temperature excursions (7). The MKT is distinct from the simple arithmetic mean temperature and is generally accepted as a more scientific basis for summarizing variable temperature than the arithmetic mean temperature.

For assessing the impact of variable humidity conditions, on the other hand, no analogous concept to MKT has yet been proposed, because there has been no established means of relating the rate of degradation with humidity. The simple arithmetic mean, therefore, is most commonly used currently for most pharmaceutical applications. As far back as 1977, however, a mathematical relationship between the relative humidity (RH) and the reaction rate in the solid state has been suggested (8), and work by Waterman et al. has since demonstrated that this relationship is widely applicable to pharmaceutical products (9–12). This relationship allows a mean kinetic relative humidity (MKRH) to be calculated analogously to MKT. This article proposes a method for calculating MKRH, and the author discusses the advantages of using MKRH over the simple arithmetic-mean relative humidity and the limitations of the MKRH approach.

Methods

Because the MKRH is analogous in concept to the MKT, it is helpful to provide a brief review of the MKT. The definition of MKT provided above describes how the MKT concept is an attempt to provide a single temperature which, if the product were stored at this constant temperature, would age the product to an equal degree to that obtained from storing the product at a variable temperature over the same period of time. The MKT is derived directly from the Arrhenius equation that relates reaction rate with temperature (Equation 1):

where k = the rate constant of the degradation reaction, A = the 'pre-exponential factor', E_a = activation energy (KJ·mol^-1), R = universal gas constant = 8.314 J·K^-1·mol^-1, and T = temperature (K).

The MKT can be calculated as shown in Equation 2:

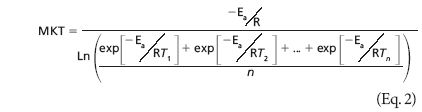
where MKT = mean kinetic temperature (K), T₁ to T_n = the variable temperature (K) measured at constant intervals, and n = the number of temperature measurements.

In the solid state, the rate of the degradation reaction has been shown to vary with temperature and relative humidity according to the humidity-corrected Arrhenius equation in Equation 3 (8–12):

where B = the moisture-sensitivity term and RH = relative humidity (%).

It can be seen from the humidity-corrected Arrhenius equation that reaction rates increase exponentially with RH; the additional B term is the magnitude of the effect of RH on the rate of degradation. The B term varies from product to product (as does E_a) and can be typically determined from a short (e.g., 2 week) accelerated stability study as described by Waterman et al. (9–12). The B term is most simply expressed as the logarithm of the ratio of the degradation rates obtained at two different relative humidity conditions (at the same temperature) divided by the difference in relative humidity as shown in Equation 4:

where k₁ is the rate constant of reaction at relative humidity RH₁, and k₂ is the rate constant of reaction at relative humidity RH₂ at the same temperature.

The B term is typically in the range of 0 to 0.09. A B term of 0 indicates that RH has no effect on reaction rate, whereas a B term of 0.09 indicates a high dependency on RH: the degradation rate would double for every 8% increase in RH.

On the basis of the humidity-corrected Arrhenius equation, the isothermal MKRH can be calculated as shown in Equation 5:

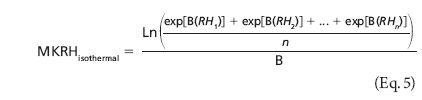
where MKRH_isothermal = mean kinetic relative humidity (%), RH₁ to RH_n = the variable relative humidity (%) measured at constant intervals, and n = the number of relative humidity measurements.

A complication that arises as a consequence of the humidity-corrected Arrhenius equation is that the established MKT equation holds true only for samples held at constant relative humidity, and Equation 5 for MKRH holds true for samples held at constant temperature.

Systems with both variable temperature and variable relative humidity

For systems that experience both temperature and relative-humidity variability, the calculations become more complex, but still can be dealt with by MKT and MKRH approaches. To illustrate one complicating factor, two similar, hypothetical scenarios are considered. In both scenarios, the same hypothetical solid-state pharmaceutical product is exposed to identical temperatures and relative humidity conditions, but in Scenario 1, the high temperature coincides with high relative humidity, and in Scenario 2, the high temperature coincides with low relative humidity, as shown in Table I.

Table I: Hypothetical scenarios for systems with both temperature and relative-humidity variability.

As a consequence of the exponential nature of the humidity-corrected Arrhenius equation, Scenario 1 is more stressful to the product than Scenario 2. For instance, if the hypothetical product had an E_a of 120 KJ·mol-1 and a B term of 0.04 (see later for a discussion on average typical values for E_a and B), Scenario 1 would result in three-fold more degradation than in Scenario 2, and yet both scenarios have identical average temperature and humidity values (i.e., identical MKT, MKRH, arithmetic mean temperature, and arithmetic mean humidity). This illustrates how, in situations in which both temperature and humidity are varying, it can be misleading to calculate the MKT or MKRH in isolation of the other. In order to express situations with both varying temperature and humidity as a single, constant temperature and humidity condition, it is first important to recognize that there is a continuum of constant temperature and humidity conditions that would result in the same amount of degradation as the varying conditions, because any increase in the constant "average" temperature can be compensated by a decrease in the constant "average" relative humidity. Any temperature can be chosen, therefore, within the variable temperature range, and a corresponding MKRH can be calculated using Equation 6:

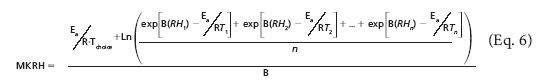
where MKRH = mean kinetic relative humidity (%) calculated for a given temperature, T_choice, T_choice = a chosen constant temperature (K), T₁ to T_n = the variable temperature (K) measured at constant intervals, RH₁ to RH_n = the variable relative humidity (%) measured at constant intervals, and n = the number of temperature and relative humidity measurements.

Alternatively, any relative humidity can be chosen (within the variable relative humidity range), and a corresponding MKT can be calculated using Equation 7:

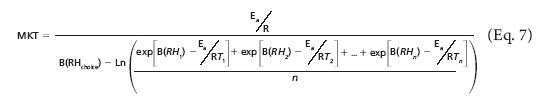
where MKT = mean kinetic temperature (K) calculated for a given relative humidity, RH_choice, and RH_choice = a chosen constant relative humidity (%).

Despite their apparent complexity, these equations are relatively easy to apply using spreadsheet software. One potential problem with this approach is that there is a degree of arbitrariness when choosing T_choice (or RH_choice). If this approach is used, then the use of the MKT calculated without consideration of the variable relative humidity (e.g., according to USP <1150>) should be a good choice for T_choice in most situations, and the associated MKRH can be calculated using Equation 7 (4). Similarly, the use of the MKRH calculated without consideration of the variable temperature should be a good choice for RH_choice, and the associated MKT can be calculated using Equation 7. Figure 1 shows the MKT and MKRH combinations calculated for Scenario 1 and Scenario 2 using this 'combined' approach to temperature and relative humidity variations. As shown in Figure 1, the use of MKT and MKRH calculated in isolation of each other overestimates the degradation for Scenario 2 but underestimates the degradation for Scenario 1, and the use of arithmetic mean temperature and humidity significantly underestimates the degradation for Scenario 1 and even underestimates Scenario 2.

Figure 1: The solid lines represent the temperature-relative humidity combinations that are calculated to result in the same amount of degradation as the variable temperature and relative humidity in Scenarios 1 and 2. These lines were calculated for a product with an activation energy (Ea) of 120 KJ·mol-1 and a B term of 0.04, which represent average values for solid-state pharmaceutical products. (ALL FIGURES ARE COURTESY OF THE AUTHOR)

Discussion

The previous section shows how the MKRH can be calculated for variations in relative humidity, as long as the moisture-sensitivity parameter, B, is known for the product. The use of MKRH has advantages over the use of arithmetic mean relative humidity in that the MKRH provides a better estimate of the constant-storage relative humidity that simulates the effects of multiple, variable-relative humidity conditions for processes that are expected to follow the humidity-modified Arrhenius equation, such as chemical degradation. It also helps avoid underestimates which can mislead product developers.

Using estimated E_a and B values to calculate MKT and MKRH. One inconvenience of the MKRH approach is that a moisture-sensitivity parameter, B, is required for the calculation, in the same way that E_a is required for the calculation of MKT. In the calculation of MKT, it is common practice to use an estimated value for E_a. USP <1150> (4) advocates the use of 83.144 KJ·mol^-1 (19.87 KCal·mol^-1) based partly on the average of the E_a values cited in the literature between 1950 and 1980 (13, 14) and partly due to the convenience that E_a/R equates to almost exactly 10,000 K^-1. The MKT is always higher than the arithmetic mean temperature; the magnitude of the difference increases with higher E_a and with more extreme differences in the variable temperature measurements. Analogously, the (isothermal) MKRH is typically higher than the arithmetic mean relative humidity, and the magnitude of the difference increases with higher B terms and with more extreme differences in the variable relative humidity measurements.

Figure 2 summarizes the E_a and B terms of approximately 40 different chemical degradation processes that occur in solid-state pharmaceutical products (e.g., tablets), as determined by the author. The average E_a for the sample of products is approximately 120 KJ·mol^-1, ranging from 74 to 172 KJ·mol^-1 and the average B term is approximately 0.04, ranging from 0 to 0.09.

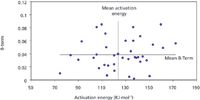
Figure 2: The range of activation energy (Ea) and B terms observed in the laboratory for a sample of 40 degradation reactions that occur in solid oral-pharmaceutical products.

The average E_a determined here is higher than the commonly cited literature value. Although the reason for this is uncertain, it may reflect that the chemical reactions in this experiment occurred in the solid state, and the literature value was, instead, mainly obtained from solution-state reactions. In any case, caution should be exercised if a generic average value for the E_a or B term is used to calculate MKT or MKRH, because a wide range of E_a and B terms appear to be observed in solid-state chemical reactions. In some cases, depending on the magnitude of temperature or relative humidity variability, the choice of E_a or B term used in the calculation may have a negligible effect on the MKT or MKRH. This can be misleading, however, because processes with high E_a terms (or B terms) are sensitive to changes in temperature (or relative humidity), and so even small differences in mean temperature (or relative humidity) can significantly change the amount of degradation. Therefore, we would recommend determining the E_a and B term on a case-by-case basis by means of a short, accelerated stability study as described by Waterman et al. (9–12). Alternatively, the E_a and B term can be estimated by careful analysis of existing long-term, intermediate, and accelerated stability data; a manuscript detailing a method for achieving this is in preparation.

Application of the MKRH approach to packaged pharmaceuticals. Manufacturers are usually concerned with the stability of packaged products and substances, but the relative humidity external to the packaging is not the same as that inside an air-tight container, such as a capped bottle or blister pack. One potentially useful application of MKRH is for providing a convenient, single, constant, relative-humidity value that can be used to summarize and assess the impact of the variable relative humidity that occurs inside pharmaceutical packaging. The relative humidity inside a pharmaceutical pack can be accurately simulated as a function of the external conditions using the methods described by Waterman et al. if the moisture vapor transmission rate (MVTR) is known for the packaging and the moisture vapor sorption isotherm is known for the product or its constituents (15). For example, Figure 3 shows how the relative humidity is expected to vary inside a capped, 40-cc polyethylene terephthalate bottle containing 100 tablets with an initial water content of 2.0%, which, according to the product's moisture vapor sorption isotherm, equates to a water activity of 0.15; the bottle is stored at 30 °C/75% RH for 6 months. The relative humidity inside the bottle starts at approximately 15% RH (i.e., equilibrated to the initial water activity of the product), and, due to the water transmission through the bottle, slowly equilibrates towards the external relative humidity of 75% RH. The MKRH approach enables this variable, dynamically changing internal relative humidity to be expressed conveniently as a scientifically relevant mean value. For comparison, Figure 3 shows the MKRH calculated using B terms of 0.04 and 0.09 and the arithmetic mean relative humidity.

Figure 3: The variable relative humidity inside a capped bottle containing product of initial water content 2.0%, which, according to the product’s moisture vapour sorption isotherm equates to a water activity of 0.15; stored at 30 °C/75% RH for six months. The mean kinetic relative humidity (MKRH) over the six-month period is shown for the product with a B term of 0.04 and 0.09, along with the arithmetic mean relative humidity.

Limitations of the MKRH approach. The MKRH approach has some limitations that need to be considered when assessing the impact of relative humidity on the stability of a pharmaceutical substance or product. The most important consideration is that MKRH covers only chemical degradation. There are a number of other factors that may be affected by humidity but would not be expected to obey the humidity-corrected Arrhenius equation (e.g., dissolution performance, morphology changes of the active or an excipient, depression of glass transition temperatures, hydration or deliquescence of the active or an excipient). The risk of humidity variability on each of these factors needs to be considered separately to the calculation of MKRH; similar limitations also apply to the use of MKT.

Conclusion

An equation for the calculation of MKRH is based upon the humidity-corrected Arrhenius equation, which has been shown to be applicable to a wide variety of solid-state pharmaceutical products and active substances. The calculation of the MKRH requires a moisture sensitivity parameter, B, to be known, analogously to the way E_a is required for the calculation of MKT. In many situations, such as those involving chemical degradation, the use of MKRH is more appropriate than the use of the arithmetic mean relative humidity, in much the same way that MKT is more appropriate than the use of arithmetic mean temperature.

It is relatively simple to calculate the MKRH for constant temperature situations or to calculate the MKT for constant humidity conditions using established methods, such as those described in USP <1150> (4). In situations that have both variable temperatures and humidity conditions, it may be necessary to use a combined temperature and relative humidity calculation as described above, which provides a continuum of constant temperature and constant relative humidity combinations that would age the product to the same degree as the set of variable temperature and variable relative humidity conditions. However, in most situations, this combined calculation is not likely to be necessary, because either the relative humidity can be considered to be effectively constant (e.g., when considering packaged products over short time periods, such as with temperature excursions that occur during the distribution of products), or the temperature can be considered constant (e.g., during stability testing of packaged products in which the relative humidity inside the packaging changes dynamically over time and testing occurs at specific conditions, such as 25 °C/60% RH or 40 °C/75% RH). Despite its limitations and its apparent complexity, the MKRH approach should prove useful in a number of pharmaceutically relevant situations, as has the analogous concept of MKT.

Acknowledgments

The author would like to thank Ken Waterman for his inspirational scientific guidance and Bill Porter for stimulating technical discussions. The author would also like to thank Tim Lukas, Bruno Hancock, and Pierre Barratt for their useful comments and feedback.

Garry Scrivens, PhD, is in Pharmaceutical Sciences at Pfizer Global Research and Development, Ramsgate Road, Sandwich, Kent, UK, CT13 9NJ, tel. + 44 0 1304 649578, garry.scrivens@pfizer.com.

Submitted: Mar. 2, 2012; Accepted Mar. 28, 2012.

References

1. ICH, Q1A(R2), Stability Testing of New Drug Substances and Products, Step 4 version (2003).

2. J. D. Haynes, J. Pharm. Sci., 60 (6), 927–929 (1971).

3. W. Grimm, Drug Dev. Ind. Pharm., 24 (4), 313–325 (1998).

4. USP 34–NF 29 General Chapter <1150>, "Pharmaceutical Stability," pp. 693–694.

5. M. Zahn, "Global Stability Practices," in Handbook of Stability Testing in Pharmaceutical Development, K. Huynh-Ba, Ed. (Springer Science and Business, New York, NY, 2009), pp. 43–91.

6. R. H. Seevers et al., Pharma. Outsourcing, 10 (3), 30–35 (2009).

7. B. Kommanaboyina and C. T. Rhodes, Drug Dev. Ind. Pharm., 25 (12), 1301–1306 (1999).

8. D. Genton and U. W. Kesselring, J. Pharm. Sci., 66 (5), 676–680 (1977).

9. K. C. Waterman and R. C. Adami, Int. J. Pharm., 293 (1–2), 101–125 (2005).

10. K.C. Waterman, et al., Pharm. Research, 24 (4), 780–790 (2007).

11. K.C. Waterman and S. T. Colgan, Regulatory Rapporteur, 5 (7), 9–14 (2008).

12. K. C. Waterman, "Understanding and Predicting Pharmaceutical Product Shelf-Life," in Handbook of Stability Testing in Pharmaceutical Development, K. Huynh-Ba, Ed. (Springer Science and Business, New York, NY, 2009), pp. 115–135.

13. W. Grimm and G. Schepky, Stabilitatsprufung in der Pharmazie, Theorie und Praxis, (Editio Cantor Verlag, Aulendorf, 1980).

14. L. Kennon, J. Pharm. Sci., 53 (8), 815–81 (1964).

15. K. C. Waterman and B. C. MacDonald, J. Pharm. Sci., 99 (11), 4437–4452 (2010).